物理双语教学课件Chapter9Oscillations振动
- 格式:doc
- 大小:351.00 KB
- 文档页数:15


oscillation 用法(一)Oscillation(振荡)的用法介绍什么是振荡?振荡(Oscillation)是指物体在固定时间内在两个或多个状态之间来回变化的过程。
在不同领域中,振荡都有不同的涵义和应用。
物理领域中的振荡1.机械振荡:机械振荡是指由于物体的弹性形变或势能的变化而产生的周期性运动。
例如,钟摆的摆动、弹簧的拉伸和压缩等都是机械振荡的例子。
2.电磁振荡:电磁振荡是指电荷或电磁场的能量在电路中周期性地来回转化的过程。
例如,交流电的频率就是电磁振荡的频率。
3.光学振荡:光学振荡是指光波在介质中传播时的周期性变化。
例如,激光器中的光波就是通过光学振荡来产生和放大的。
数学领域中的振荡1.正弦振荡:正弦振荡是指以正弦函数为基础的周期性变化。
在数学中,正弦函数是最基本的一类周期函数,描述了很多自然界中的变化规律。
2.傅里叶级数:傅里叶级数是把一个周期函数分解为多个正弦函数的和的方法。
利用傅里叶级数理论,我们可以分析和预测振荡的性质和特征。
3.振荡方程:振荡方程是描述振荡系统行为的方程。
例如,单摆的运动可以用简谐振动方程描述,而电路中的振荡行为可以用LCR电路的振荡方程表示。
其他领域中的振荡1.经济领域:在经济学中,振荡可以用于描述市场的周期性波动。
经济振荡往往与经济周期和商业周期有关,对于经济预测和政策制定有一定的指导意义。
2.生物领域:在生物学中,振荡现象广泛存在于生物体内。
例如,生物钟调控着生物体的昼夜节律,心脏的搏动也是一种生物振荡现象。
3.信息领域:在信息科学中,振荡可以用于描述信号的周期性变化。
例如,音频和视频信号中的波形振动就是一种经典的振荡现象。
以上只是振荡在不同领域中的一些常见应用和用法,振荡作为一个重要的概念和现象,还有许多其他领域中的应用和深入研究。
物理领域中的振荡的用法1. 机械振荡•弹簧振荡:当给弹簧施加力或变形后,会产生弹性形变,从而使弹簧发生周期性的振动。
•摆锤振荡:摆锤是一个具有一定质量的物体,在重力作用下能够以固定点为中心进行摆动。



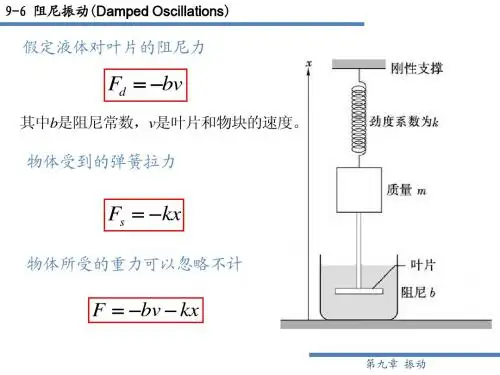


Oscillations & Vibrations Department of PhysicsSoutheast UniversitySeptember 2011◆简谐振动◆阻尼振动◆受迫振动与共振Oscillation is the repetitive variation,typically in time,of some measure about a central value (often a point of equilibrium)or between two or more different states.Familiar examples include a swinging pendulum and AC power.---wikipedia例子:LC回路对水平放置的振子的描述:质量为m 的物体在x 方向无摩擦运动;物体与一弹簧相连,弹簧的左端被固定;x 是物体离开平衡位置的位移,也是弹簧长度的变化量; 物体偏离平衡位置时,它所受的合外力倾向于使它回到平衡位置上来.我们称这个力为恢复力.对水平放置的简谐振子的描述:当恢复力与位移x 成正比(即满足胡克定律)时,水平放置的振子的振动最简单,该振动被称为简谐振动.作简谐振动的振子被称为简谐振子.x F x F kx=-根据牛顿第二定律,简谐振子的运动方程为:22x x d xkx F ma m dt -===220d xkx m dt +=这个简谐振动的角频率被定义为:kmω=水平放置的简谐振子的运动方程于是变成:20x x ω+=20q q ω+=q 可以这个例子中的位移;LC 回路中电容器某极板的带电量或流过自感线圈的电流,等等.电容器两端电势差为自感线圈的自感电动势为导线中的电流为/u q c =d d i Ltε=-d d qi t=-||u ε=1q q LC +=设垂直于纸面向外的方向为z 轴正方向根据角动量定理d d Lt τ=质点相对于挂点的角动量为ˆL I I ωω==z d d t θω=作用在质点上的、相对于挂点的合外力矩为sin ()ˆr F Lmg τθ=⨯=-z 2I mL =整理可得sin 0gL θθ+=sinθθ≈θ当摆角很小时,0gL θθ+≈根据叫动量定理可得:在摆角很小时,0dmg I θθ+≈I =刚体相对于过挂点转轴的转动惯量;d =重心与挂点之间的距离。

Chapter 9 OscillationsWe are surrounded by oscillations─motions that repeat themselves. (1). There are swinging chandeliers, boats bobbing at anchor, and the surging pistons in the engines of cars. (2). There are oscillating guitar strings, drums, bells, diaphragms in telephones and speaker systems, and quartz crystals in wristwatches. (3). Less evident are the oscillations of the air molecules that transmit the sensation of sound, the oscillations of the atoms in a solid that convey the sensation of temperature, and the oscillations of the electrons in the antennas of radio and TV transmitters.Oscillations are not confined to material objects such as violin strings and electrons. Light, radio waves, x-rays, and gamma rays are also oscillatory phenomena. You will study such oscillations in later chapters and will be helped greatly there by analogy with the mechanical oscillations that are about to study here.Oscillations in the real world are usually damped; that is, the motion dies out gradually, transferring mechanical energy to thermal energy by the action of frictional force. Although we cannot totally eliminate such loss of mechanical energy, we can replenish the energy from some source.Simple Harmonic Motion1. The figure shows a sequenceof “snapshots” of a simpleoscillating system, a particlemoving repeatedly back andforth about the origin of the xaxis.2. Frequency: (1). One importantproperty of oscillatory motionis its frequency , or number ofoscillations that arecompleted each second . (2). The symbol for frequency is f, and (3) its SI unit is hertz (abbreviated Hz), where 1 hertz = 1 Hz = 1 oscillation per second = 1 s -1.3. Period: Related to the frequency is the period T of the motion,which is the time for one complete oscillation (or cycle). That is f T 1=.4. Any motion that repeats itself at regular intervals is calledperiod motion or harmonic motion . We are interested here in motion that repeats itself in a particular way. It turns out that for such motion the displacement x of the particle from the origin is given as a function of time by)cos()(φω+=t x t x m , inwhich φωand x m ,, are constant. The motion is called simple harmonic motion (SHM), the term that means that the periodic motion is a sinusoidal of time .5. The quantity m x , a positive constant whose value depends onhow the motion was started, is called the amplitude of the motion; the subscript m stands for maximum displacement of the particle in either direction.6. The time-varying quantity )(φω+t is called the phase of themotion, and the constant φ is called the phase constant (or phase angle ). The value of φ depends on the displacement and velocity of the particle at t=0.7. It remains to interpret the constant ω. The displacement )(t xmust return to its initial value after one period T of the motion. That is, )(t x must equal)(T t x + for all t. To simplify our analysis, we put 0=φ. So we then have)](cos[cos T t x t x m m +=ωω. The cosine function first repeats itself when its argument (the phase) has increased by π2 rad, so that we must haveπωπωω22)(=+=+T or t T t . It means f T ππω22==. The quantity ω is called the angular frequency of the motion; its SI unit is the radian per second.8. The velocity of SHM: (1). Take derivative of thedisplacement with time, we can find an expression for thevelocity of the particle moving with simple harmonic motion. That is, )2/cos()sin()()(πφωφωω++=+-==t v t x dtt dx t v m m . (2). The positive quantity m m x v ω= inabove equation is called thevelocity amplitude .9. The acceleration of SHM:Knowing the velocity for simpleharmonic motion, we can find anexpression for the acceleration ofthe oscillation particle by differentiating once more. Thus we have)cos()2/sin()()(πφωπφωω++=++-==t a t v dtt dv t a m m The positive quantity m m m x v a 2ωω== is called the accelerationamplitude . We can also to get )()(2t x t a ω-=, which is thehallmark of simple harmonic motion: the acceleration is proportional to the displacement but opposite in sign, and the two quantities are related by the square of the angular frequency .9.2 The Force Law For SHM1. Once we know how the acceleration of a particle varies withtime, we can use Newton’s second law to learn what forc emust act on the particle to give it that acceleration. For simple harmonic motion, we have kxω. This result-a=-(2=)xmmaF-=force proportional to the displacement but opposite in sign-is something like Hook’s law for a spring, the spring constant here being 2ωmk=.2.We can in fact take above equation as an alternative definition of simple harmonic motion. It says: Simple harmonic motion is the motion executed by a particle of mass m subject to a force that is proportional to the displacement of the particle but opposite in sign.3.The block-spring system forms a linear simple harmonic oscillator(linearoscillator for short),where linear indicatesthat F is proportional to x rather than to some other power of x. (1). The angular frequencyωof the simple harmonic motion of the block is mω. (2). The period of the linear=k/oscillator is k=.2πmT/9.3Energy in Simple Harmonic Motion1.The potential energy of a linear oscillator depends on howmuch the spring is stretched or compressed, that is, on )(t x.We have )(cos 2121)(222φω+==t kx kx t U m . 2. The kinetic energy of the system depends on haw fast theblock is moving, that is on)(t v . We have )(sin 21)(sin )(21)(sin 2121)(22222222φωφωφωω+=+=+==t kx t x m k m t x m mv t K m m m 3. The mechanical energy is2222221)(sin 21)(cos 21m m m kx t kx t kx K U E =+++=+=φωφω The mechanical energy of a linear oscillator is indeed a constant, independent of time.An Angular simple Harmonic Oscillator1. The figure shows an angular version of a simple harmonicoscillator; the element of springinessor elasticity is associated with thetwisting of a suspension wire ratherthan the extension and compressionof a spring as we previously had. Thedevice is called a torsion pendulum ,with torsion referring to the twisting.2. If we rotate the disk in the figure from its rest position andrelease it, it will oscillate about that position in angular simple harmonic motion. Rotating the disk through an angleθ in either direction introduce a restoring torque given byθκτ-=. Here κ (Greek kappa) is a constant, called thetorsion constant , that depends on the length, diameter, and material of the suspension wire.3. From the parallelism between angular quantities and linearquantities (give a little more explanation), we have κπIT 2=for the period of the angular simple harmonic oscillator, or torsion pendulum.PendulumWe turn now to a class of simple harmonic oscillators in which the springiness is associated with the gravitational force rather than with the elastic properties of a twisted wire or a compressed or stretched spring.1. The Simple Pendulum(1). We consider a simplependulum, which consists ofa particle of mass m (calledthe bob of the pendulum)suspended from an un-stretchable, massless string of length L , as in the figure. The bob is free to swing back and forth in the plane of the page, to the left and right of a vertical linethrough the point at which the upper end of the string is fixed.(2). The forces acting the particle, shown in figure (b), are its weight and the tension in the string. The restoring force is the tangent component of the weight θsin mg , which is always acts opposite the displacement of the particle so as to bring the particle back toward its central location, the equilibrium (0=θ). We write the restoring force as θsin mg F -=, where the minus sign indicates that F acts opposite the displacement.(3). If we assume that the angle is small , the θsin is very nearly equal to θ in radians, and the displacement s of the particle measured along its arc is equal to θL . Thus, we have s Lmg L s mg mg F )(-=-=-≈θ. Thus if a simple pendulum swings through a small angle, it is a linear oscillator like the block-spring oscillator.(4). Now the amplitude of the motion is measure as the angular amplitude m θ, the maximum angle of swing. Theperiod of a simple pendulum is g L L mg m k m T /2)//(2/2πππ===. Thisresult hods only if the angularamplitude m θ is small .2. The Physical Pendulum(1). The figure shows a generalizedphysical pendulum, as we shall call realistic pendulum , with its weight g m acting at the center of mass C.(2). When the pendulum is displaced through an angle θ in either direction from its equilibrium position, a restoring torque appears. This torque acts about an axis through the suspension point O in the figure and has the magnitude ))(sin (h mg θτ-=. The minus sign indicates that the torque is a restoring torque, which always acts to reduce the angle θ to zero.(3). We once more decide to limit our interest to small amplitude , so thatθθ≈sin . Then the torque becomes θτ)(mgh -=.(4). Thus the period of a physical pendulum ismgh I T /2π=, when m θ is small. Here I is the rotational inertia of thependulum.(5). Corresponding to any physical pendulum that oscillates about a given suspension point O with period T is a simple pendulum of length L 0 with the same period T . The point along the physical pendulum at distance L 0 from point O is called the center of oscillation of the physical pendulum for the given suspension point.3. Measuring g: We can use a physical pendulum to measurethe free-fall acceleration g through measuring the period of the pendulum.Simple Harmonic Motion and Uniform circular Motion 1.Simple harmonic motion is the projection of uniform circular motion on a diameter of the circle in which the latter motion occurs.2.The figure (a) gives anexample. It shows areference particle P’moving in uniformcircular motion withangular speed ωin areference circle. Theradiusx of the circle ismthe magnitude of theparticle’s position vector.At any time t, theangular position of theparticle is φω+t.3.The projection of particle P’ onto the x axis is a point P. The projection of the position vector of particle P’ onto the x axisgives the location )(t x of P . Thus we find )cos()(φω+=t x t x m . Thus if reference particle P’ moves in uniform circular motion, its projection particle P moves in simple harmonic motion.4. The figure (b) shows the velocity of the reference particle.The magnitude of the velocity ism x ω, and its projection on the x axis is )sin()(φωω+-=t x t v m . The minus sign appears because the velocity component of P points to the left, in the direction of decreasing x .5. The figure (c) shows the acceleration of the reference particle.The magnitude of the acceleration vector ism x 2ω and itsprojection on the x axis is )cos()(2φωω+-=t x t a m . 6. Thus whether we look at the displacement, the velocity, or theacceleration, the projection of uniform circular motion is indeed simple harmonic motion .Damped Simple Harmonic MotionA pendulum will swing hardly at all under water, because the water exerts adrag force on the pendulum that quicklyeliminates the motion. A pendulumswinging in air does better, but still themotion dies out because the air exerts a drag force on the pendulum, transferring energy from the pendulum’s motion.1. When the motion of an oscillator is reduced by an externalforce, the oscillator and its motion are said to be damped . An idealized example of a damped oscillator is shown in the figure: a block with mass m oscillates on a spring with spring constant k. From the mass, a rod extends to a vane (both assumed massless) that is submerged in a liquid. As the vane moves up and down, the liquid exerts an inhibiting drag force on it and thus on the entire oscillating system. With time, the mechanical energy of the block-spring system decreases, as energy is transferred to thermal energy of the liquid and vane.2. Let us assume that the liquid exerts a damped force d Fthat isproportional in magnitude to the velocity v of the vane and block. Then bv F d -=, where b is a damped constant that depends on the characteristics of both the vane and the liquid and has the SI unit of kilogram per second. The minus sign indicates that d F opposes the motion.3. The total force acting on the block is ∑=-=--=dtdx b kx bv kx F . So we have equation 022=++kx dt dx b dt x d m , whose solution is )'cos()(2/φω+=-t e x t x m bt m , where 'ω, the angular frequency of thedamped oscillator, is given by 224'm b m k -=ω.4. We can regard the displacement of the damped oscillator as acosine function whose amplitude, which ism bt m e x 2/-, gradually decreases with time.5. The mechanical energy of a damped oscillator is not constantbut decreases with time. If the damping is small , we can find )(t E by replacing m x with m bt m e x 2/-, the amplitude of thedamped oscillation. Doing so, we findm bt m e kx t E /221)(-≈, which tells us that the mechanical energy decreases exponentially with time .Forced Oscillations and Resonance1. A person swing passivelyin a swing is an example offree oscillation. If a kindfriend pulls or pushes theswing periodically, as in thefigure, we have forced, ordriven, oscillations . There are now two angular frequencies with which to deal with: (1) the natural angular frequency ω of the system, which is the angular frequency at which it would oscillate if it were suddenly disturbed and then left tooscillate freely, and (2) the angular frequencyd ω of theexternal driving force.2. We can use the right figure torepresent an idealized forcedsimple harmonic oscillator if weallow the structure marked “rigidsupport” to move up and down ata variable angular frequency d ω. A forced oscillator oscillates atthe angular frequencyd ω of driving force, and its displacement is given by )cos()(φω+=t x t x d m , where m xis the amplitude of the oscillations. How large the displacement amplitudem x is depends on a complicated function of d ω and ω.3. The velocity amplitude m v of the oscillations is easier todescribe: it is greatest whenωω=d , a condition calledresonance . Above equationis also approximately thecondition at which thedisplacement amplitude m xof oscillations is greatest.The figure shows how the displacement amplitude of an oscillator depends on the angular frequencyof thed driving force, for three values of the damped coefficient b. 4.All mechanical structures have one or more naturalfrequencies, and if a structure is subjected to a strong external driving force that matches one of these frequencies, the resulting oscillations of structure may rupture it. Thus, for example, aircraft designers must make sure that none of the natural frequencies at which a wing can vibrate matches the angular frequency of the engines at cruising speed.。