Numerical Simulation on Oscillation-Sliding-Uplift Rock Coupled Motion of Caisson Breakwater Und
- 格式:pdf
- 大小:475.84 KB
- 文档页数:12


第24卷第12期 V ol.24 No.12 工 程 力 学 2007年 12 月 Dec. 2007 ENGINEERING MECHANICS153———————————————收稿日期:2006-03-25:修改日期:2006-07-16基金项目:国家自然科学重点基金资助项目(50639030);教育部博士点基金资助项目(20050423002)作者简介:*黄维平(1954),男,浙江人,教授,博士,博导,主要从事海洋工程研究(E-mail: wphuang@); 王爱群(1955),男,山东人,教授,学士,主要从事水利学试验研究(E-mail: ghaq@);李华军(1962),男,山东人,教授,博士,博导,院长,主要从事海洋工程研究(E-mail: huajun@).文章编号:1000-4750(2007)12-0153-05海底管道悬跨段流致振动实验研究及涡激力模型修正*黄维平,王爱群,李华军(中国海洋大学海岸与海洋工程研究所,青岛 266071)摘 要:对输送液体的模型管道进行了涡激振动试验研究,试验结果表明:当理论涡脱频率与管道的固有频率不一致时,作用在振荡管道上的涡激力并非简谐扰力,而是具有一定带宽的窄带随机扰力。
因此,管道的涡激振动响应也是一个随机过程。
当理论涡脱频率与管道的固有频率接近时,管道的涡激振动响应逼近简谐振动。
试验结果也表明:作用在振荡圆柱体上的涡激力频率不仅是流速和圆柱体直径的函数,也是圆柱体固有频率的函数。
关键词:海底管道;涡致振动;试验研究;斯特罗哈频率;涡激升力 中图分类号:TU311.3 文献标识码:AEXPERIMENTAL STUDY ON VIV OF SPAN OF SUBSEA PIPELINEAND IMPROVED MODEL OF LIFT FORCE*HUANG Wei-ping , WANG Ai-qun , LI Hua-jun(Institute of Coastal and Offshore Engineering, Ocean University of China, Qingdao 266071, China)Abstract: Tests for the vortex-induced vibration (VIV) of the models of subsea pipeline with internal flow have been carried out. The results show that if there is a big difference between vortex shedding frequency and natural frequency of cylinder, the lift force acting on oscillating cylinder is a stochastic force with narrow bandwidth and if there is a little difference between them, the response of models is periodic oscillation. It is also revealed that the frequency of vortex shedding on oscillating cylinder will change with not only the velocity of fluid and the diameter of the cylinder, but also natural frequency of the cylinder.Key words: subsea pipeline; VIV; experimental study; Strouhal frequency; lift force浅海石油开发中,由于海底冲刷而导致海底管道出现悬空现象常常困扰油田的安全生产,悬跨段的流致涡激振动将引起管道的疲劳破坏。

第15卷 第4期强激光与粒子束Vol.15,No.4 2003年4月HIGH POWER LASER AND PAR TICL E B EAMS Apr.,2003 文章编号: 100124322(2003)0420397204直线感应加速器束流崩溃不稳定性数值模拟Ξ张开志1, 林郁正2, 王华岑1(1.中国工程物理研究院流体物理研究所,四川绵阳621900; 2.清华大学加速器实验室,北京100084) 摘 要: 在理论分析的基础上,开发了直线感应加速器束流崩溃不稳定性数值模拟程序。
描述了利用该程序开展的研究工作,这些研究揭示了束流崩溃不稳定性的一般规律,分析了相关参数对束流崩溃不稳定性的影响,最后提出了直线感应加速器束流崩溃不稳定性抑制方法。
关键词: 直线感应加速器; 束流崩溃不稳定性; 横向阻抗; 横向尾场; 质心横向位移; 数值模拟 中图分类号: TL50 文献标识码: A 直线感应加速器中的束流崩溃不稳定性(BBU)是束流脉冲与感应加速腔相互作用的结果。
束流脉冲经过加速腔时将在其中激励起横向尾场,而横向尾场又将使束流质心产生横向高频振荡,这两种因素互相耦合,最终导致束流崩溃不稳定性。
由于束流崩溃的过程非常复杂,采用解析分析方法得出的结论比较粗略[1],必须采用数值模拟以及试验才能进行比较全面深入的研究。
1 数值模拟原理 将束流脉冲等分成m个束片,研究它们在n个加速腔中的运动。
束流脉冲经过加速腔时,将受到加速电场,横向尾场和螺线管线圈磁场的作用,因而能量、动量和质心的横向位移都会发生变化。
将加速腔分成漂移段和加速间隙两段,在漂移段只考虑轴向磁场的作用,我们假定在一个加速腔中,螺线管磁场是均匀分布的。
从文献[1]中的理论分析出发,可以导出第i个加速腔漂移段中第j个束片的质心横向位移r d(i,j)和横向动量r′d(i,j)的递推关系r d(i,j)=r(i-1,j)cos(kβl)+r′(i-1,j)/[kβsin(kβl)](1a)r′d(i,j)=-r(i-1,j)kβsin(kβl)+r′(i-1,j)cos(kβl)(1b)式中:kβ=qB/2m0cβγ,q是电荷,B是螺线管线圈磁场;l是加速腔长度。

自激振荡脉冲射流喷嘴的修正空化模型汪朝晖;陈思;邓晓刚;王在良【摘要】根据自激振荡脉冲射流喷嘴中的空化发生机理,一种考虑剪切力(包括雷诺剪切力和粘性剪切力)和由湍动能产生的压力脉动对空化影响的修正空化模型被提出来提高喷嘴内流场的模拟精度.修正空化模型通过用户自定义函数UDF挂入到Fluent中定义空化压力属性.采用该修正空化模型模拟计算所得到的不同入口压力和腔径条件下的喷嘴出口压力峰值与以往的实验结果吻合得很好,验证了该修正空化模型的正确性.进一步对剪切力和由湍动能产生的压力脉动对空化的影响进行了分析,并且研究了不同入口压力条件下这两个因素对空化发生的影响的强弱程度以及空化初生的能力.【期刊名称】《机械设计与制造》【年(卷),期】2019(000)002【总页数】5页(P265-268,272)【关键词】自激振荡脉冲射流喷嘴;剪切力;湍动能;修正空化模型;UDF;空化初生【作者】汪朝晖;陈思;邓晓刚;王在良【作者单位】武汉科技大学机械自动化学院,湖北武汉 430081;武汉科技大学机械自动化学院,湖北武汉 430081;重庆科技学院机械与动力工程学院,重庆 401331;江苏科圣化工机械有限公司,江苏淮安 223002【正文语种】中文【中图分类】TH16;TK263.41 引言自激振荡脉冲射流喷嘴能够依靠其自身的特殊结构和特定的边界条件产生自激振荡脉冲效应,使连续入口射流变成高速的脉动出口射流,在没有外界提供附加能量就能提高其喷射性能,因此被认为是一种十分具有前景的射流装置。
由于这一特性,自激振荡脉冲射流喷嘴广泛应用于矿山、破碎、钻井等领域。
目前,已有大量学者采用实验和基于CFD的模拟手段对该喷嘴进行研究[1-4]。
相比于实验方法,基于CFD的模拟方法具有节省时间、人力、物力等优越性。
此外自激振荡效应产生的原因是腔室内部空化演变的结果[5]。
然而喷嘴腔室内空化的演变过程是一个十分复杂的一个过程,采用实验的方法很难精确地去描述腔室内的空化过程以及捕捉微小的细节。

Virgin Pulse CD随身听无【期刊名称】《电器评介》【年(卷),期】2005(000)005【摘要】英国著名品牌Virgin推出的Virgin Pulse CD随身听带有一个可分离的皮带拉手,把它套在手上时手指刚好触到按键,操作十分方便,而当不使用时,把皮带翻过来刚好护住按键和液晶屏,该随身听外形椭圆,表面没有一个螺丝,设计十分完美,每一个部分都散发着诱人的魅力。
【总页数】2页(P4-5)【作者】无【作者单位】无【正文语种】中文【中图分类】TN912.231【相关文献】1.A Comparative Study of Stability of Extra Virgin Olive Oil, Virgin Coconut Oil and Grape Seed Oil against Domestic Deep Frying [J], Nyam Kar Lin;Chew Kin Ken;;;;;;;;;;;;;;;2.Effect of the duty cycle of pulsed current on nanocomposite layers formed by pulsed electrodeposition [J],M.Aliofkhazraei;Sh.Ahangarani;A.Sabour Rouhaghdam3.Theoretical Studies of the Output Pulse with Variation of the PumpingPulse for RF Excited CO_2 Pulsed Waveguide Laser [J], A Rauf;周为;辛建国4.The Theory and Experimental Study of the Self-Excited Oscillation Pulsed Jet Nozzle (Pipeline Pulsed Flow Generator) [J], Shiqiang Lai;Zhengfang Liao5.Numerical simulation of atmospheric pulsemodulated radio-frequency glow discharge ignition characteristics assisted by a pulsed discharge [J], 潘呈献;施政铭;韩乾翰;郭颖;石建军因版权原因,仅展示原文概要,查看原文内容请购买。

基于CFD和气动声学理论的空腔自激振荡发声机理杨党国;李建强;梁锦敏【摘要】应用CFD技术和气动声学时域理论(FW-H积分方程),探讨了空腔自激振荡发声机理.腔内噪声计算以空腔流动解为基础,采用了气动声学时域理论,对该理论进行了推导说明,并利用圆柱绕流声学特性验证该方法基本可行.研究获得的空腔自激振荡模态分析结果与Rossiter和Heller等的预测结果基本相同,捕捉到了自激振荡的频域特性;分析表明空腔上方形成的剪切层中的脱落涡与腔后壁相撞,产生的一次声波辐射至腔前壁激发新的脱落涡,新的脱落涡与腔后壁再次相撞产生二次声波形成的流动声学反馈回路是导致空腔自激振荡和噪声产生的主要原因,且腔内声压幅值主要出现在一阶和二阶振荡模态,声音能量主要集中在较低频率区域.【期刊名称】《空气动力学学报》【年(卷),期】2010(028)006【总页数】7页(P724-730)【关键词】空腔;气动声学;自激振荡;发声机理;CFD;FW-H方程【作者】杨党国;李建强;梁锦敏【作者单位】中国空气动力研究与发展中心空气动力学国家重点实验室,四川,绵阳,621000;中国空气动力研究与发展中心空气动力学国家重点实验室,四川,绵阳,621000;中国空气动力研究与发展中心空气动力学国家重点实验室,四川,绵阳,621000【正文语种】中文【中图分类】V211.3;O422.80 引言空腔绕流广泛存在于航空航天飞行器中如飞机起落架舱、燃烧室、飞机部件接缝、武器舱等。
高速气流流经空腔,当满足一定的空气动力学条件和几何形状条件时,由于腔口剪切流与腔内流动的相互作用,腔内流动可能出现强烈的自持振荡,腔内外存在复杂的非定常流动。
流场不仅包含涡生成、脱落与破裂,还包含流动分离、膨胀波与激波及声与流动相互作用等。
腔内噪声使空腔结构承受较大的非定常载荷,严重时会危及腔内的设备和电子器件,甚至会引起空腔自身结构疲劳损坏。
国外Rossiter于1964年提出了空腔流声共振反馈模型,并给出预估振荡频率的半经验公式[1],后来Heller提出空腔后缘处的反馈声波速度应为当地声速,对Rossiter公式进行了修正[2]。

分类号:密级:U D C :编号:工学硕士学位论文固体火箭发动机内涡脱落现象的大涡模拟硕士研究生:李鹏飞指导教师:贺征副教授学位级别:工学硕士学科、专业:航空宇航推进理论与工程所在单位:航天与建筑工程学院论文提交日期:2012年12月18日论文答辩日期:学位授予单位:哈尔滨工程大学Classified Index:U.D.C:A Dissertation for the Degree of M. EngLarge eddy simulation of vortex shedding forsolid rocket motorCandidate: Li PengfeiSupervisor: Associate Prof. He ZhengAcademic Degree Applied for: Master of EngineeringSpecialty:Aerospace Propulsion theory and Engineering Date of Submission: Dec. 18, 2012Date of Oral Examination:University: Harbin Engineering University哈尔滨工程大学学位论文原创性声明本人郑重声明:本论文的所有工作,是在导师的指导下,由作者本人独立完成的。
有关观点、方法、数据和文献的引用已在文中指出,并与参考文献相对应。
除文中已注明引用的内容外,本论文不包含任何其他个人或集体已经公开发表的作品成果。
对本文的研究做出重要贡献的个人和集体,均已在文中以明确方式标明。
本人完全意识到本声明的法律结果由本人承担。
作者(签字):日期:年月日哈尔滨工程大学学位论文授权使用声明本人完全了解学校保护知识产权的有关规定,即研究生在校攻读学位期间论文工作的知识产权属于哈尔滨工程大学。
哈尔滨工程大学有权保留并向国家有关部门或机构送交论文的复印件。

化工进展Chemical Industry and Engineering Progress2023 年第 42 卷第 8 期脉动热管计算流体力学模型与研究进展卜治丞,焦波,林海花,孙洪源(山东交通学院船舶与港口工程学院,山东 威海 264200)摘要:脉动热管利用工质的潜热和显热实现高效的热传递,过程中伴随气液塞强烈的往复振荡,流动与传热现象极其复杂。
利用计算流体力学模拟可以获得管内气液界面形态、流型转换及振荡压降等重要信息。
本文对公开发表的相关研究进行了综述,介绍各个模型的主要公式、数值模拟的求解方法、优势和现有的局限性,总结现有模拟研究开展的主要工作和结论。
通过分析发现了目前存在的问题:相变模型中蒸发、冷凝系数的确定仍未有明确的理论依据;二维模型中管径的确定方法还未形成共识;将气-液-固三相流动的颗粒流体简化为均质流体。
基于上述问题,本文提出了利用计算流体力学模拟脉动热管后续的研究方向。
关键词:脉动热管;计算流体力学;气液两相流;相变;传热中图分类号:TK172.4;TQ021 文献标志码:A 文章编号:1000-6613(2023)08-4167-15Review on computational fluid dynamics (CFD) simulation and advancesin pulsating heat pipesBU Zhicheng ,JIAO Bo ,LIN Haihua ,SUN Hongyuan(Naval Architecture and Port Engineering College, Shandong Jiaotong University, Weihai 264200, Shandong, China)Abstract: The pulsating heat pipe (PHP) realizes efficient heat transfer through latent and sensible heat of the working fluid. Due to the strong reciprocating oscillation of the gas and liquid plug, the flow and heat transfer mechanisms are extremely complex. Computational fluid dynamics (CFD) simulation on PHPs can provide important information, such as gas-liquid interface shape, flow pattern transition, oscillating pressure drops, etc . Thus, the published CFD simulations on PHPs are reviewed in this paper. The main formulas, numerical simulation methods, advantages and limitations are introduced, and the available simulation research and conclusions are summarized. The analysis reveals some issues to be solved: there is no definite theoretical basis for the choosing of evaporation and condensation coefficients in phase change model; an agreement on the determination of pipe diameter in two-dimensional model has not been reached; the particle fluid of gas-liquid-solid three-phase flow is simplified into the homogeneous fluid. Based on the above problems, further research directions for using CFD to simulate PHPs are proposed.Keywords: pulstating heat pipe; computational fluid dynamics; gas-liquid flow; phase change; heat transfer综述与专论DOI :10.16085/j.issn.1000-6613.2022-1771收稿日期:2022-09-22;修改稿日期:2023-01-15。

收稿日期:2009-05-20基金项目:国家自然科学基金重点资助项目(50539080);国家重点基础发展规划(973计划)资助项目(2007CB209407);国家自然科学基金设备专项资助项目(50727904)作者简介:冯现大(1985-),男,山东潍坊人,硕士研究生,主要从事隧道、煤矿等突水方面的研究.E -mai l:feng.xianda@gmai 文章编号:1672-3961(2009)04-0021-04海底隧道涌水量影响因素的数值模拟研究冯现大,李树忱,徐帮树(山东大学岩土与结构工程研究中心,山东济南250061)摘要:针对某海底隧道,从数值模拟分析的角度,研究涌水量计算的影响因素.建立大量的海底隧道计算模型,采用不同模型尺寸和边界条件,计算其涌水量从而寻找规律.结果表明:边界范围至少为隧道尺寸的7倍时,才能保证涌水量计算结果的准确性;固定孔隙水压力边界引起涌水量被高估,自由孔隙水压力边界与此相反;侧压力系数对海底隧道涌水量的影响基本可以忽略.关键词:涌水量;海底隧道;数值模拟中图分类号:TU4511 文献标志码:ANumerical simulation study on influence factors ofthe seepage volume of submarine tunnelsFE NG Xian -da,LI Shu -chen,XU Bang -shu(Geotechnical and Structural Engineering Research Cen ter,Shandong University,Jinan 250061,China)Abstract :The influence factors of the seepage volume in submarine tunnels were studied by means of nu merical si mulation.A large number of models with di fferent model scales and boundary conditions were built for the numerical si mulation.The computed results showed that when the distance from the boundary to the tunnel profile was at least 7times the tunnel diameter,an accurate seepage volume could obtained,and the fixed boundary makes the seepage volu me be overestimated,while the free pore pressure boundary was underes timated.Key w ords :seepage volu me;submarine tunnel;numerical si mulation0 引言自1936年第一条海底隧道)))日本关门隧道始建以来,世界各地已有近百条海底隧道相继建造.迄今有海底隧道的国家主要包括日本、英国、法国、美国、挪威、澳大利亚、丹麦、冰岛、中国等[1-4].在特殊的环境地质条件下,建造海底隧道无疑是最安全经济、路线最短的方案,但海底隧道的设计与施工还有很多技术问题亟待解决[5].其中,涌水量的预测计算就是海底隧道防排水设计和施工中一个亟待解决的实际问题,迄今为止尚无成熟的理论和公认的准确计算方法.陆上隧道的涌水量预测研究相关文献较多,而海底隧道涌水量研究较少[6-7].隧道涌水量的预测常用解析法、经验类比法和数值模拟法,解析方法和经验类比法仅可以粗略估算涌水量,因为隧道涌水的复杂性和多变性,实际应用中应以数值模拟结果为准[8],所以数值模拟方法就显得尤为重要.为获得较为准确的涌水量计算值,本文从数值模拟分析的角度,研究海底隧道涌水量的影响因素,分别采用不同模型尺寸、不同边界条件和不同的侧压力系数进行第39卷 第4期Vol.39 No.4山 东 大 学 学 报 (工 学 版)JOUR NA L OF SHAN DO NG UNIVERSITY (EN GIN EERING SCIENCE)2009年8月Aug.2009了大量的数值模拟计算,得出了各个因素对海底隧道涌水量的影响规律.1 FLAC 3D 流固耦合基本原理FLAC3D 模拟多孔介质(如土体)中流体流动时,流体的模拟独立于结构计算.其主要通过孔隙水压力的消散引起岩体中位移的变化.流体在孔隙介质中的流动依据Darcy 定律,流固耦合过程满足Biot 方程[9].(1)运动方程流体的运动用Darcy 定律来描述.对于均质、各向同性固体和流体密度是常数的情况,这个方程具有如下形式:q i =-k [p -Q f x j g j ],i ,(1)式中,q i 是渗透流量,p 是孔隙压力,k 是介质的渗透系数(或机动系数),Q f 是流体密度,g j (j =1,2,3)是重力的三个分量.(2)平衡方程平衡方程的形式为R ij ,j +Q g i =Qd v id t,(2)式中,Q =(1-n )Q s +ns Q w 是体积密度,Q s 和Q w 是固体和流体的密度.(3)本构方程由于流体的流动导致孔隙介质中孔隙压力(p ),饱和度(s ),体积应变(e )和温度(T )的改变.则孔隙流体方程为1M 5p 5t +n s 5s 5t =1s 5F 5t -A 5e 5t +B 5T5t,(3)式中,M 是Biot 模量[N/m 2],n 是孔隙率,A 是Biot 系数,B 是热传导系数[1/e ],用此来考虑流体和颗粒的热膨胀.(4)相容方程应变率和速度梯度之间的关系为N ij =12[u i ,j+v i ,j],(4)式中,v i 是介质中某点的速度.2 工程概况某海底隧道拟采用钻爆法施工,设计为双线四车道.单隧道净轮廓如图1所示.隧址上部以粘性土为主,夹少量砾砂,下部为粘土质碎石.基岩为流纹质熔结凝灰岩,灰紫色、紫灰色,凝灰结构,块状构造,局部具流纹状构造.弱风化层岩芯呈碎石状,少数短块状,裂隙发育,锤击易碎.微风化层岩芯较完整,呈柱状、少数碎块状,弱风化流纹质熔结凝灰岩和微风化流纹质熔结凝灰岩承载力较高;层厚较大,揭露厚度大于8105m;分布稳定,工程地质条件较好.图1 隧道内轮廓Fig.1 The profile of the tunnel隧址处地下水主要以松散岩类孔隙承压水、基岩裂隙水为主.松散岩类孔隙承压水分布范围小,且不连续.未发现断层破碎带的存在,基岩较完整,节理不发育,透水性弱.该处有松散岩类孔隙承压水赋存条件.弱风化层基岩顶面作为透水边界,假定受到海平面静水压力的作用,孔隙水初给充足.3 数值计算方案311 计算模型选取某个典型的断面简化为平面分析.计算时取水平面内垂直隧道轴线方向为x 轴,铅直方向为y 轴.x 方向最大范围为?160m,y 方向下边界最大范围为隧道底板线向下100m,上表面为弱风化层顶面.弹塑性有限差网格如图2,运用FLAC 3D 程序计算涌水量.计算中,考虑软土层渗透性较大水头位置取海平面到软土层底面高度.根据渗流时间的不同进行7次计算,渗流时间依次为1h 、2h 、4h 、8h 、16h 、24h 、48h.图2 初始数值模型图Fig.2 The ini tial numerical model22山 东 大 学 学 报 (工 学 版)第39卷312 物理力学参数根据地质报告、岩石室内力学实验、岩石力学参数手册和相关工程经验,本次主要岩层物理力学参数选取如表1.表1 主要岩层物理力学参数及渗透系数Table 1 Mechanical parameters and permeable coefficientof rock mass地层名称密度Q /(g #cm -3)弹模E /GPa 泊松比L 凝聚力c /KPa 摩擦角</(b )渗透系数k /(c m #s -1)¹2156416201261127305@10-7º215991230122215532185@10-8注:¹表示弱风化流纹质熔结凝灰岩;º表示为微风化流纹质熔结凝灰岩.313 计算方案本文模拟计算边界条件、模型尺寸以及侧压力系数对涌水量的影响.在所有模型中,单元大小相同,以便消除离散化本身的影响.(1)模型尺寸对涌水量的影响为了描述方便,本文用L 表示左右边界距隧道洞壁的距离,用H 表示下边界距隧道底板的距离,如图3所示.采用两种方案研究模型尺寸对涌水量的影响:¹固定H 为100m,当L 分别等于20m 、30m 、40m 、50m 、60m 、70m 、80m 、90m 、100m 、110m 、120m 、130m 、140m 时,共建立了13个模型计算海底隧道涌水量;º固定L 为100m,当H 分别等于20m 、30m 、40m 、50m 、60m 、70m 、80m 、90m 、100m 时,共建立了9个模型计算海底隧道涌水量.图3 模型尺寸示意图Fi g.3 The size of numerical model(2)边界条件对涌水量的影响取L =160m,H =100m,分别计算完全透水和完全不透水两种边界条件下海底隧道的渗流量.(3)侧压力系数对涌水量的影响取L =160m,H =100m,分别计算侧压力系数为016和019时海底隧道的渗流量.4 计算结果411 隧道模型尺寸对涌水量的影响(1)边界完全透水时,图4和图5分别为涌水量随L 和H 的变化曲线.当模型边界完全透水时,随着L (H )的增大,涌水量逐渐减小.用D 表示隧道洞径,文中D U 10m.当L (H )<3D 时,涌水量明显偏大,当L (H )>5D 后,涌水量的变化趋于平稳,当L (H )U 7D 时涌水量稳定不再减小.图4 边界完全透水时L 对渗流量的影响曲线Fig.4 The effect curve of L on the seepage volume with fully per -vi ous boundaries图5 边界完全透水时H 对渗流量的影响曲线Fig.5 The effect curve of L on the seepage volume with fully per -vi ous boundaries(2)边界完全不透水时,图6和图7分别为涌水量随L 和H 的变化曲线.由图6和图7可见,当模型边界完全不透水时,随着L (H )的增大,涌水量逐渐增大.当L (H )<3D 时,涌水量明显偏小,当L (H )>5D 后,涌水量的变化趋于平稳,当L (H )U 7D 时涌水量稳定不再增大.图6 边界完全不透水时L 对渗流量的影响曲线Fig.6 The effect curve of L on the seepage volume with completelyimperviable boundaries412 边界条件对涌水量的影响取L =160m,H =100m,分别计算模型边界完全透水和完全不透水两种边界条件下海底隧道的涌水量,如表2所示.第4期冯现大,等:海底隧道涌水量影响因素的数值模拟研究23图7边界完全不透水时H对渗流量的影响曲线Fig.7The effect curve of H on the seepage volume withcompletely imperviable boundaries表2不同边界条件下海底隧道涌水量Table2The seepage volu me under different boundary conditions(m3#d-1)边界条件Q1h Q2h Q4h Q8h Q16h Q24h 完全透水210801182611675115831152911512完全不透水211011181711608114551132411232注:Q n h(n=1,2,3,)表示渗流时间为n h时隧道涌水量.从表2可见,若模型尺寸相同,两种边界条件下海底隧道涌水量均随着渗流时间逐渐减小.模型边界完全透水时,其渗流量要大于边界完全不透水时的渗流量.413侧压力系数对涌水量的影响取L=160m,H=100m,分别计算侧压力系数为016和019时海底隧道的涌水量,结果表明侧压力系数对海底隧道的涌水量影响很小,可以忽略.5结语通过数值模拟分析,本文研究了不同尺寸、不同边界条件、不同侧压力系数对海底隧道涌水量的影响规律,可以得出以下结论:(1)完全透水边界引起渗流量被高估,完全不透水边界与此相反;(2)两种类型的边界条件包含了问题的解,所以可以进行两次模拟,通过取平均值得到问题真解的合理估计.(3)当L/D>7且H/D>7时,才能保证涌水量计算结果的准确性.(4)侧压力系数对海底隧道的涌水量影响很小.参考文献:[1]ODGARD A,B RIDGE D G,ROS TAM S.Design of the store-belt railway tunnel[J].Tunnelling and Undergroud Space Technology,1994,19(3):293-307.[2]EISE NSTEIN Z rge undersea tunnels and the progress oftunneling technology[J].Tunnelling and Undergroud Space Technology,1994,9(3):283-292.[3]KITAMURA A.Tachnical development for the Sei kan tunnel[J].Tunnelling and Undergroud Space T echnology,1986,1 (3/4):341-349.[4]杨家岭,邱祥波,陈卫忠,等.海峡海底隧道及其最小岩石覆盖厚度问题[J].岩石力学与工程学报,2003,22 (增1):2132-2137.YANG Jialing,QIU Xiangbo,C HE N Weizhong,et al.Subsea tunnel through channel and its mini mu m rock cover[J].Ch-i nese Journal of Rock Mechanics and Engineering,2003,22 (S1):2132-2137.[5]曾超,邱祥波,李术才,等.海底隧道的数值模拟研究[J].岩石力学与工程学报,2003,22(增1):2264-2267.ZE NG Chao,QIU Xiangbo,LI Shucai,et al.Numerical simu-lation on subsea tunnel[J].Chinese Journal of Rock Mechan-ics and Engineering,2003,22(S1):2264-2267.[6]邓英尔,谢和平.低渗透微尺度孔隙气体渗流规律[J].力学与实践,2005,27(2):33-35.DENG Ying.er,XIE Heping.Gas flows in micro-scale pore of low permeability porous media[J].Mechanics in Engineering, 2005,27(2):33-35.[7]王建秀,朱合华,叶为民.隧道涌水量的预测及工程应用[J].岩石力学与工程学报,2004,24(7):1150-1153.WANG Jian x iu,ZHU Hehua,YE Weimin.Forward and inve-rse analyses of water flow into tunnels[J].Chi nese Journal of Rock Mechanics and Engineering,2004,24(7):1150-1153.[8]徐帮树,李树忱,李术才,等.海底隧道涌水量与覆岩厚度关系研究[J].力学与实践,2007,29(1):34-37.XU Bangshu,LI Shuchen,LI Shucai,et al.The relation be-tween seepage volume and rock cover thickness in subsea tunnel [J].Mechanics in Engineering,2007,29(1):34-37.[9]李术才,徐帮树,李树忱,等.舟山海底隧道最小岩石覆盖厚度研究报告[R].济南:山东大学,2007.LI Shucai,XU Bangshu,LI Shuchen,et al.The study report for the minimum rock cover of Zhoushan subsea tunnel[R].J-i nan:Shandong Universi ty,2007.(编辑:孙培芹)24山东大学学报(工学版)第39卷。


大气科学系微机应用基础Primer of microcomputer applicationFORTRAN77程序设计FORTRAN77 Program Design大气科学概论An Introduction to Atmospheric Science大气探测学基础Atmospheric Sounding流体力学Fluid Dynamics天气学Synoptic Meteorology天气分析预报实验Forecast and Synoptic analysis生产实习Daily weather forecasting现代气候学基础An introduction to modern climatology卫星气象学Satellite meteorologyC语言程序设计 C Programming大气探测实验Experiment on Atmospheric Detective Technique云雾物理学Physics of Clouds and fogs动力气象学Dynamic Meteorology计算方法Calculation Method诊断分析Diagnostic Analysis中尺度气象学Meso-Microscale Synoptic Meteorology边界层气象学Boundary Layer Meteorology雷达气象学Radar Meteorology数值天气预报Numerical Weather Prediction气象统计预报Meteorological Statical Prediction大气科学中的数学方法Mathematical Methods in Atmospheric Sciences专题讲座Seminar专业英语English for Meteorological Field of Study计算机图形基础Basic of computer graphics气象业务自动化Automatic Weather Service空气污染预测与防治Prediction and Control for Air Pollution现代大气探测Advanced Atmospheric Sounding数字电子技术基础Basic of Digital Electronic Techniqul大气遥感Remote Sensing of Atmosphere模拟电子技术基础Analog Electron Technical Base大气化学Atmospheric Chemistry航空气象学Areameteorology计算机程序设计Computer Program Design数值预报模式与数值模拟Numerical Model and Numerical Simulation接口技术在大气科学中的应用Technology of Interface in Atmosphere Sciences Application海洋气象学Oceanic Meteorology现代实时天气预报技术(MICAPS系统)Advanced Short-range Weather Forecasting Technique(MICAPS system)1) atmospheric precipitation大气降水2) atmosphere science大气科学3) atmosphere大气1.The monitoring and study of atmosphere characteristics in near space as an environment for space weapon equipments and system have been regarded more important for battle support.随着临近空间飞行器的不断发展和运用,作为武器装备和系统环境的临近空间大气特性成为作战保障的重要条件。

第9卷㊀第1期2024年1月气体物理PHYSICSOFGASESVol.9㊀No.1Jan.2024㊀㊀DOI:10.19527/j.cnki.2096 ̄1642.1081电磁助推翼段加速地面效应及稳定性分析罗星东ꎬ㊀侯自豪ꎬ㊀李少伟ꎬ㊀薄靖龙ꎬ㊀翟茂春(中国航天科工飞航技术研究院磁电总体部ꎬ北京100074)GroundEffectsandStabilityAnalysisofAirfoilAcceleratedbyElectromagneticPropulsionLUOXingdongꎬ㊀HOUZihaoꎬ㊀LIShaoweiꎬ㊀BOJinglongꎬ㊀ZHAIMaochun(InstituteofMagneticLevitationandElectromagneticPropulsionꎬHIWINGTechnologyAcademyofCASICꎬBeijing100074ꎬChina)摘㊀要:电磁发射空天飞行器是未来可重复使用天地往返运输系统的重要发展方向之一ꎬ近地助推加速过程受电磁悬浮力影响ꎬ面临着复杂的地面效应与弹性稳定性问题ꎮ以NACA0012二维翼段为研究对象ꎬ建立了电磁力与气动力耦合的动力学模型ꎬ对翼段近地Ma=0~1.5加速过程中的流场特征㊁运行姿态和气动特性进行了数值模拟ꎮ结果表明ꎬ翼段加速诱导地面效应可分为4阶段ꎮ第1阶段ꎬ上下翼面为亚声速流动ꎬ翼段姿态和气动载荷基本无振荡ꎮ第2阶段ꎬ上翼面开始出现跨声速流动ꎬ下翼面流动以典型的变截面跨声速流动为主导ꎬ并伴随壅塞-通流模态转换ꎮ第3阶段ꎬ上翼面保持跨声速流动ꎬ下翼面流动壅塞再现ꎬ并呈现出完全膨胀的跨声速壅塞流动状态ꎮ在第2㊁3阶段ꎬ翼段姿态和气动载荷低频大幅振荡ꎮ第4阶段ꎬ上翼面发展为超声速流动ꎬ下翼面保持完全膨胀壅塞流动ꎬ翼段姿态和气动载荷高频小幅振荡ꎮ在此基础上ꎬ探究了悬浮高度㊁悬浮刚度㊁磁体间距对系统稳定性的影响ꎮ发现增加悬浮高度ꎬ有利于在一定程度上提高系统稳定性ꎻ适当增加悬浮刚度或悬浮磁体间距ꎬ同时限定电磁助推目标速度小于系统振荡发散临界Mach数ꎬ有利于明显提高系统稳定性ꎮ关键词:电磁发射ꎻ地面效应ꎻ弹性系统ꎻ壅塞流动ꎻ稳定性㊀㊀㊀中图分类号:V211.5㊀㊀文献标志码:A收稿日期:2023 ̄08 ̄31ꎻ修回日期:2023 ̄10 ̄09基金项目:国家自然科学基金(12072014)第一作者简介:罗星东(1993 )㊀男ꎬ硕士ꎬ主要研究电磁发射地面效应㊁飞行器内外流ꎮE ̄mail:star_east@outlook.comAbstract:Aerospacevehiclelaunchedbyelectromagneticpropulsionisapotentialoptionforfuturereusablespacetranspor ̄tationsystems.Complexgroundeffectsandstabilityissuesareinducedgenerallyduetotheintroductionofelectromagneticlevitationforce.Adynamicmodelcoupledwithelectromagneticforceandaerodynamicforcewasestablishedforthetwo ̄di ̄mensionalwing(NACA0012).NumericalsimulationswereconductedontheflowcharacteristicsꎬoperationalattitudeꎬandaerodynamiccharacteristicsofthewingduringtheMa=0~1.5accelerationprocess.Itindicatesthatgroundeffectscanbedividedintofourstages.Inthefirststageꎬsubsonicflowsarepresentedonboththeupperandlowerwingsurfacesꎬandthereisbasicallynooscillationfortheattitudeandaerodynamicloadsofthewing.Inthesecondstageꎬtransonicflowemer ̄gesontheupperwingsurfaceꎬwhiletheflowonthelowerwingsurfaceisdominatedbyatypicalvariablecross ̄sectiontransonicflowaccompaniedbythetransitionfromthechokedflowmodetotheunchokedone.Inthethirdstageꎬtheupperwingsurfacemaintainstransonicflowsꎬwhilethelowerwingsurfaceundergoeschokedflowswhicharefullyexpanded.Inboththesecondandthirdstagesꎬthewingattitudeandaerodynamicloadsoscillatesignificantlyatlowfrequencies.Inthefourthstageꎬtheupperwingsurfaceundergoessupersonicflowsꎬwhilethelowerwingsurfacemaintainschokedflows.Thewingattitudeandaerodynamicloadsoscillateslightlyathighfrequencies.Onthisbasisꎬtheeffectsofsuspensionheightꎬsuspensionstiffnessandspacingbetweensuspensionmagnetsonthesystemstabilitywereexplored.Itisfoundthatincrea ̄singthesuspensionheightisbeneficialforimprovingsystemstability.Increasingthesuspensionstiffnessorspacingbetweensuspensionmagnetsappropriatelyꎬwhilelimitingthetargetspeedofelectromagneticpropulsiontobelessthanthecritical气体物理2024年㊀第9卷Machnumberofsystemoscillationdivergenceꎬisbeneficialforsignificantlyimprovingsystemstability.Keywords:electromagneticlaunchꎻgroundeffectꎻelasticsystemꎻchokedflowꎻstability引㊀言新一代空天飞行器采用组合循环动力技术ꎬ可水平起降与重复使用ꎬ并可自由穿梭于低空㊁临近空间乃至近地轨道ꎬ是未来争夺太空经济和军事主动权的重要途径ꎮ由于飞行过程须跨越亚㊁跨㊁超㊁高超声速ꎬ所以需要兼顾飞行器在宽速域㊁大空域下的气动布局和组合动力效率等问题ꎬ这对系统设计提出了极大挑战[1]ꎮ涡波效应-乘波设计㊁机翼-乘波设计和变形/组合设计是目前发展宽速域飞行器的几种主要思路[2]ꎮ近年来ꎬ电磁发射技术逐渐成熟ꎬ为克服上述难题提供了另一种颇具潜力的解决方案[3]ꎮ利用电磁橇将飞行器在近地助推至超声速ꎬ能够有效规避低速起飞阶段ꎬ增加系统设计冗余度ꎮ国内外已对此开展了电磁助推发射方案验证和缩比模型试验验证[4]ꎮ不同于开域飞行ꎬ地面为近地流动营造了独特的半开放约束空间ꎬ通常诱发以复杂激波现象为主导的跨/超声速地面效应干扰ꎮ除空气动力学特性外ꎬ飞行器还处于电磁弹性力-气动力-结构弹性力多物理场耦合环境中ꎬ面临着复杂的气动弹性及稳定性问题ꎮ深入认知电磁助推加速地面效应并探索调控系统稳定性的手段是电磁发射技术开发的重要前提ꎮ依托地效飞行器[5ꎬ6]㊁航天器着陆[7ꎬ8]等研究背景ꎬ研究人员对低亚声速地面效应已建立起较丰富的认识[9 ̄11]ꎮMorrow等[12]通过研究发现ꎬ与低亚声速地面效应不同ꎬ跨/超声速地面效应更为复杂ꎬ以激波及其反射现象为主要特征ꎮ罗世彬等[13]综述了电磁助推发射的气动关键技术ꎬ认为飞行器-电磁橇在加速至超声速过程中ꎬ多体之间强烈的激波干扰会影响滑跑稳定性ꎮ研究人员对跨/超声速地面效应开展了必要讨论ꎮ在实验研究方面ꎬDoig等[14 ̄16]㊁Barber等[17]㊁Kleine等[18]㊁Sheridan等[19]采用镜像模型法ꎬ巧妙地消除了风洞中静止地面边界层的影响ꎬ对尖劈和子弹等简化构型在不同离地高度㊁来流速度下的超声速近地流场进行了实验ꎮ研究发现ꎬ当离地高度较小时ꎬ飞行器前缘上部呈现为脱体弓形激波ꎬ而下部因局部地面效应呈现为驻定正激波ꎮ除风洞实验外ꎬPurdon等[20]通过对比实弹射击和风洞实验结果ꎬ研究了静止地面对子弹超声速近地流场的影响ꎮ在数值模拟研究方面ꎬOliviu等[21]研究了圆柱体近地流动中的激波反射和尾涡结构ꎮGao等[22]研究了跨声速地面效应下激波-边界层干扰导致的压力脉动和抖振现象ꎮ陈晓东等[23]研究了磁悬浮助推发射装置的气动特性并进行了风洞缩比实验ꎮ肖虹等[24]计算了Ma=0.1~2.0范围内某钝头体火箭橇实验中的地面效应干扰ꎮ王伟等[25]针对细长体开展了Ma=2.5下不同攻角以及不同离地高度的地面效应研究ꎮYu等[26]进一步研究了乘波体构型在复杂地面构型下的地面效应流场和气动特性ꎮ与上述静态地面效应不同ꎬ助推加速引起的非定常地面效应更为复杂多变[27]ꎬ常伴随流场结构非定常转变和气动载荷非线性时变ꎬ并出现双解问题和迟滞现象[28 ̄30]ꎮ针对非定常地面效应流动机理的研究尚处于起步阶段ꎬ仍待深入研究ꎮ气动弹性及稳定性问题在电磁发射中同样不可忽略ꎮ已有研究主要关注气动力-结构弹性力构成的经典弹性系统ꎮ例如ꎬNuhait等[31]㊁Dessi等[32]研究了二维翼型在地面效应下的颤振特性ꎬ结果表明地面效应具有使翼型失稳的作用ꎮ张斌等[33]通过涡诱导的圆柱自由振荡算例和二维颤振标模ꎬ研究了地面效应对气动弹性动态响应和颤振边界的影响ꎮ此外ꎬ电磁弹性力的引入将使得气动弹性问题更加突出㊁独特和复杂ꎮ为突出重点和分解难点ꎬ本文基于逐步解耦的思路ꎬ选取NACA0012典型二维翼段作为研究对象ꎬ对电磁弹性力-气动力耦合作用下的翼段加速地面效应进行研究ꎬ重点关注翼段加速过程中的流场㊁姿态和气动载荷的演变规律ꎬ同时考察弹性系统参数对电磁助推稳定性的影响ꎮ1㊀气动-电磁耦合模型和数值计算方法1.1㊀物理模型和控制方程图1为考察的二维NACA0012翼段示意图ꎮ坐标系原点O与翼段质心初始位置重合ꎬX轴沿水平方向ꎬ与电磁推进方向相反ꎻY轴沿铅垂方向ꎬ竖直向上ꎻZ轴由右手法则确定ꎮ翼段上下型线对称ꎬ弦长l为1.0mꎬ质心距离翼前缘0.4mꎬ俯仰角为体轴与X轴夹角ꎬ规定抬头为正ꎬ简记为θꎬ初始时刻θ=θ0ꎮ单位展长翼段质量为82.0kgꎬ转64第1期罗星东ꎬ等:电磁助推翼段加速地面效应及稳定性分析动惯量I为11.4kg m2ꎮ电磁悬浮系统的地面模组内置于地面ꎬ翼段通过预先布置于AꎬB两处的磁体与地面模组感应产生电磁悬浮力ꎬ从而悬浮于平面轨道上ꎮ质心离地悬浮高度为hꎬ初始时刻h=0.5mꎮ电磁悬浮力与悬浮高度相关ꎮ悬浮力可简化为位于翼段上AꎬB两点的 弹簧振子 ꎮ弹簧刚度k0与电磁悬浮刚度一致ꎬ为150kN/mꎮ翼段以加速度a=100m/s2向左匀加速运动ꎬ并保留沿Y向平动和绕Z轴转动ꎮ图1㊀电磁助推NACA0012翼段受力示意图Fig.1㊀SchematicdiagramofforceanalysisoftheNACA0012wingacceleratedbyelectromagneticpropulsion对于存在运动边界(即翼段)的非定常数值模拟ꎬ采用任意Lagrange ̄Euler形式的流动控制方程求解ꎮ在控制体V内ꎬ积分形式的质量守恒㊁动量守恒和能量守恒方程通式为ddtʏVρϕdV+ʏ∂Vρϕ(u-us) dA=ʏ∂VΓ(Ñϕ) dA+ʏVSϕdV(1)式中ꎬρ是气体密度ꎻ∂V代表控制体边界ꎻA是网格面矢量ꎻϕ=(1ꎬuiꎬT)是通用变量ꎬ其中ꎬi=1ꎬ2ꎬ3ꎻu=uj是速度矢量ꎬ其中ꎬj=1ꎬ2ꎬ3ꎻus=(us1ꎬus2ꎬus3)是网格运动速度矢量ꎻΓ=(0ꎬμꎬkT/cp)是广义扩散系数ꎻSϕ=(0ꎬ∂p/∂xi+SiꎬST)是广义源项ꎮ其中ꎬμ是动力黏度ꎬkT是流体传热系数ꎬcp是比热容ꎬp是静压ꎬT是温度ꎬSi是动量方程源项ꎬST是能量方程黏性耗散项ꎮ为封闭上述方程ꎬ引入理想气体状态方程p=ρRTꎬ其中ꎬR是摩尔气体常数ꎮ上述方程中ꎬ位于运动物体表面的us项须通过求解电磁弹性力-气动力耦合的动力学方程组获得ꎮ为建立动力学模型ꎬ以下对电磁弹性力进行建模ꎬ并给出电磁弹性力-气动力耦合受力模型ꎮ对图1中翼段进行简要受力分析ꎮ翼段受气动阻力D和气动升力L作用ꎬ二者对质心产生气动力矩Mair=-OPˑ(L+D)ꎬ其中P点为气动压心ꎮ此外ꎬ电磁悬浮力F1ꎬF2分别对质心产生电磁力矩M1ꎬM2ꎮ规定电磁悬浮力㊁气动力与XꎬY轴正方向一致为正ꎬ电磁力矩和气动力矩抬头为正ꎮ则有F1(t)=-k(yA-yA0)+F1ꎬt=0(2)F2(t)=-k(yB-yB0)+F2ꎬt=0(3)M1(t)=-OAˑF1(4)M2(t)=-OBˑF2(5)式中ꎬ等号第2项F1ꎬt=0和F2ꎬt=0表示求解初始时刻弹簧振子AꎬB具有的Y向初始电磁悬浮力ꎮ因此ꎬ绕翼段质心的刚体动力学二自由度控制方程为my㊆=L+F1+F2-mg(6)Iθ㊆=Mair+M1+M2(7)式中ꎬy㊆是翼段质心垂向加速度ꎬθ㊆㊀是翼段绕质心的俯仰角加速度ꎮ对上述矢量方程进行标量化处理ꎬ则电磁悬浮力F1ꎬF2为F1=-k(yA-yA0)+F1ꎬt=0(8)F2=-k(yB-yB0)+F2ꎬt=0(9)式中ꎬyAꎬyB分别是任意时刻AꎬB点的Y向坐标ꎮ由刚体运动关系ꎬyAꎬyB可由O点平动位移y和相对O点的转动位移给定ꎮ以图1中B点为例χB=arccosOBt=0 (1ꎬ0)OBt=0(10)yB=y-OBsin(χB+Δθ)(11)式中ꎬχB是初始时刻由质心水平单位正矢量顺时针74气体物理2024年㊀第9卷转动至与OB共线时的几何夹角ꎬ值域为[0ꎬp]ꎮDθ是相对于初始时刻的俯仰角变化量ꎮ同理χA=arccosOAt=0 (1ꎬ0)OAt=0(12)yA=y-OAsin(χA+Δθ)(13)进一步地ꎬ将式(8)~(13)代入式(6)㊁(7)可得弹性系统控制方程的标量一般形式my㊆+k[2y-lBsin(χB+Δθ)-lAsin(χA+Δθ)-(yA0+yB0)]=L-mg+(F1ꎬt=0+F2ꎬt=0)(14)Iθ㊆-lAcos(χA+Δθ)ˑ{-k[y-lAsin(χA+Δθ)-yA0]+F1ꎬt=0}+lBcos(χB+Δθ)ˑ{-k[y-lBsin(χB+Δθ)-yB0]+F2ꎬt=0}=Mair(15)式中ꎬlA和lB表示AꎬB弹簧作用点与质心的距离ꎬ即lA=OAꎬlB=OBꎮ本文考察相对理想化的初始状态ꎬ假定磁体AꎬB均位于体轴上ꎬlA=0.4mꎬlB=0.6mꎬ磁体间距l0=lA+lB=1.0mꎬ翼段初始俯仰角θ0=0ꎬ则有yA0=yB0=0ꎬχA=pꎬχB=0ꎬDθ=θꎻ同时假定弹簧初始处于松弛状态ꎬ则F1ꎬt=0=F2ꎬt=0=0ꎮ则式(14)㊁(15)可写为my㊆+k(2y-lBsinθ+lAsinθ)=L-mg(16)Iθ㊆+k2(l2B+l2A)sin2θ+ky(lA-lB)cosθ=Mair(17)对于以上弹性系统ꎬ图2给出了电磁弹性力-气动力-惯性力耦合作用下的翼段近地加速非定常分步求解策略ꎮ图2㊀翼段加速过程非定常求解策略Fig.2㊀Unsteadysolutionstrategyforwingacceleration1.2 网格和计算方法翼段近地加速过程的计算域如图3所示ꎮ计算域的入口及出口均采用压力远场边界条件ꎬ并设置在远离翼段10倍弦长距离以外ꎮ时变来流的Mach数为Ma=0~1.5ꎬ压力为1atm(1atm=1.01325ˑ105Pa)㊁温度为288.15Kꎮ翼段和地面满足黏性无滑移绝热边界条件ꎬ地面速度与来流速度一致ꎮ图3㊀计算域及边界条件Fig.3㊀Computationaldomainandboundaryconditions采用重叠网格法模拟翼段近地加速过程中的沉浮和俯仰运动ꎬ流向网格尺度约5mmꎬ近壁面网格以增长率1.18发展25层ꎬ满足y+~1要求ꎮ图4展示了翼段邻域重叠网格ꎬ计算结果表明重叠交界面附近的网格匹配性较好ꎮ采用Fluent密度基隐式求解器开展非定常数值模拟ꎬ湍流模型采用k ̄ωSST模型ꎬ通量采用Roe ̄FDS格式ꎬ流场采用2阶迎风格式求解ꎬ采用CFL=1.5ꎮ在非定常计算中ꎬ时间迭代步长按照Δts=min(Δx/(u+c)ꎬ2πm/k)给定ꎬ其中Dx是流向网格尺度ꎬu是当地水平流动速度ꎬ本文计算统一取Δts=5ˑ10-5sꎮ图4㊀翼段邻域的重叠网格Fig.4㊀OversetgridsaroundtheNACA0012wing为了考察网格无关性ꎬ对来流Mach数Ma=0.8条件下不同网格尺度下NACA0012翼段定常气动力进行了对比ꎮ其中ꎬ较稀疏网格数为1.4ˑ105(流向网格尺度10mm)ꎬ中等尺度网格数为2.7ˑ105(流向网格尺度5mm)ꎬ较密集网格数为1.12ˑ84第1期罗星东ꎬ等:电磁助推翼段加速地面效应及稳定性分析106(流向网格尺度3mm)ꎮ结果表明ꎬ随着网格数量的增加ꎬ阻力㊁升力和力矩均趋于收敛ꎬ本文选取中等尺度网格开展研究ꎮ1.3㊀计算方法验证在跨/超声速阶段ꎬ激波干扰和激波-边界层干扰是典型的地面效应流动特征ꎮ采用Doig风洞实验结果[11]ꎬ对本文数值方法模拟地效的有效性进行了验证ꎮ实验来流条件为Ma=2.4ꎬRe=3.07´105ꎬα=0ʎꎬ地面效应采用固定壁板方式模拟ꎮ在相同条件下开展了数值模拟ꎬ图5给出了数值纹影和子弹表面压力系数分布ꎮ为了更加直观地对比地面效应波系的位置及形态ꎬ这里将数值纹影与实验纹影对称放置ꎮ可见ꎬ数值与实验结果吻合较好ꎬ对激波捕捉的位置相近ꎮ(a)Schlierenphotograph(b)Surfacepressuredistribution图5㊀超声速子弹地面效应的数值与实验对比ꎬMa=2.4Fig.5㊀ComparisonofgroundeffectsofthesupersonicprojectilebetweennumericalandexperimentalresultsꎬMa=2.4进一步地ꎬ采用AGARD提供的NACA0012跨声速简谐振荡实验[24]对非定常数值方法开展了验证ꎮ翼型的振荡形式如下α(t)=α0+αmsin(ωrt)(18)式中ꎬα0为平均来流攻角ꎬαm为振荡幅值ꎬωr为角频率ꎮ图6给出了NACA0012跨声速简谐振荡抬头上仰α=2.34ʎ时刻的数值与实验压力系数分布对比结果ꎮ数值计算获得的压力分布与实验数据吻合良好ꎬ说明本文数值方法对含运动边界的动态俯仰模拟具有较高精度ꎮ图6㊀攻角α=2.34ʎ姿态时翼段压力系数分布Fig.6㊀Pressurecoefficientdistributiononthewingsurfaceꎬα=2.34ʎ2㊀结果与讨论本节首先对二维NACA0012翼段在相对悬浮高度为h/l=0.5下的典型加速地面效应进行研究ꎬ以厘清近地流场㊁翼段姿态和气动载荷的演变规律ꎬ而后考察翼段悬浮高度㊁悬浮刚度㊁悬浮磁体间距3个关键因素对电磁助推加速稳定性的影响ꎮ2.1㊀翼段加速地面效应及气动力特性翼段近地电磁悬浮推进过程中的典型时刻加速流场变化如图7所示ꎮ为了便于分辨各流场中翼段相对初始位置的沉浮位移和姿态ꎬ在图7中标识O点以显示初始时刻翼段质心位置ꎮ由于弹簧振子在初始时刻处于完全松弛状态ꎬ所以也可由沉浮位移判断电磁悬浮力的方向ꎮ同时ꎬ在图7中标识箭头位置和方向以表示压心位置和升力方向ꎬΔxOP/l为质心指向压心的流向距离与弦长的比值ꎬ正值则表示压心在质心右方ꎮ图7表明ꎬ翼段在加速过程中的流场结构和受力特性复杂多变ꎬ根据速域可大致分为4个阶段ꎮ94气体物理2024年㊀第9卷(a)Ma=0.40㊀(b)Ma=0.50㊀(c)Ma=0.60(d)Ma=0.70㊀(e)Ma=0.76㊀(f)Ma=0.80(g)Ma=0.86㊀(h)Ma=0.90㊀(i)Ma=1.00(j)Ma=1.10㊀(k)Ma=1.30㊀(l)Ma=1.50图7㊀NACA0012翼段近地助推加速典型Mach数下流场压力系数云图Fig.7㊀PressurecoefficientdistributionoftheNACA0012wingatrepresentativeMachnumbers第1阶段ꎬ当Maɤ0.60ꎬ上下翼面呈现亚声速流动ꎮ自由空间内上下翼面流动基本对称ꎬ压心位置近似与质心重合ꎬ翼段姿态相对稳定ꎮ而受地面效应影响ꎬ上下翼面流动逐渐出现差异ꎬ下翼面低压区迅速增大ꎬ出现局部超声速区域(图7(c))ꎬ翼段压心前移(向左移动)并受到负升力ꎬ因此翼段下沉并低头ꎮ第2阶段ꎬ当0.60<Maɤ0.76ꎬ上翼面前缘逐渐向跨声速流动过渡并形成局部超声速低压区域ꎻ而下翼面与地面所构造的约束空间类似收缩-扩张型喷管ꎬ当来流在下翼面建立起声速喉道(图7(d))ꎬ流动产生壅塞并产生一系列压缩波向翼段前方传播ꎮ下翼面流动呈现为典型的变截面跨声速流动:亚声速-声速-超声速ꎬ但流动处于过膨胀状态ꎬ恢复激波S1位于翼段下方ꎮ但在电磁斥力及翼段前缘上方超声速低压持续作用下ꎬ翼段快速抬头并上升ꎬ喷管的收缩比减弱ꎬ下翼面流动伴随壅塞-通流状态快速转换(图7(e))ꎮ因此ꎬ下翼面出现壅塞是第1阶段向第2阶段转变的明显判据ꎮ第3阶段ꎬ当0.76ɤMa<0.90ꎬ上翼面保持跨声速流动特点ꎬ以局部超声速低压膨胀区和其后的恢复激波S2为主要特征ꎬ随着来流速度增加ꎬS2逐渐后退ꎬ尾部斜激波S3形成ꎮ在电磁拉力作用下ꎬ翼段开始下降ꎬ下翼面重新建立起壅塞ꎬ与第2阶段壅塞流动不同的是ꎬ此时激波S1已完全退出下翼面ꎬ流动处于完全膨胀状态ꎮ因此壅塞再现是第2阶段向第3阶段转变的判据ꎮ综合第2和第3阶段来看ꎬ流动的非定常特性和壅塞-通流的交05第1期罗星东ꎬ等:电磁助推翼段加速地面效应及稳定性分析替变换引起了翼段的升力方向㊁压心位置和悬浮姿态显著变化ꎬ对其运行稳定性影响明显ꎮ第4阶段ꎬ当0.90ɤMa<1.50ꎬ上翼面呈现出完全超声速流动ꎬ以此为标志流动开始进入第4阶段ꎻ下翼面持续保持壅塞ꎬ处于完全膨胀状态ꎮ当来流处于超声速速域时ꎬ翼段前方压缩波逐渐汇聚成为激波S4并向翼段靠近ꎬ下翼面壅塞流动以前缘脱体正激波为主要特征ꎬ翼段下半部多余流量从翼段前缘溢流口向翼段上半部泄流ꎮ该阶段ꎬ翼段受超声速地面效应影响ꎬ压心位置和悬浮姿态相对稳定ꎬ受到正升力和电磁拉力ꎬ翼段上浮ꎮ与流场分析相对应ꎬ图8给出了加速过程中的翼段质心垂向位移和俯仰角的演变曲线ꎮ可以看出ꎬ在第1阶段ꎬ质心垂向位移和俯仰角曲线较为顺滑ꎮ质心高度不断下降ꎬ最大位移量为-0.1mꎬ翼段连续低头ꎬ最小俯仰角约为-3.8ʎꎮ在第2ꎬ3阶段ꎬ由于气动压心 后移-前移-后移 和升力 负-正 的迅速转变ꎬ该阶段内的质心位移和俯仰角曲线出现明显振荡ꎮ特别地ꎬ对于第2阶段ꎬ在下翼面壅塞消失过程中ꎬ原本宽泛的低压区迅速缩小直至消失(图7(e))ꎬ前缘压力得到恢复ꎬ同时前缘上表面局部超声速低压区开始显现ꎬ这一高一低造成翼段整体抬头趋势较明显ꎮ而当处于第3阶段ꎬ壅塞再现ꎬ随着上翼面恢复激波S2的后退和低压区的扩大ꎬ上下翼面流动特征趋近并相对稳定ꎬ压心始终位于质心附近ꎬ抬头趋势被抑制ꎬ俯仰角和质心位移整体保持平稳振荡ꎮ在第4阶段ꎬ质心位移和俯仰角的平均值先基本不变后略微降低ꎬ曲线的振幅明显减弱但频率明显升高ꎮ进一步地ꎬ对助推加速过程中的翼段气动力/力矩系数进行研究ꎬ其中参考面积为Sreff=1m2ꎮ从图9可以看出ꎬ翼段气动载荷变化规律和图8中姿态变化规律相近ꎬ图8㊀翼段质心位移和俯仰角演变Fig.8㊀Evolutionofcentroiddisplacementandpitchangleofthewing图9㊀翼段气动载荷系数演变Fig.9㊀Evolutionofaerodynamicforcecoefficientsofthewing因此ꎬ亚声速地面效应对翼段运行姿态的影响特点可简要总结为基本无振荡ꎻ跨声速地面效应诱导翼段运行姿态低频大幅振荡ꎻ而超声速地面效应诱导翼段运行姿态高频小幅振荡ꎮ2.2㊀翼段稳定性分析2.2.1㊀气动-电磁弹性系统稳定性讨论通过前述分析发现ꎬ在跨/超声速运行阶段尤其是第2ꎬ3阶段ꎬ流场变化显著ꎬ翼段受到较强的非定常气动载荷冲击ꎬ表现出大幅度周期性振荡ꎬ容易产生稳定性问题ꎮ实际上ꎬ电磁悬浮力可认为是弹性力ꎬ这与传统的气动力-结构弹性力所构成的气动弹性问题类似ꎮ因此ꎬ本节从方程相似角度对悬浮加速运行稳定性进行分析ꎮ无外力干扰下的经典二元翼段弹性体颤振控制方程[34]分别由质心动力学方程和绕动点刚心E的转动方程建立ꎬ可写为m(y㊆E-θ㊆Exa+g)+CyEy +KyEy=L=QyE(19)IEθ㊆E-m(y㊆E+g)xa+CθEθE+KθEθ=Mair-Lxa=QθE(20)式中ꎬ广义坐标yE和θE分别是弹性体刚心的沉浮位移和绕刚心的俯仰角ꎻIE表示绕刚心转动惯量ꎻxa为质心与刚心距离且质心位于刚心后方ꎻCyE和CθE分别为翼段弹性体刚心沉浮和俯仰阻尼系数ꎻMair是对质心的气动力矩ꎻKyE和KθE分别为翼段弹性体沉浮和俯仰刚度系数ꎻ两式等号右边QyE和QθE分别对应刚心气动升力和刚心气动力矩ꎮ相较之下ꎬ虽然本文所考察的电磁弹性力-气动力系统(式(16)~(17))暂不考虑结构弹性和结构阻尼ꎬ即已略去与xa和结构阻尼有关的量ꎬ但由于有电磁外部力的约束且不显含广义坐标θꎬ也具有一定复杂度ꎮ特别地ꎬ在小攻角振荡假设下ꎬ式15气体物理2024年㊀第9卷(16)~(17)可改写为m(y㊆+g)+2ky+k(lA-lB)θ=L(21)Iθ㊆+k(l2A+l2B)θ=Mair-k(lA-lB)y(22)将式(19)~(20)与式(21)~(22)对比ꎬ不难发现ꎬ由电磁弹性力-气动力耦合模型推导出的弹性动力学控制方程与经典气动弹性控制方程形式类似ꎮ主导电磁悬浮近地加速系统的不稳定性因素主要存在3项ꎮ一是悬浮高度hꎬ该项直接影响地面效应和相关气动载荷ꎬ即方程等号右边的升力项L和力矩项Mairꎮ二是悬浮刚度kꎬ其直接影响了系统的刚度ꎮ三是悬浮磁体间距ꎬ由lA和lB决定ꎮ以下分别对悬浮高度㊁悬浮刚度和磁体间距对系统稳定性的影响开展研究ꎮ2.2.2㊀悬浮高度对系统稳定性的影响控制磁体间距和悬浮刚度不变ꎬ研究了不同初始相对悬浮高度h/l=0.5ꎬ1.0ꎬ1.5对系统稳定性的影响ꎮ图10给出了不同相对悬浮高度下翼段近地加速过程中质心位移和俯仰角演变曲线ꎮ可以看到ꎬ当增加悬浮高度ꎬ由于下翼面阻塞度减小ꎬ流动产生壅塞所需的Mach数增加ꎬ地面效应减弱ꎬ因此第1阶段向第2阶段的转变Mach数明显延后ꎬ但质心位移和俯仰角曲线的振荡幅度在不同悬浮高度下仍基本一致ꎮ此外ꎬ不同悬浮高度下ꎬ第3阶段向第4阶段转变的阈值基本不变ꎮ这主要是由于第3向第4阶段转变的标志是恢复激波S2退出上翼面ꎬ而不同离地悬浮高度下上翼面流动特征基本一致ꎮ(a)Centroiddisplacement㊀㊀㊀(b)Pitchangle图10㊀不同悬浮高度下翼段加速质心位移和俯仰角演变Fig.10㊀Evolutionofcentroiddisplacementandpitchangleofthewingatdifferentsuspensionheights上述结果表明ꎬ增加悬浮高度能够缩短跨/超声速地面效应气动激励的作用时间ꎮ并且ꎬ翼段在第4阶段的质心垂向高度下降更快ꎬ俯仰角曲线振荡的平均值基本趋于0ʎ攻角ꎬ但振幅略有增加ꎮ从系统稳定性来看ꎬ适当增加悬浮高度有利于减弱地面效应ꎬ从而提高系统稳定性ꎮ但考虑到工程可实现性ꎬ悬浮高度的可调节范围受诸多限制ꎬ对于实际工程应做进一步讨论ꎮ2.2.3㊀悬浮刚度对系统稳定性的影响控制悬浮高度和磁体间距不变ꎬ通过在悬浮刚度k0基准上依次扩大10倍和50倍ꎬ研究不同悬浮刚度下的系统稳定性ꎮ图11给出了悬浮刚度k=k0ꎬ10k0ꎬ50k0下翼段近地加速过程中质心位移和俯仰角演变曲线ꎮ图11表明ꎬ当增加悬浮刚度至10k0ꎬ50k0时ꎬ在加速前期阶段ꎬ翼段的质心沉浮位移和俯仰角的振荡幅度能够被有效抑制ꎬ位移振荡范围均限制在ʃ10mm区间ꎮ但较大的悬浮刚度反而导致翼段更高频的振荡ꎬ直至发散ꎬ其中俯仰角最大值约40ʎꎬ远远超过失速迎角ꎬ严重破坏了系统稳定性ꎮ并且ꎬ悬浮刚度50k0相比10k0工况的发散更加提前ꎬ出现在亚声速域ꎮ(a)Centroiddisplacement25第1期罗星东ꎬ等:电磁助推翼段加速地面效应及稳定性分析(b)Pitchangle图11㊀不同悬浮刚度下翼段加速质心位移和俯仰角演变Fig.11㊀Evolutionofcentroiddisplacementandpitchangleofthewingatdifferentsuspensionstiffness为解释系统发散现象ꎬ进一步地ꎬ对不同悬浮刚度下翼段加速过程中的沉浮位移和俯仰角进行时频分析ꎮ图12给出了加速过程中各频率翼段沉浮及俯仰运动随Mach数变化的能量分布ꎮ其中ꎬ红色区域表征翼段运动能量集中频率ꎬ红色长虚线和短虚线分别表示无气动激励下翼段自由振荡的1阶及2阶固有频率ꎮ可见ꎬ当悬浮刚度为k0时ꎬ翼段沉浮与俯仰运动的频率均偏离自由振荡系统固有频率ꎮ翼段的运动主要受到气动力/力矩周期性脉动激励ꎮ随着Mach数增大ꎬ气动激励由亚声速阶段的简单低频激励(约1Hz)发展为跨声速阶段的复杂低频激励(1Hz与8Hz共存)ꎻ在超声速阶段ꎬ激励频率进一步升高ꎬ并引发系统以高于2阶固有频率的振动(20Hz左右)ꎮ当悬浮刚度增大至10k0时系统悬浮刚度加强ꎬ悬浮力足以克服亚跨声速阶段的气动激励ꎬ但随着固有频率升高ꎬ气动激励与系统2阶模态发生耦合ꎬ加之悬浮系统阻尼不足ꎬ诱发系统以2阶特征频率发生颤振式的不稳定振动ꎮ当悬浮刚度增大至50k0时ꎬ系统1阶与2阶固有频率进一步升高并趋近ꎬ气动激励同时与1阶㊁2阶模态发生耦合ꎬ从而诱发系统提前在Ma=0.8时发生不稳定振动ꎮ从另一方面讲ꎬ若减小电磁助推加速的目标速度至系统发散前(如Ma=1.2)ꎬ限定系统悬浮刚度k0<k<10k0ꎬ则在该范围内增加悬浮刚度能够显著减弱系统振荡幅度ꎬ提高系统稳定性ꎮ2.2.4 悬浮磁体间距对系统稳定性的影响控制悬浮高度和悬浮刚度不变ꎬ进而研究不同悬浮磁体间距下(lm=lA+lB=0.5l0ꎬ1.0l0ꎬ2.0l0)的系统稳定性ꎮ式(16)~(17)表明ꎬ增加磁体的前后纵向间距ꎬ可以增大电磁悬浮力的力臂ꎬ从而增加系统的俯仰刚度ꎮ图13给出了不同磁体间距下翼段近地加速过程中质心位移和俯仰角演变曲线ꎮ结果表明ꎬ增加悬浮磁体间距ꎬ能够大幅抑制加速过程中的质心沉浮位移和俯仰角的振荡幅度ꎬ但翼段在第4阶段的振荡频率略有升高ꎮ减小悬浮磁体间距ꎬ翼段出现明显沉浮和俯仰振荡的速域提前ꎬ并表现为低频大幅振荡ꎮ(a)k=k0(b)k=10k035。


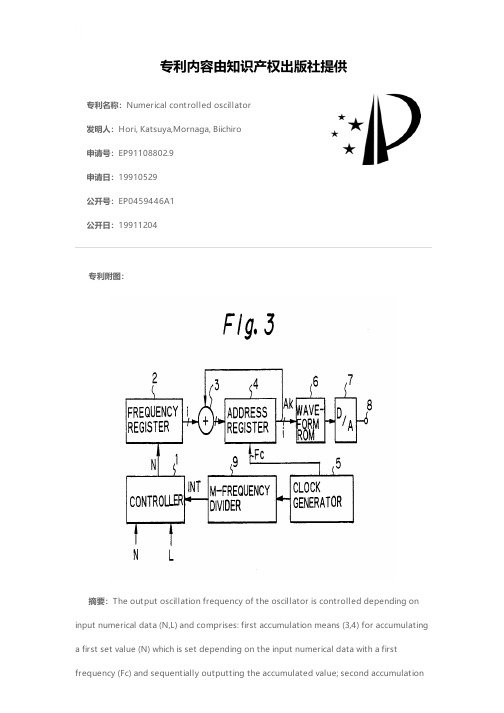

第 62 卷第 5 期2023 年9 月Vol.62 No.5Sept.2023中山大学学报(自然科学版)(中英文)ACTA SCIENTIARUM NATURALIUM UNIVERSITATIS SUNYATSENI具有间断解的PTT型黏弹性流体热对流数值模拟*张栏1,蒋子超1,陈玉惠1,张仪2,杨耿超1,姚清河11. 中山大学航空航天学院,广东深圳 5181072. Robotics and Mechatronics, University of Twente, Enschede 7522 NB, the Netherlands摘要:从间断解充分发展的黏弹性流体Rayleigh-Bénard(RB)热对流具备不同流态的时间信息和发展过程的流态变换,研究该问题对阐明Phan-Thien-Tanner(PTT)型黏弹性流体RB热对流的流动机理具有重要意义。
本文首次采用二阶迎风型总变差不增差分格式对PTT型黏弹性流体的RB热对流进行了数值模拟,并基于与解析解的对比论证了该数值方法在空间离散上的收敛性与二阶精度。
数值结果表明,本文的数值方法可模拟自间断初始场演化的PTT型黏弹性流体RB热对流,此外,本文还对该流动发展到稳态周期解的瞬态流动现象作了基本阐释,流动现象与从先验速度场发展的流动现象一致。
关键词:间断解;总变差不增格式;黏弹性流体;Phan-Thien-Tanner模型;Rayleigh-Bénard对流中图分类号:O373 文献标志码:A 文章编号:2097 - 0137(2023)05 - 0136 - 09Numerical simulation of thermal convection ofPTT viscoelastic fluid with discontinuous solutionZHANG Lan1, JIANG Zichao1, CHEN Yuhui1, ZHANG Yi2, YANG Gengchao1, YAO Qinghe11. School of Aeronautics and Astronautics,Sun Yat-sen University,Shenzhen 518107,China2. Robotics and Mechatronics, University of Twente, Enschede 7522 NB, the NetherlandsAbstract:The study of the time information and transitional behavior of flow states in the develop‐ment of intermittent solutions in Rayleigh-Bénard (RB) convection of viscoelastic fluids has great sig‐nificance in understanding the flow mechanics of Phan-Thien-Tanner (PTT) viscoelastic fluid RB con‐vection. In this paper,we first use a second-order upwind-type total variation diminishing (TVD)scheme to numerically simulate PTT viscoelastic fluid RB convection,and demonstrate the conver‐gence and second-order accuracy of the numerical method in spatial discretization based on the compar‐ison between the numerical and analytical solutions. The numerical results indicate that the numerical method employed in this paper can simulate the evolution of PTT viscoelastic fluid RB thermal convec‐tion with discontinuity initial field, and provide a fundamental explanation for the transient flow phe‐nomena of this flow up to the development of steady periodic solutions, which are consistent with the flow phenomena developed from the a priori velocity field.Key words:discontinuous solution;total variation diminishing scheme;viscoelastic fluid;Phan-Thien-Tanner model; Rayleigh-Bénard convectionDOI:10.13471/ki.acta.snus.2023D008*收稿日期:2023 − 02 − 22 录用日期:2023 − 03 − 28 网络首发日期:2023 − 06 − 19基金项目:国家重点研发计划(2018YFE9103900);国家自然科学基金(11972384);广东省基础与应用基础研究基金(2021B1515310001,2022B1515120009)作者简介:张栏(1998年生),男;研究方向:计算流体力学;E-mail:*********************通信作者:姚清河(1980年生),男;研究方向:计算流体力学、大规模并行算法;E-mail:****************第 5 期张栏,等:具有间断解的PTT型黏弹性流体热对流数值模拟热对流是由温度差驱动的流体运动,是自然界中普遍而重要的现象,广泛存在于海洋、大气、地核以及恒星和行星的内部。

收稿日期:2014-05-20。
基金项目:国家自然科学基金资助项目(51266010);江西省科技支撑计划资助项目(20123BBG70195)。
作者简介:魏昊然(1991—),男,硕士生;通信作者:彭冬根(1975—),男,副教授,博士。
引文格式:魏昊然,彭冬根,张景欣,等.太阳能溶液集热/再生器数值模拟分析[J ].南昌大学学报:工科版,2014,36(3):252-256,292.文章编号:1006-0456(2014)03-0252-05太阳能溶液集热/再生器数值模拟分析魏昊然,彭冬根,张景欣,申传涛(南昌大学建筑工程学院,江西南昌330031)摘要:太阳能溶液集热/再生器是太阳能溶液除湿制冷空调系统中的核心部分,通过数值模拟的方法,对空气入口温度、湿度,溶液入口温度、浓度,环境温度等因素对太阳能集热/再生器的再生效率的影响进行了数值模拟分析。
结果表明:将环境温度、入口空气温度、入口溶液温度由20ħ增大至40ħ后,对应的再生效率分别上升了0.745%、3%、93%。
提高入口溶液温度能够最大提升太阳能溶液集热/再生器的再生效率。
随着通入空气的流量的增加,空气流量由0.02kg /s 增加至0.14kg /s ,再生效率增加了31%左右,而随着入口溶液浓度由0.30增加至0.38,再生效率由0.55下降至0.43,效率下降了22%。
随着太阳辐射由0.5kW /m 2提升至1.5kW /m 2,再生效率由0.58下降至0.44,效率下降了24.13%。
关键词:太阳能溶液集热/再生器;数值模拟;入口参数;再生效率中图分类号:TK519文献标志码:ANumerical simulation analysis of solar liquid collect or /regeneratorWEI Haoran ,PENG Donggen ,ZHANG Jinxin ,SHEN Chuantao(School of Civil Engineering and Architecture ,Nanchang university ,Nanchang 330031,China )Abstract :Solar liquid collector /regenerator is the core part of solar liquid desiccant cooling air conditioning system.The numerical simulation analysis was carry out for stduy the impacts of air inlet temperature and humidity ,liquid inlet temperature and concentration ,environment temperature on the regeneration efficiency of solar liquid collector /regenerator by numerical simulation method.The results showed that the corresponding regeneration effi-ciency is increased 0.745%,3%,93%with increasing of the environment temperature ,inlet air temperature ,inlet liquid temperature from 20ħto 40ħ.The regeneration efficiency of solar liquid collector /regenerator can be maximized with increasing of inlet liquid temperature.With the aeration flow is increased ,air flow is increased to 0.14kg /s from 0.02kg /s ,regeneration efficiency is about increased 31%,with inlet liquid concentration is in-creased to 0.38from 0.3,regeneration efficiency is decreased to 0.43from 0.55,the efficiency is decreased 22%.With solar radiation is increased to 1.5kW /m 2from 0.5kW /m 2,regeneration efficiency is decreased to 0.44from 0.58,efficiency is decreased 24.13%.Key Words :solar liquid collect or /regenerator ;numerical ;inlet parameter ;regeneration efficiency 随着经济的快速发展和人们生活水平的提高,建筑能耗占总能耗的比例在逐年增加。

NUMERICAL SIMULATION OF COUPLED HEAT AND MASS TRANSFER IN HYGROSCOPIC POROUS MATERIALS CONSIDERING THE INFLUENCE OF ATMOSPHERIC PRESSURE Li FengzhiDepartment of Engineering Mechanics,Dalian University of Technology,Dalian,China and Institute of Textiles and Clothing,The Polytechnic University of Hong Kong,Hung Hom,Hong KongLi YiInstitute of Textiles and Clothing,The Polytechnic University of Hong Kong,Hung Hom,Hong KongLiu YingxiDepartment of Engineering Mechanics,Dalian University of Technology,Dalian,ChinaLuo ZhongxuanDepartment of Applied Mathematics,Dalian University of Technology,Dalian,ChinaA model of simultaneous transport in hygroscopic porous materials was developed.Water in fabrics is considered to be present in three forms:liquid water in the void space between fibers,bound water in the fibers,and vapor.It is assumed that the heat and mass transport mechanisms include movement of liquid water due to the capillarity and atmospheric pressure gradient,diffusion of vapor within interfibers due to the partial pressure gradient of vapor and total gas pressure gradient,diffusion of vapor into fiber,evaporation,and condensation of water.The moisture diffusion process into hygroscopic porous materials such as wool fabrics was simulated.At normal atmospheric pressure,the results were compared with experimental data on the temperature and water content changes reported in the literature.The distribution of temperature,moisture concentration,liquid water saturation,and atmospheric pressure in the void space between fibers at different boundary conditions are numerically computed and compared.The conclusion is that atmospheric pressure gradient has significant impact on heat and mass transport processes in hygro-scopic porousmaterials.Received 13March 2003;accepted 16July 2003.We would like to thank The Hong Kong Polytechnic University for funding this research throughprojects T612and A188in the ASD in Fashion,Design and Technology Innovation.Address correspondence to Li Yi,Institute of Textiles and Clothing,The Polytechnic University ofHong Kong,Hung Hom,Hong Kong.E-mail:tcliyi@.hkNumerical Heat Transfer,Part B ,45:249–262,2004Copyright #Taylor &Francis Inc.ISSN:1040-7790print/1521-0626online DOI:10.1080/104077904902688142491.INTRODUCTIONThe clothing system plays a very important role in determining the human body core temperature and other human thermal responses because it determines how much of the heat generated in the human body can be exchanged with the environment.With the development of new technology,it is becoming important to know how the human body will behave thermally under different environmental conditions with various clothing systems.In order to obtain a comfortable micro-climate for human body,it is necessary to understand the thermal and moisture transport behavior of the clothing.The first clothing model that describes the mechanism of transient diffusion of heat and moisture transfer into an assembly of hygroscopic textile materials was introduced and analyzed by Henry [1].He developed a set of two differential coupled governing equations for the mass and heat transfer in a small flat piece of clothingNOMENCLATUREc v volumetric heat capacity of the fabric,J =m 3Kc vf volumetric heat capacity of fiber,J =m 3KC fwater vapor concentration in the fibers of the fabric,kg =m 3div divergenceDdiffusivity of vapor,m 2=sD f ðw c ;t Þdiffusion coefficient of water vapor in the fibers grad gradienth c convection mass transfer coefficient,m =sh l $g mass transfer coefficient,m =sh t convection heat transfer coefficient,J =m 2Kh vap latent heat of evaporation =condensation,J =kgk mix thermal conductivity of the fabric,W =m KK intrinsic permeability,m 2K rg relative permeability of water vapor K rw relative permeability of liquid water L thickness of fabricm a mass flux of dry air under gas pressure gradient driving m v mass flux of vapor under gas pressure gradient drivingm D v diffusion mass flux of water vapor m w diffusion mass flux of liquid water M w mole mass of water vapor,kg =mol p a dry air partial pressure,kg =m s 2p c capillary pressure,kg =m s 2p g pressure of gas phase,kg =m s 2p v water vapor partial pressure,kg =m s 2rradius,mS liquid water volumetric saturation (liquid volume =pore volume)S v specific area of the fabric,l =m T temperature of the fabric,K T 1environmental temperature,KWevaporation or condensation flux of water in interfiber void space of fabric,kg =m 3sw c water content of the fibers in the fabric,kg =kg G boundarye porosity of fabricl heat of sorption or desorption water or vapor by fibers,J =kgm g dynamic viscosity of gas,kg =m s m w dynamic viscosity of water,kg =m s r a density of dry air,kg =m 3r w density of liquid water,kg =m 3r vwater vapor concentration in the air filling the interfiber void space,kg =m 3r vs saturated water vapor concentration,kg =m 3r v ?environmental water vapor concentration,kg =m 3Superscripts n previous time n þ1present time Subscripts 0initial value 1left boundary N right boundary ?environment250L.FENGZHI ET AL.SIMULATION OF COUPLED HEAT AND MASS TRANSFER251 material.The analysis of Henry was based on a simplified analytical solution. Downes and Mackay[2]and Watt[3]found experimentally that the sorption of water vapor by wool is a two-stage process.In order to describe the complicated process of the two-stage adsorption behavior in textile materials,Nordon and David [4]presented a model in terms of experimentally adjustable parameters appropriate for thefirst and second stages of moisture sorption.However,their model did not take into account the physical mechanisms of the process.For this reason,Li and Holcombe[5]introduced a new two-stage absorption model to better describe the coupled heat and moisture transport in fabrics.Li and Luo[6]improved the sorption rate equation by assuming that the moisture sorption by a woolfiber can be generally described as a uniform-diffusion equation for both stages of sorption.Luo et al.[7]presented a dynamic model of heat and moisture transfer with sorption and condensation in porous clothing assemblies.Their model considers the effect of water content in the porousfibrous batting on the effective thermal conductivity as well as radiative heat transfer.However,the above-mentioned models ignore the effect of liquid water movement.Fan and Wen[8]reported a model,in which eva-poration and mobile condensates are considered.Li and Zhu[9]reported a new model that takes into account the condensation=evaporation and liquid diffusion by capillary action,which is a function offiber surface energy,contact angle,and fabric pore size distributions.Furthermore,they investigate the effects of the pore size distribution,fiber diameter[10],thickness and porosity[11]on the coupled heat and moisture transfer in porous textiles.Wang Zhong et al.[12]reported a model con-sidering the radiation and conduction heat transfer coupled with liquid water transfer,moisture sorption,and condensation in porous polymer materials.How-ever,in the above references[1–12],the models were based on the mass diffusion due only to the concentration gradient;the effect of the atmospheric pressure gradient on heat and moisture transfer in porous materials was ignored.For example,when a person runs,the atmospheric pressure between the inside and the outside of clothing is different,which may have significant impact on the thermal comfort and protec-tion of clothing.Although the effects of the atmospheric pressure gradient on mass transfer in porous media were considered by previous researchers[13,16],these models are established for nonhygroscopic materials.In order to investigate the influence of atmospheric pressure gradient on heat and mass transfer within hygroscopic textile materials,and to provide insights into the functional design of clothing for windy conditions and active sportswear outdoors,we report a new model by introducing new equations=terms and integrating them.2.MATHEMATICAL FORMULATIONA schematic diagram of the physical mechanisms within fabric is shown in Figure1.To obtain the governing equation,we have made the following assump-tions.First,the clothing is isotropic,and the gas phase is considered to be an ideal gas composed of dry air and water vapor,which are regarded as two miscible species. Second,local thermal equilibrium exists among all phases.This is reasonable,as the pore dimension in the textile material is small.Third,volume changes of thefibers due to changing moisture content are neglected.Fourth,diffusion within thefiber is considered to be so slow that the moisture content at thefiber surface is always insorptive equilibrium with that of the surrounding air.Fifth,water in fabrics is considered to be present in three forms:free liquid water in the void space of interfibers,bound water in the fibers,and water vapor.The mass transport mechanisms are movement of free liquid water due to the capillarity and atmo-spheric pressure gradient,diffusion of vapor in the space between fibers due to the partial pressure gradient of vapor and total gas pressure gradient,and diffusion of vapor in the fiber due to sorption =desorption.Based on the above assumptions,we can establish the following mathematical equations for the coupled heat and mass transfer in the clothing according to the conservation of masses and heat energy:q E ð1ÀS Þr v ½ ½ q t þq C f 1ÀE ðÞÂÃq t ÀE ð1ÀS ÞS v h l $g r vs ðT ÞÀr v ½ ¼div ðÀm D v Þþdiv ðÀm v Þq ½E S r wq t þE ð1ÀS ÞS v h l $g r vs ðT ÞÀr v ½ ¼div ðÀm w Þq E ð1ÀS Þr a ½ ½ q t ¼div ðm D v Þþdiv ðÀm a ÞC v q T q t Àl ð1ÀE Þq C f q t þh vap E ð1ÀS ÞS v h l $g r vs ðT ÞÀr v ½ ¼div ½k mix ðgrad T Þ :8>>>>>>>>>>>>>>>>><>>>>>>>>>>>>>>>>>:ð1ÞHere,E is porosity;S is saturation of free liquid water;S v is the specific area of thefabric;r v is water vapor concentration;r a is the concentration of dry air;r w is the density of liquid water;C f is the water vapor concentration in the fibers;h l $gisFigure 1.Schematic diagram of the physical model.252L.FENGZHI ET AL.the mass transfer coefficient;r vsðTÞis the concentration of saturated water vapor at T;h vap is the latent heat of evaporation=condensation;l is the sorption heat of thefiber;m v is the massflux of vapor under total atmospheric pressure gradient;m Dv isthe diffusion massflux of water vapor;m a is the massflux of dry air under total atmospheric pressure gradient;m w is the diffusion massflux of liquid water;c v is the effective volume heat capacity;and k mix is the effective thermal conductivity of the fabric.Thefirst equation in(1)represents the mass balance of water vapor.Thefirst term on the left side represents vapor storage within the void space of the interfiber; the second term represents water vapor accumulation rate within thefiber,i.e.,the sorption rate offibers;whereas the third term is evaporation or condensationflux of the water in the interfiber void space.The right side of the equation represents water-vapor diffusion.Thefirst term denotes the effect of the vapor diffusion under vapor partial pressure gradient,whereas the second term represents the effect of the vapor diffusion under total gas pressure gradient driving.Similarity,the second equation represents the mass balance of liquid water,and the third equation represents the mass balance of dry air.The fourth equation in(1)represents the energy balance. Thefirst term on the left side of the fourth equation represents energy storage, whereas the second and third terms represent sorption latent heat of water vapor by fibers and the latent heat of liquid water within interfibers,respectively.The right side represents the heat conduction.Sorption and desorption of moisture by thefibers obey the Fickian Law[6]:q C f q t ¼1rqq rrD fðw c;tÞq C fq r!ð2Þwhere D fðw c;tÞis the diffusion coefficient,which has different presentation at dif-ferent stages of moisture sorption;C f is the moisture concentration in thefiber; w c is the bound water content in thefibers.The boundary condition around thefiber is determined by assuming that the moisture concentration at thefiber surface is instantaneously in equilibrium with the surrounding air.Hence,the moisture concentration at thefiber surface is determined by the relative humidity of the surrounding air and temperature,i.e.,[6]C fðR fÞ¼fðRH;TÞð3Þwhere f is the moisture sorption isotherm of thefibers,which can be determined experimentally for differentfibers.The pore sizes infibrous materials are generally small,so that the diffusion of flow is governed by Darcy’s law[1].In the pores formed betweenfibers,we have the massflux of water vapor and dry air under total atmospheric pressure gradient, respectivelym v¼Àr v KK rgm ggradðp gÞÂÃð4Þm a¼Àr a KK rgm ggradðp gÞÂÃð5ÞSIMULATION OF COUPLED HEAT AND MASS TRANSFER253where p g is the atmospheric pressure,K is intrinsic permeability,K rg is relative permeability of water vapor,and m g is the gas-phase dynamic viscosity.For the free liquid water phase,Darcy’s law is expressed as[13]m w¼Àr w KK rwm wgradðp gÀp cÞð6Þwhere K rw is the relative permeability of liquid water,m w is the dynamic viscosity of the liquid water,and p c is the capillary pressure.For the water-vapor phase,diffusion massflux under partial pressure gradient is expressed as[13]m Dv ¼À1:952Â10À7eð1ÀSÞÂT0:8p agradðp vÞð7Þwhere p a is the partial pressure of dry air and p v is the partial pressure of water vapor.In order to generate a solution to the equations mentioned above,we need to specify an initial condition and boundary conditions on the fabric surfaces in terms of concerntration,saturation,temperature,and atmospheric pressure.Initially,a fabric is equilibrated to a given atmospheric pressure,temperature,saturation,and humidity;the temperature,atmosphere,saturation,and concentration are uniform throughout the clothing at known value.T¼T0r v¼r v0S¼S0p g¼p g0C f¼fðRH0;T0Þð8ÞThe boundary conditions arem DvÁ n j GþmÁ n j G1¼h cðr vÀr v1ÞS jG1¼S B;m wÁ n j g12¼qð9ÞK mix grad TÁ n j G¼h tðTÀT1Þp g j G¼p g Gwhere G denotes the boundary,h c is the mass transfer coefficient,h t is the convective heat transfer coefficient,and the subscript1denotes the environment.3.DISCRETIZATION OF THE CONTINUUM EQUATIONTo derive a numerical solution for Eqs.(1),(8),and(9)by using thefinite-volume method,we select a well-distributed control volume with D x,as shown in Figure2.For example,thefirst equation of(1)can be rewritten as follows:A qr vq tþBq Sq tþCþD¼qq xEqr vq xþqq xFq Tq xþqq xGq p gq xð10ÞwhereA¼eð1ÀSÞB¼Àer v C¼e f q C fq tD¼Àeð1ÀSÞh lg S v r vsðTÞÀr v½254L.FENGZHI ET AL.E ¼ð1:952e À7Þe ð1ÀS ÞT 0:8p a RTM wF ¼ð1:952e À7Þe ð1ÀS ÞT 0:8p a R r vM wG ¼r vKK rg m gIf the control volume P is located in the inner of zone,integration of Eq.(10)over the control volume givesZ Or A qr v q t þB q S q t þC þDdx ¼Z Orq q x E qr v q x þq q x F q T q x þq q x G q p gq x !dxð11ÞThenA n p r n þ1vp Àr n v D t D x þB n p S n þ1vp ÀS n vp D t D x þC n pD x þD np D x ¼E qr v q x eÀE qr v w þF q T e ÀF q T w þG q p g e ÀGq p gwð12ÞNow the right-hand side of Eq.(12)can be written asE qr v q x e ÀE qr v q x w þF q T q x e ÀF q T q x w þG q p g q x e ÀGq p g q xw¼E n e r n þ1v E Àr n þ1v pD xÀE n wr n þ1v p Àr n þ1v WD xþF n eT n þ1EÀT n þ1p D xÀF n wT n þ1p ÀT n þ1W D x þG n e p n þ1gE Àp n þ1gp D x ÀG n wp n þ1gpÀp n þ1gW D xð13ÞWith the new symbols,Eq.(12)becomesK w v r n þ1v W þK wt T n þ1W þK wg p n þ1gW ÀK p v r n þ1v P ÀK ps S n þ1P ÀK pt T n þ1P ÀK pg p n þ1gPþK e v r n þ1v EþK et T n þ1EþK eg p n þ1gE¼RRð14ÞFigure 2.Schematic map of control volume.SIMULATION OF COUPLED HEAT AND MASS TRANSFER 255where K wv¼m E n w,K wt¼m F n w,K wg¼m G n w,K pv¼m E n wþm E n eþA n p,K pt¼m F nw þm F n e,K ps¼B n p,K pg¼m G n wþm G n e,K cv¼m E n e,K et¼m F n e,K eg¼m G n e,RR¼ÀA np r nvpÀB n p S n pþC n p D tþD n p D t.If control volume P is located on the left boundary,we consider that the integration area is half of the inner volume andE qr vq xþFq Tq xþGq p gq xP ¼h cl r nþ1vpÀr vp1ð15ÞThen,we can obtainÀK pv r nþ1vP ÀK ps S nþ1PÀK pt T nþ1PÀK pg p nþ1gPþK ev r nþ1vEþK et T nþ1EþK eg p nþ1gE¼RRð16Þwhere m¼D t=D x2,Z¼D t=D x,K pv¼2m E n eþA n pþ2Z h cl,K pt¼2m F n e, K ps¼B n p,K pg¼2m G n e;K ev¼2m E n e,K et¼2m F n e,K eg¼2m G n e,RR¼ÀA n p r n vpÀB n p S n pþC n pD tþD npD tÀ2Z h el r vp1.Similarly,if control volume P is located on the right boundary:K w v r nþ1n W þK wt T nþ1WþK wg p nþ1gWÀK p v r nþ1n PÀK ps S nþ1PÀK pt T nþ1PÀK pg p nþ1gP¼RRð17Þwhere m¼D t=D x2,Z¼D t=D x,K w v¼2m E n w,K wt¼2m F n w,K wg¼2m G n w, K p v¼2m E n wþA n pþ2Z h cN,K pt¼2m F n w,K ps¼B n p,K pg¼2m G n w;RR¼ÀA n p r n n pÀB n p S npþC n p D tþD n p D tÀ2Z h cN r n p1N,where h cN is the mass transfer coefficient in theright boundary,and r n p1N is the concentration of right environment water vapor.Following the same procedure as for the water-vapor mass conservation,we can derive the discretization equations for liquid water,energy,and dry air.By specifying initial conditions we can calculate the coefficient of the discretization and its right term,the values of water-vapor concentration,liquid water saturation, temperature,and atmospheric pressure at next time,can be obtained.The dis-cretization errors of these schemes are O½ðD xÞ2þðD tÞ2 .And the full implicit scheme is stable without any restriction on D t=D x2.In order to obtain grid independence of the solution,we selected grids of different sizes and did computations.The difference in solution betweenfine and coarse grids with increase of size by2is less than2%.4.NUMERICAL SIMULATION AND DISCUSSIONFor computation,the values of the main parameters are listed as Table1.parison between Theoretical Predictions and Experimental MeasurementsAs reported previously by Li Yi et al.[5],a wool fabric sample measuring 3cm615cm62.96mm was suspended in a cell from an electronic balance,which can measure the weight change of fabric during the sorption process.In the cell the temperature was controlled at293.12Æ2K,and the atmospheric pressure was controlled at1atm.The relative humidity was produced by aflow-divider humidity 256L.FENGZHI ET AL.generator,which can change the relative humidity (RH)from 0%to 99%in 1%steps to an accuracy of Æ0.1%of total flow.Fabric was equlibriated in the cell at 0%RH for 90min,then the RH in the cell was rapidly changed from 0%to 99%at time zero,and this RH was maintained for 90min.The surface temperature of the specimen was measured by using a fine thermocouple wire attached to fabric surface.The mean water content of the fabric was calculated on the basis of the weight recorded by a balance.The detailed information on experimental measurements and uncertainty analysis for experimental data was reported previously [5].Table 1.Parameter values for computation ParameterSymbol Unit Value and referenceDensity of a fiber r f kg =m 31,300[14]Volumetric heat C vf kJ =m 3K 373.3þ4661W c þ4.221T [14]capacity of fabric Thermal conductivity of fabricK fab W =m 2K (38.49370.72w c þ0.113w c 270.002w c 3)61073[14]Head of sorption of water vapor by fibers l kJ =kg 1602.5exp(711.72w c )þ2522.0[14]Diffusion coefficientof water vapor in fiberD f ðw c ;t Þm 2=s(1.04þ62.8w c 71342.59w c 2)610714t <540s [14]1:6164f 1Àexp ½À18:16exp ðÀ28:0w c Þ g t !540s [14]Dynamic viscosity of gasm g kg =m s 1.8361075[15]Dynamic viscosity of liquid waterm w kg =m s 1.061073[15]Intrinsic permeability of fabricKm 21.5610713[16]Figure parison of the temperature between theoretical predictions and experimental measurements.SIMULATION OF COUPLED HEAT AND MASS TRANSFER257The new model described in Section2is applied to this experimental condition by specifying corresponding boundary conditions.The comparisons of temperature and bound water content between theoretical prediction and experimental mea-surements[10]are shown in Figures3and4,respectively.Figure3shows tem-perature changes at the surface of the fabric during the dynamic-moisture diffusion process.Since the temperature of surroundings was kept constant at293.15K,in the testing conditions,no external heatflow was provided to the fabric.Hence the temperature rise in the fabric was due purely to the heat released during the moisture-sorption process.The mean error between theoretical prediction and experimental measurements on temperature is0.13%.Figure4shows the mean moisture uptake of the fabric during humidity transient.The mean error on water content of fabric is4.6%.The diameter of thefiber significantly affects its sorption rate.The difference between measured and actual diameter of thefiber and porosity of fabric may be the main sources of paring the predicted temperature and mean moisture uptake with the experimental results,it is obvious that the models are able to predict the moisture sorption of thefibers with satisfactory accuracy.4.2.Influence of Atmospheric Pressure GradientIn order to investigate the effect of the pressure gradient on heat and mass transfer in porous materials,we carried out a series of computational experiments; two cases are selected to show the trends.In the computations,the fabric sample is assumed to be made of woolfiber with thickness L¼3.0mm,and the initial and boundary conditions were specified as follows.The initial conditions wereT0¼298:15K r v0¼0:01kg=m3S0¼0p g0¼1:0135e5PaAt the left (x ¼0)boundary,T 1¼298:15K r v 1¼0:02kg =m 3m w j G ¼0p g G ¼1:0135e 5PaAnd at the right (x ¼L)boundary,T 1¼298:15K ;r v 1¼0:02kg =m 3S ¼0:4p g G ¼2:0135e 5Pa in Case 11:0135e 5Pa in Case 2(The difference between case 1and case 2is the pressure boundary condition at the location x ¼L .Other conditions are the same.The corresponding numerical simu-lations and comparisons are shown in Figures 5–9.Figure 5a shows the atmospheric pressure variation in the fabric with time under the pressure boundary condition of case 1.We can see that the atmospheric pressure is uniform constant initially,then the atmospheric pressure redistributes with the new boundary pressures.The pressure at x ¼L reaches 2atm.Figure 5bFigure 5.Distribution of atmosphericpressure.Figure 6.Distribution of water-vapor concentration.SIMULATION OF COUPLED HEAT AND MASS TRANSFER 259represents the pressure distribution under the condition of case 2.The pressure is uniform because of the same initial and boundary conditions.Figure 6a shows the distribution of the water-vapor concentration in case 1:the water vapor concentration at x ¼0is higher than that at x ¼L .This is because of the effect of atmospheric pressure.The atmospheric pressure gradient,which is the main driving force of water-vapor movement,makes the water vapor diffuse from high pressure (location x ¼L )to low pressure (location x ¼0).Figure 6b shows dis-tribution of the water-vapor concentration in case 2.The water vapor diffuses due to the driving force of water vapor partial pressure gradient only,when the total pressure gradient does not exist.Because of the water-vapor diffusion,the water-vapor concentration in the fabric increases from 0.01to 0.02kg =m 3with time.Then,the concentration of the water vapor reaches equilibrium.Figure 7shows the distribution of water content in fiber.Since the hygro-scopicity of wool is high,water vapor is absorbed.The different water vapor con-centration affects the relative humidity of the water vapor,and then affects the amount of water absorbed by the fiber.From Figures 7a and 7b we can see that the distributions of water content in the fiber agree with the water-vapor concentration in Figures 6a and 6b ,respectively.Figure 7.Water content distribution offiber.Figure8shows the predicted temperature distribution in the wool fabric during the vapor diffusion process.From Figure8,we can see that the temperature rises in the middle layer of the fabric because of the heat released during moisture sorption. At the beginning,the temperature rises quickly,due to the large sorption rate of fiber.Then the temperature decreases gradually,with time reaching equilibrium with the environmental temperature because of much lower sorption rate and heat exchange with environment.At the beginning,the temperature in case1is lower than that in case2.The reason is that the atmosphere pressure gradient affects the dis-tribution of the water-vapor concentration.The different water-vapor concentration affects the amount of water absorbed by thefiber,which leads to different sorption heat.Most of the water content of thefibers in the fabric,except at the boundary x¼L in case1,is lower than that in case2(see Figure7),so the temperature in case1 is lower than that in case2.From Figures8a and8b we also can see the temperature at location x¼L is larger than that at location x¼0in thefirst stage.This is because there is much more liquid water at location x¼L than that at x¼0,and the conductivity of liquid water is greater than that of fabric.Figure9shows the distribution of liquid water saturation during the process of liquid water diffusion into the wool fabric by capillary action.We can see that the liquid water diffuses from side x¼L to x¼0.The reason is that the diffusion potential of the liquid water is liquid water pressure,which equals the difference between atmospheric pressure and capillary pressure.The capillary pressure in the region of high saturation of liquid water(x¼L)is lower than that in the region of low saturation of liquid water(x¼0).In case1,the atmospheric pressure at x¼L is higher than that at x¼0.And in case2,the atmospheric pressure at x¼0is equal to that at x¼L.So the total liquid water driving potential at location x¼L is higher than that at location x¼0in both case1and case2.From Figures9a and9b we can see that the difference in the distributions of liquid water saturation between case1 and case2is not large in this computational condition.5.CONCLUSIONIn this article,we report a new model of heat and moisture transfer inhygroscopic porous textile materials,considering the influence of the atmospheric。

Lecture 2: Numerical Methods for Differentiations and IntegrationsAs we have discussed in Lecture 1 that numerical simulation is a set of carefully planed numerical schemes to solve initial value problems numerically. Let us consider the following three types of differential equations.dy (t )dt=f (t ) (2.1) dy (t )dt=f (y ,t )(2.2) ∂y (x ,t )∂t =f (t ,y ,∂y ∂x ,∂2y∂x2,...,ydx ,...∫)(2.3)The numerical methods for time integration of these equations will be discussed in the next section (Section 4). Before we conduct the time integration, we need to determine thedifferentiations∂y ∂x ,∂2y∂x 2,... and integrations ydx ,...∫ on the left hand side of the equation(2.3) at each grid point.In this section, we are going to discuss the following four types of numerical methods, which are commonly used in spatial differentiations and integrations.1. Finite Differences (based on Taylor’s expansion)2. FFT (Fast Fourier Transform)3. Cubic Spline2.1. Finite DifferencesFor convenience, we shall use the following notation in the rest of this lecture notes.f ijk n =f (x =i Δx ,y =j Δy ,z =k Δz ,t =n Δt )=f (x i ,y j ,z k ,t n )Given a tabulate functioni :12...N x i :x 1x 2...x N f i :f 1f 2...f NUsing finite difference method, we can obtain derivatives of a tabulated function f . Table 2.1 lists examples of the first-order and second-order finite-difference expressions of[d f /dx ]x =x i , [d 2f /dx 2]x =x i , and [d 3f /dx 3]x =x i . Table 2.2 lists examples of the finite∫,difference expressions of y=f dxTable 2.1. The numerical differentiations based on finite difference methodExercise 2.1.(a) Show that the Central Difference shown in Table 2.1 is a second-order scheme(b) Show that the Forward Difference and the backward difference shown in Table 2.1 arefirst-order schemes., and(c) Determine the forth order central difference expressions of [d f/dx]x=x i, based on the Taylor expansions of the function f.[d2f/dx2]x=x iExercise e the first-order, the second-order, and the forth-order finite-differences expressions to determine the d f/dx, and d2f/dx2, an analytical function f with a fixed Δx. Determine the numerical errors in your results. Compare the numerical errors obtained from different finite differences expressions.Figure 2.1. A summary diagram of the central difference, forward difference, and the backward difference schemes.Table 2.2. The spatial integrations based on finite difference methodExercise 2.3.Use the first order, the second order, and the forth order integration expressions listed in Table 2.2 to determine y (x =π/4) with dy (x )/dx =cos(x ) and boundary conditiony (x =0)=0. Determine the numerical errors in your results. Compare the numericalerrors obtained from different integration expressions.2.2. FFT (Fast Fourier Transform)A function can be expanded by a complete set of sine and cosine functions. In the Fast Fourier Transform, the sine and cosine tables are calculated in advance to save the CPU time of the simulation.For a periodic function f , one can use FFT to determine its differentiations and integrations, i.e.,d fdx=FFT −1{ik [FFT (f )]} fdx ∫=FFT −1{1ik [FFT (f )]} for k >0.Exercise 2.4.Use an FFT subroutine to determine the first derivatives of a periodic analytical functionf . Determine the numerical errors in your results.Exercise 2.5.Use an FFT subroutine to determine the first derivatives of a non-periodic analytical function f . Determine the numerical errors in your results.2.3. Cubic SplineA tabulate function can be fitted by a set of piece-wise continuous functions, in which the first and the second derivatives of the fitting functions are continuous at each grid point. One need to solve a tri-diagonal matrix to determine the piece-wise continuous cubic spline functions. The inversion of the tri-diagonal matrix depends only on the position of grid points. Thus, for simulations with fixed grid points, one can evaluate the inversion of the tri-diagonal matrix in advance to save the CPU time of the simulation.For a non-periodic function f, it is good to use the cubic spline method to determine its differentiations and integrations at each grid point.Results of differentiations obtained from the cubic spline show the same order of accuracy as the results obtained from the forth order finite differences scheme.Exercise 2.6.Use a Cubic Spline subroutine to determine the first derivatives of an analytical functionf. Determine the numerical errors in your results.ReferencesHildebrand, F. B., Advanced Calculus for Applications, 2nd edition,Prentice-Hall, Inc., Englewood, Cliffs, New Jersey, 1976.Press, W. H., B. P. Flannery, S. A. Teukolsky, and W. T. Vetterling, Numerical Recipes (in C or in FORTRAN and Pascal), Cambridg e University Press, Cambridge, 1988.System/360 Scientific Subroutine Package Version III, Programmer’s Manual,5th edition, IBM, New York, 1970.。
![气科院大气物理面试英语专业词汇[1]](https://img.taocdn.com/s1/m/dc738b36dcccda38376baf1ffc4ffe473368fdf6.png)
大气科学系微机应用基础Primer of microcomputer applicationFORTRAN77程序设计FORTRAN77 Program Design大气科学概论An Introduction to Atmospheric Science大气探测学基础Atmospheric Sounding流体力学Fluid Dynamics天气学Synoptic Meteorology天气分析预报实验Forecast and Synoptic analysis生产实习Daily weather forecasting现代气候学基础An introduction to modern climatology卫星气象学Satellite meteorologyC语言程序设计 C Programming大气探测实验Experiment on Atmospheric Detective Technique云雾物理学Physics of Clouds and fogs动力气象学Dynamic Meteorology计算方法Calculation Method诊断分析Diagnostic Analysis中尺度气象学Meso-Microscale Synoptic Meteorology边界层气象学Boundary Layer Meteorology雷达气象学Radar Meteorology数值天气预报Numerical Weather Prediction气象统计预报Meteorological Statical Prediction大气科学中的数学方法Mathematical Methods in Atmospheric Sciences专题讲座Seminar专业英语English for Meteorological Field of Study计算机图形基础Basic of computer graphics气象业务自动化Automatic Weather Service空气污染预测与防治Prediction and Control for Air Pollution现代大气探测Advanced Atmospheric Sounding数字电子技术基础Basic of Digital Electronic Techniqul大气遥感Remote Sensing of Atmosphere模拟电子技术基础Analog Electron Technical Base大气化学Atmospheric Chemistry航空气象学Areameteorology计算机程序设计Computer Program Design数值预报模式与数值模拟Numerical Model and Numerical Simulation接口技术在大气科学中的应用Technology of Interface in Atmosphere Sciences Application海洋气象学Oceanic Meteorology现代实时天气预报技术(MICAPS系统)Advanced Short-range Weather Forecasting Technique(MICAPS system)1) atmospheric precipitation大气降水2) atmosphere science大气科学3) atmosphere大气1.The monitoring and study of atmosphere characteristics in near space as an environment forspace weapon equipments and system have been regarded more important for battle support.随着临近空间飞行器的不断发展和运用,作为武器装备和系统环境的临近空间大气特性成为作战保障的重要条件。