下载文档原格式
自适应控制什么是自适应控制自适应控制是一种控制系统设计方法，它通过实时监测和调整系统的参数来适应不确定的外部环境和内部系统变化。
自适应控制可以提高控制系统的性能和鲁棒性，使其能够快速、准确地响应不断变化的环境或系统参数。
在传统的控制系统中，通常假设系统的数学模型是已知和固定的。
然而，在实际应用中，系统的动态特性常常受到各种因素的影响，如外部扰动、参数变化、非线性效应等。
这些因素使得传统的控制方法往往无法满足系统的控制要求。
而自适应控制则能够通过不断地观测和在线调整系统参数，使系统能够适应这些变化，并实现良好的控制效果。
自适应控制的基本原理自适应控制的基本原理是根据系统的实时反馈信息来调整控制器的参数。
具体来说，自适应控制系统通常由以下几个部分组成：1.参考模型：参考模型是指描述所期望控制系统输出的理想模型，通常由一组差分方程来表示。
参考模型的作用是指导控制系统的输出，使其能够尽可能接近参考模型的输出。
2.系统模型：系统模型是指描述被控对象的数学模型，包括其输入、输出和动态特性。
系统模型是自适应控制的重要基础，它确定了控制系统需要调整的参数和控制策略。
3.控制器：控制器是自适应控制系统的核心部分，它根据系统输出和参考模型的误差来实时调整控制器的参数。
控制器可以通过不同的算法来实现，如模型参考自适应控制算法、最小二乘自适应控制算法等。
4.参数估计器：参数估计器是自适应控制系统的关键组件，它用于估计系统模型中的未知参数。
参数估计器可以通过不断地观测系统的输入和输出数据来更新参数估计值，从而实现对系统参数的实时估计和调整。
5.反馈环路：反馈环路是指通过测量系统输出并将其与参考模型的输出进行比较，从而产生误差信号并输入到控制器中进行处理。
反馈环路可以帮助控制系统实时调整控制器的参数，使系统能够适应外部环境和内部变化。
自适应控制的应用领域自适应控制在各个领域都有广泛的应用，特别是在复杂和变化的系统中，其优势更为突出。
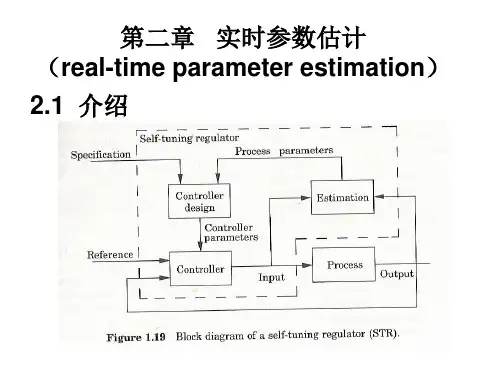
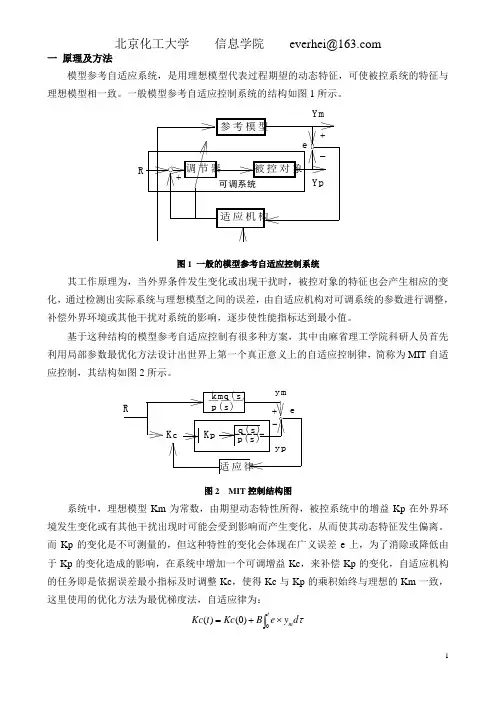
一 原理及方法模型参考自适应系统，是用理想模型代表过程期望的动态特征，可使被控系统的特征与理想模型相一致。
一般模型参考自适应控制系统的结构如图1所示。
图1 一般的模型参考自适应控制系统其工作原理为，当外界条件发生变化或出现干扰时，被控对象的特征也会产生相应的变化，通过检测出实际系统与理想模型之间的误差，由自适应机构对可调系统的参数进行调整，补偿外界环境或其他干扰对系统的影响，逐步使性能指标达到最小值。
基于这种结构的模型参考自适应控制有很多种方案，其中由麻省理工学院科研人员首先利用局部参数最优化方法设计出世界上第一个真正意义上的自适应控制律，简称为MIT 自适应控制，其结构如图2所示。
图2 MIT 控制结构图系统中，理想模型Km 为常数，由期望动态特性所得，被控系统中的增益Kp 在外界环境发生变化或有其他干扰出现时可能会受到影响而产生变化，从而使其动态特征发生偏离。
而Kp 的变化是不可测量的，但这种特性的变化会体现在广义误差e 上，为了消除或降低由于Kp 的变化造成的影响，在系统中增加一个可调增益Kc ，来补偿Kp 的变化，自适应机构的任务即是依据误差最小指标及时调整Kc ，使得Kc 与Kp 的乘积始终与理想的Km 一致，这里使用的优化方法为最优梯度法，自适应律为：⎰⨯+=tm d y e B Kc t Kc 0)0()(τYp Yme+__+R参考模型调节器被控对象适应机构可调系统———kmq(s)p(s)KcKpq(s)-----p(s)适应律Rymype+-MIT 方法的优点在于理论简单，实施方便，动态过程总偏差小，偏差消除的速率快，而且用模拟元件就可以实现；缺点是不能保证过程的稳定性，换言之，被控对象可能会发散。
二 对象及参考模型该实验中我们使用的对象为：122)()()(2++==s s s p s q K s G pp 参考模型为：121)()()(2++==s s s p s q K s G mm 用局部参数最优化方法设计一个模型参考自适应系统，设可调增益的初值Kc(0)=0.2，给定值r(t)为单位阶跃信号，即r(t)=A ×1(t)。
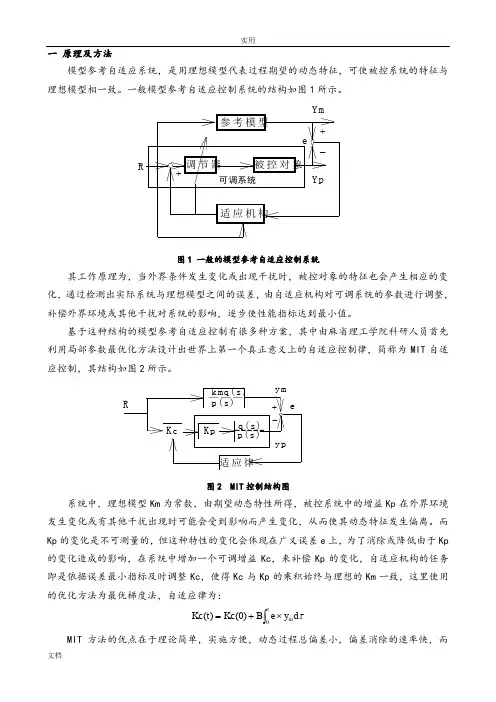
一 原理及方法模型参考自适应系统，是用理想模型代表过程期望的动态特征，可使被控系统的特征与理想模型相一致。
一般模型参考自适应控制系统的结构如图1所示。
图1 一般的模型参考自适应控制系统其工作原理为，当外界条件发生变化或出现干扰时，被控对象的特征也会产生相应的变化，通过检测出实际系统与理想模型之间的误差，由自适应机构对可调系统的参数进行调整，补偿外界环境或其他干扰对系统的影响，逐步使性能指标达到最小值。
基于这种结构的模型参考自适应控制有很多种方案，其中由麻省理工学院科研人员首先利用局部参数最优化方法设计出世界上第一个真正意义上的自适应控制律，简称为MIT 自适应控制，其结构如图2所示。
图2 MIT 控制结构图系统中，理想模型Km 为常数，由期望动态特性所得，被控系统中的增益Kp 在外界环境发生变化或有其他干扰出现时可能会受到影响而产生变化，从而使其动态特征发生偏离。
而Kp 的变化是不可测量的，但这种特性的变化会体现在广义误差e 上，为了消除或降低由于Kp 的变化造成的影响，在系统中增加一个可调增益Kc ，来补偿Kp 的变化，自适应机构的任务即是依据误差最小指标及时调整Kc ，使得Kc 与Kp 的乘积始终与理想的Km 一致，这里使用的优化方法为最优梯度法，自适应律为：⎰⨯+=tm d y e B Kc t Kc 0)0()(τMIT 方法的优点在于理论简单，实施方便，动态过程总偏差小，偏差消除的速率快，而Yp Yme+__+R参考模型调节器被控对象适应机构可调系统———kmq(s)p(s)KcKpq(s)-----p(s)适应律Rymype+-且用模拟元件就可以实现；缺点是不能保证过程的稳定性，换言之，被控对象可能会发散。
二 对象及参考模型该实验中我们使用的对象为：122)()()(2++==s s s p s q K s G pp 参考模型为：121)()()(2++==s s s p s q K s G mm 用局部参数最优化方法设计一个模型参考自适应系统，设可调增益的初值Kc(0)=0.2，给定值r(t)为单位阶跃信号，即r(t)=A ×1(t)。
