北邮高等数学英文版课件Lecture 10-2
- 格式:pptx
- 大小:2.07 MB
- 文档页数:46


高等数学北邮第2版介绍《高等数学北邮第2版》是由北京邮电大学出版社出版的教材,主要面向高等院校的理工科专业学生。
本教材是高等数学领域的经典教材之一,经过多年的使用和改进,逐渐发展成为一本综合性的高等数学教材。
本教材分为多个部分,涵盖了高等数学的各个方面,包括数列与极限、函数与连续、导数与微分、定积分与定积分应用、常微分方程等内容。
每个章节都以理论知识和例题相结合的形式,旨在帮助学生深入理解高等数学的概念和原理,并培养学生的数学思维和解题能力。
目录1.数列与极限2.函数与连续3.导数与微分4.定积分与定积分应用5.常微分方程6.二重积分与曲线积分7.空间解析几何数列与极限在数学中,数列是将自然数映射到数域的一个函数。
数列的极限是数列中接近某一特定值的值。
数列与极限是高等数学中的重要概念,它们贯穿了高等数学的各个领域。
本章介绍了数列的基本概念和性质,包括数列的定义、极限的定义和性质、极限存在准则等等。
通过学习本章,学生将掌握数列的极限计算方法,并能够应用数列的极限解决实际问题。
函数与连续函数是数学中的一个基本概念,它描述了两个数集之间的对应关系。
连续是函数在某一点处没有跳跃或间断的特性,连续性是函数中的一个重要概念。
本章介绍了函数的基本概念和性质,包括函数的定义、函数的运算、反函数与复合函数等。
同时,本章还介绍了连续函数的定义和性质,并通过例题讲解了如何判断函数的连续性以及如何应用连续函数解决实际问题。
导数与微分导数是函数在某一点处的变化率,微分是函数在某一点处的近似变化量。
导数与微分是高等数学中的核心概念,它们在数学和物理等领域具有广泛的应用。
本章介绍了导数的定义和性质,包括导数的计算公式、导数的几何意义、高阶导数等。
同时,本章还介绍了微分的定义和性质,包括微分的近似计算、微分中值定理等。
通过学习本章,学生将掌握导数和微分的计算方法,并能够应用导数和微分解决实际问题。
定积分与定积分应用定积分是计算曲线下面积的数学工具,定积分应用是将定积分应用于实际问题的过程。

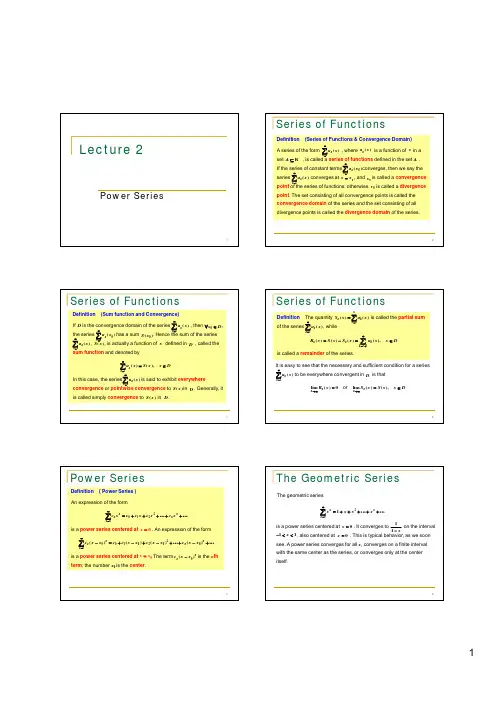







英语讲解高等数学教材Mathematics is often regarded as a challenging subject for many students, especially at the advanced level. To assist students in grasping the concepts more effectively, providing English explanations alongside the high-level mathematics textbook can greatly enhance understanding. This article aims to highlight the benefits of incorporating English explanations into the teaching of high-level mathematics.1. Enhancing ComprehensionEnglish explanations in high-level mathematics textbooks can help students comprehend complex concepts more easily. By presenting the material in a different language, students are encouraged to think and analyze the content from a fresh perspective. Additionally, English explanations can provide alternative explanations and examples, ensuring that students have a well-rounded understanding of the topic.2. Improving Language ProficiencyIntegrating English explanations into high-level mathematics textbooks also helps students improve their English language proficiency. As they engage with mathematical concepts in English, students are exposed to academic vocabulary and sentence structures commonly used in technical subjects. This exposure enhances their language skills and prepares them for future academic pursuits.3. Bridging the Language GapHigh-level mathematics often involves sophisticated vocabulary and specialized terminology. Some students may find it challenging to understand these terms solely in their native language. By providing English explanations, the language gap is bridged, enabling students to grasp the meaning of technical terms more accurately. This ensures that no language barrier impedes students' understanding and learning progress.4. Facilitating International StudyWith the increasing globalization of education, many students aspire to pursue higher studies abroad. English explanations in high-level mathematics textbooks prepare students for this international exposure. By familiarizing themselves with technical vocabulary in English, students gain confidence in their ability to study mathematics in an English-speaking environment.5. Encouraging Deeper ReflectionEnglish explanations encourage students to think critically and deeply about mathematical theories and concepts. When students are exposed to different explanations and perspectives in English, they are encouraged to engage in analytical thinking, compare different approaches, and develop a more comprehensive understanding of the topic at hand.In conclusion, incorporating English explanations into high-level mathematics textbooks brings numerous benefits to both comprehension and language proficiency. By bridging the language gap, students are able to fully grasp complex concepts, regardless of their language background. Moreover, this integration prepares students for future academic pursuits and international study. Ultimately, English explanations enhance students'understanding and enjoyment of high-level mathematics, paving the way for success in this challenging subject.。
形式系统由{∑, TERM, FORMULA, AXIOM, RULE}等5个部分构成,其中 称为符号表,TERM 为项集;FORUMULA 为公式集;AIXIOM 为公理集;RULE 为推理规则集。
:1、 符号表∑为非空集合,其元素称为符号。
2、 设∑*为∑上全体字的组合构成的集合。
项集TERM 为∑*的子集,其元素称为项;项集TERM 有子集V ARIABLE 称为变量集合,其元素称为变量。
F(X) a, X,3、 设∑*为∑上全体字的组合构成的集合。
公式结FORMULA 为∑*的子集,其元素称为公式;公式集有子集ATOM ,其元素称为原子公式。
A(f(a,x1,y)), A →B4、 公理集AXIOM 是公式集FROMULA 的子集,其元素称为公理。
5、 推理规则集RULE 是公式集FORMULA 上的n 元关系集合,即RULE=)}2(|{n FORMULA r n n n r ⊆∧≥∧∃是正整数,其元素称为形式系统的推理规则。
其中公式集和项集之间没有交叉,即TERM ∩FORMULA =φ,公式和项统称为表达式。
由定义可知,符号表∑、项集TERM 、公式集FORMULA 是形式系统的语言部分。
而公理集AXIOM 、推理规则集RULE 为推理部分形式系统特性1、 符号表∑为非空、可数集合(有穷、无穷都可以)。
2、 项集TERM 、公式集FORMULA 、公理集AXIOM 和推理规则集RULE 是递归集合,即必须存在一个算法能够判定给定符号串是否属于集合(可判定的)。
3、 形式系统中的项是用来描述研究的对象,或者称为客观世界的。
而公式是用来描述这些研究对象的性质的。
这个语言被称为对象语言。
定义公式和项产生方法的规则称为词法。
公理:I))((A B A →→ II))()(())(((C A B A C B A →→→→→→ III ))())()(((A B B A →→⌝→⌝证明:A →A(1)A →(A →A)((A →(B →C))→((A →B)→(A →C))((A →(B →A))→((A →B)→(A →A))((A →((A →A)→A))→((A →(A →A))→(A →A))A →((A →A)→A))(A →(A →A))→(A →A)(A →(A →A))A →ABB A A →, 已知:R 是一个有关公式的性质证明:R 对于所有公式有效I. 对于)(FSPC Atom p ∈,则)(P RII. 假设公式A 和B 都具有RIII. )(FSPC Atom A ∈∀,且)(A R ,则)(A R ⌝IV. B A ,∀是公式,如果)(A R 且)(B R ,则)(B A R →根据:形式系统的联结词只有两个→⌝,,因为在命题逻辑的语义上,其他联结词可以有这两个联结词表示。
北京邮电大学高等数学教材英文版Beijing University of Posts and Telecommunications Advanced Mathematics Textbook (English Version)IntroductionThe Beijing University of Posts and Telecommunications (BUPT) is renowned for its comprehensive and cutting-edge curriculum. As part of its commitment to providing a world-class education, BUPT offers an English version of its advanced mathematics textbook. This article will delve into the content, structure, and importance of the BUPT Advanced Mathematics Textbook (English Version).Content OverviewThe BUPT Advanced Mathematics Textbook covers a wide range of topics, including calculus, linear algebra, differential equations, and probability theory. Each chapter provides a comprehensive introduction to the topic, followed by detailed explanations and examples that illustrate the concepts. The textbook also includes exercises and practice problems to reinforce understanding and improve problem-solving skills.Structure of the TextbookThe textbook consists of several sections, each specifically designed to enhance comprehension and facilitate learning. Here is an overview of the main sections:1. Introduction: This section provides a brief overview of the topic, its relevance, and its applications in various fields.2. Theory and Concepts: In this section, the fundamental theory and concepts related to the topic are presented in a clear and concise manner. Definitions, theorems, and formulas are introduced, followed by in-depth explanations.3. Examples and Illustrations: To further enhance understanding, the textbook includes numerous examples and illustrations that demonstrate the application of the theory and concepts explained in the previous section. These examples provide real-life scenarios where the mathematical principles can be applied.4. Exercises and Problem Solving: A vital component of the textbook, this section offers a wide range of exercises and problems to test the reader's comprehension and analytical skills. Solutions and step-by-step explanations are provided to assist students in the learning process.Importance of the TextbookThe English version of the BUPT Advanced Mathematics Textbook serves a crucial role in promoting international education and collaborations. It enables international students to access and comprehend BUPT's advanced mathematics curriculum, fostering cross-cultural exchange and learning. Moreover, the textbook contributes to the overall improvement of mathematics education by providing a comprehensive resource for both students and instructors.ConclusionThe BUPT Advanced Mathematics Textbook (English Version) plays a significant role in Beijing University of Posts and Telecommunications'commitment to academic excellence and internationalization. With its comprehensive content, well-structured format, and emphasis on application and problem-solving, the textbook equips students with the necessary knowledge and skills to excel in advanced mathematics. It serves as a valuable resource for both domestic and international students, further reinforcing BUPT's reputation as a leading institution in mathematics education.。