换元积分法PPT课件

格式：ppt
大小：957.00 KB
文档页数：10

下载文档原格式

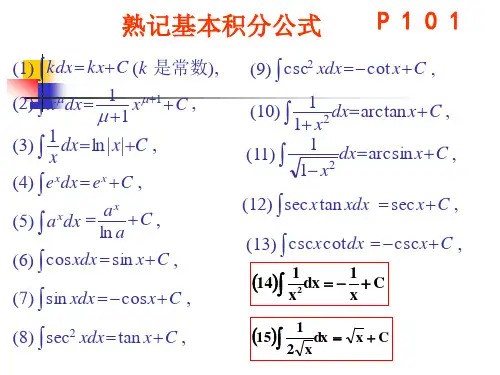
4.2_换元积分法

x x
dx 3
t2
t
3
2tdt
2
t2 3 dt 2 t3 6t C 3
再将t x 3代回整理得
x dx 2 x3 3
3
x3 6 x3C
补充例：求
1 dx
ex 1
解: 令 ex 1 t 则x ln(1 t 2 )
dx
2t 1 t2
dt , 于是
1 dx
ex 1
Fu C
Fx C
由此可得换元法定理P103定理4.3
P103定理4.3 设 f (u)具有原函数，u ( x)可导，
则有换元公式
f [ ( x)] ( x)dx [ f (u)du]u ( x)
第一类换元公式（凑微分法）说明使用此公式的关键在于将
g( x)dx 化为 f [( x)]( x)dx.
2
2
xex2dx 1 ex2 x2 dx（直接凑微分） 2
1 ex2dx 2
2
1 2
eudu
堂上练习 P108-习题4.2----4、5、6、
4、
2x 1 x2 dx
1 1 x2
1 x2
dx
1
1 x2
d1
x
2
ln
1
x
2
C
5、 x x2 5dx 1 2
x2
1 t
2t 1 t2
dt
2
1 1 t 2 dt
2arctant C
2arctan ex 1 C
课堂练习：求
x 1dx . x
解 : 令 x 1 t,则x 1 t 2 , dx 2tdt;于是有
x-1 dx. 2 x
t2 1 t 2 dt

换元积分法

1 4
(
2x 3
2x 1)dx
1 4
2x
3dx
1 4
2x 1dx
1 8
2
x
3d(2
x
3)
1 8
2x 1d(2x 1)
1
31
3
2x 3 2x 1 C.
12
12
14
例12. 求 tan3 x dx tan2 x tan x dx
(sec2 x 1)tan x dx
a2 x2
a
18
例17. 求
解:
1 1 x2 a2 2a
(x a) (x a) ( x a)( x a)
1( 1 2a x a
1) xa
∴
原式
=
1 2a
dx xa
dx
x
a
1 2a
d( x a) xa
d( x a) xa
1 ln
2a
xa
ln
xa
C 1 ln 2a
xa xa
)
1 2
1
1 x
2
d(1
x2
)
u 1 x2
1 2
1du u
1 ln u C 2
1 ln(1 x2 ) C . 2
例2 x 1 x2dx 1 1 x2d(-x2 ) 2
1 2
1 x2d(1 x2 ) u 1 x2 1
2
udu
1
2
3
u2
C
1
3
u2
C
1
(1
x
2
)
3 2
29
小结
1、常用的几种凑微分形式:
1
(1) f (ax b)dx a

经济数学44换元积分法

2 1 a [a 1 x d ( a x )a 1 x d ( a x ) ]
2 1 aln axln axC
21alnaaxx C．类似可得
x2 1a2dx21alnx x a aC．
ESC
一、第一换元积分法
例15 求 secxdx ．解 sexcdx c1oxsdx
例2
求
1 x2
1
ex
dx.
e 被积函数是两个因子:
1 x
和
1 x2
的乘积
解
因
(1x)

1 x2
,
视
于是被积函数具有形式
(x)
1, x
可用换元积分法
x12e1x e1x(x12)f((x))(x)
本例可不设出中间变量
u

1 x
,按如下格式书写:
1 x2
1 212 1lnxd(12lnx)
1 2lnlnxC
ESC
一、第一换元积分法
例13 co1 s2x(tanx1)dx
•解法一
co1 s2x(tanx1)dx (tanx1)d(tanx1)(tanx21)2C
•解法二
co1 s2x(tanx1)dx (tanx1)d(tanx)tan22xtanxC
x2x4dx1 2x21 42xdx12 x214d(x24)
于是 1 0 x2
x
4
dx

12ln(x24)
1 0
1 2lnx(24)C,
1 2[l1 n 2(4)ln02(4)
由牛顿—莱
]布尼茨公式
12(ln52ln2).
ESC
一、第一换元积分法

定积分的换元法和分部积分法课件

常数倍性质
定积分具有常数倍性质，即对于任意非零常数c，有c乘以被积函数的定积分等于该常数乘以被积函数在积分区间上的增量。
定积分的计算
直接法
直接代入被积函数进行计算，适用于简单的被积函数和明确的积
分区间。
换元法
通过变量替换简化被积函数或积分区间，适用于较为复杂的积分
问题。
分部积分法
通过将两个函数的乘积进行分部积分，将一个复杂函数的积分转化为更简单函数的积分，适用于
计算旋转体的体积
01
定积分可以用于计算旋转体的体积，例如旋转抛物面下的体积
。
求解平面图形的面积
02
定积分可以用于求解平面图形的面积，例如椭圆、圆、三角形
等。
求解曲线长度
03
定积分可以用于求解曲线的长度，例如圆的周长、正弦函数的
长度等。
05
定积分的应用
定积分在物理中的应用
计算物体在恒力作用下的运动轨迹
分部积分法在求解三角函数的不定积分中有着广泛的应用，例如求解$int sin x dx$或$int cos x dx$等。
求解复杂函数的不定积分
对于一些复杂函数的不定积分，分部积分法可以将其转化为简单函数的定积分，从而简化计算过程。例如求解$int x^2 e^x dx$等。
04
定积分的几何意义
03
分部积分法在定积分中的应用
分部积分法的定义和原理
分部积分法的定义
分部积分法是一种求解定积分的技巧，通过将一个不定积分转化为两个函数的乘积的导数，从而简化计算过程。
分部积分法的原理
基于微积分基本定理，通过将一个复杂函数的不定积分转化为简单函数的定积分，实现积分的求解。

高数课件-定积分的换元积分法与分部积分法

0 ( 1
sin t
t
dt )d(
x2 2
)
[ x2
2
x 1
2
sin t
t
dt
]10
1 0
x2 2
sin x2 x2
2 xdx
0
1
0
x
sin
x
2dx
1 (cos1 1) 2
1
例13 设f (t)连续, f (1) 0 , 解
1
例14 證明
n1n331 ,
n n2 4 2 2
n 為偶數
当 x 0 时, t 0; x a 时, t
2
∴
原式 = a 2
2 cos2 t d t
0
a2 2
2 0
(1
cos
2
t)d
t
y
y
a2 x2
a2
4
o
ax
1
例2 求 0a
1
dx
(x2 a2)3
(a 0)
解令x a tant, dx a sec2 t d t
当 x 0 时, t 0; x a 时, t
t dt 1
1 t2
2
1
12(1
t
2
)
1 2
d (1 t 2 )
3
12 1t2 2
1 2
1 3
2 2 3
3 2
1
例4
1 x2 1
1
x4
dx 1
1 x2
1
2x 1 x4 1
2x dx
1
1 x2
1
dx
2x 1
1 1
x
2x 4 1

§3.3定积分换元法

π 2
0
sin n xdx = − ∫
π 2
0
sin n −1 xd (cos x )
π 2 0
= − sin n −1 x cos x
[
= (n − 1) ∫
π 2 0 π 2
]
π 2 0
+∫
cos xd (sin n −1 x )
cos 2 x sin n − 2 xdx
= (n − 1) ∫
0
8．已知 g ( x ) = ∫ t f ′( x − t )dt ，求 g′( x ) 。
0
x
g( x ) = ∫ t f ′( x − t )dt
0
x 0
x
令x−t=u
=
− ∫ ( x − u ) f ′(u )du
x
0
= ∫ ( x − u ) f ′(u )du = x
x
∫0 f ′(u )du − ∫0 uf ′(u )du
a a ∫ 0 f(− x) dx
0
f(x) dx =
+
a ∫0
f(x) dx = ∫ [ f(x) + f(− x)] dx.
0
a
续上
∴∫
a
−a
f(x) dx = ∫ [f(x) + f( − x)] dx ，
0
a
（2）∵ f ( x ) 为偶函数，即 f (− x ) = f ( x ) ，
∴∫
π 2 sin 2 t − 1 dt π sin t 6
6 cos t dt = π cos t sin t 2
∫
6 cos t dt π cos t ⋅ sin t 2

《换元积分法》课件

确定新变量
在原积分中，选择一个易于积分的变量替换原积分中的变量，以简化积分过程。
选择新变量原则
选择的新变量应使得积分过程更简单、直观，便于计算。
常见新变量选择
对于形如$int f(x) dx$的积分，常见的新变量选择有$t = g(x)$或$x = g(t)$，其中 $g(x)$是原函数$f(x)$的可导函数。
要点二
计算简便
通过换元，可以将复杂积分转化为简单积分，降低计算难度。
换元积分法的优缺点
• 易于理解：换元积分法的原理相对直观，易于理解。
换元积分法的优缺点
需要选择合适的换元
选择合适的换元是关键，如果选择不当，可能导致计算过程复杂化。
对初学者有一定难度
换元积分法涉及到变量替换，对初学者来说可能有一定的学习难度。
新积分计算的注意事项
在计算新积分的过程中，需要注意积分的上下限是否发生变化，以及积分的计算是否正确。
04
换元积分法的实例
实例一：计算定积分
总结词
换元积分法在计算定积分中的应用
VS
详细描述
通过换元积分法，可以将复杂的定积分转化为容易计算的定积分，从而简化计算过程。例如，利用三角换元法将复杂的定积分转化为简单的定积分。
换元积分法的定义
换元积分法的定义
换元积分法是通过引入新的变量替换原来的变量，将复杂的积分转化为容易积分的积分，从而解决定积分问题的一种方法。
换元积分法的步骤
首先，根据题目要求，选择适当的变量替换原来的变量；然后，根据新的变量，确定积分上下限；最后，进行定积分计算。
换元积分法的公式
三角换元公式
确定新积分上下限
上下限变换原则
根据新变量的定义，将原积分的上下限代入新变量的表达式中，得到新的积分上下限。

第2节换元积分法

或
因为 { F [ ( x )]} f [ ( x )] ( x )
若 f ( u) , ( x )及 ( x )均为连续函数，且
f (u)du F (u) C
则
f [ ( x )] ( x )dx F [ ( x )] C
1/28/2019 6:06 AM
f ( x) f ( x ) f ( x ) [1 ]d x 2 f ( x ) f ( x) f ( x ) f 2 ( x ) f ( x ) f ( x ) [ ]d x 2 f ( x ) f ( x)
1/28/2019 6:06 AM
1 f ( x) 2 f ( x) f ( x) ] C d[ ] [ f ( x ) f ( x ) 2 f ( x )
1 令 u x 3 , 则 xdx du 2 1 1 2 2 x x 3 dx u du 2
2
1 2 1 u C ( x 3) C 3 3
3 2
3 2
1/28/2019 6:06 AM
第5章
不定积分
当运算熟练时，可以不必将 u 写出来。例3 求不定积分解
2 6t 5dt t 3 4 6 dt t t 1 t
1 t2 1 1 dt 6 dt 6 ( t 1)dt 6 1 t 1 t
3t 6t 6ln t 1 C
2
3 3 x 6 6 x 6ln
1/28/2019 6:06 AM
cot xdx ln sin x C
第5章
不定积分
例5
解
dx 求不定积分 2 2 (a 0) a x 1 1 1 dx a 2 x 2 2a ( a x a x )dx 1 1 1 ( dx dx ) 2a a x a x 1 (ln a x ln a x ) C 2a 1 a x ln C 2a a x

2第二节换元积分法

2 sin 2
x 2
1 cos x
csc x cot x.
2 cos x sin x
sin x
2
目录上一页下一页退出
解（二）
csc
xdx
1 sin
x
dx
sin x sin2 x
dx
1
1 cos2 x d(cos x) u cos x
1
1 u2
du
1 2
1
1
u
1
1
u
du
2 cos xd(cos x) cos x2 C.
目录上一页下一页退出
例2
求
3
1 dx. 2x
解
1 1 1 (3 2x),
3 2x 2 3 2x
3
1 2
dx x
1 2
3
1 2
x
(3
2
x)dx
1 2
1du u
1 ln u 2
C
1 2
ln(3
2x)
C.
一般地
f
(ax b)dx
例5 求
1 dx a 0.
a2 x2
解
1 dx 1
a2 x2
a
1 dx
1
x a
2
1
1
x a
2
d
x a
arcsin x C a
目录上一页下一页退出
例6 求 tan xdx.
解
tan xdx
sin cos
x x
dx
1 cos
x
d
cos
x
ln cos x C.
x4

第2讲不定积分的换元积分法

∫
arctan x d x = ∫ 2v d v x (1 + x)
= v2 + C
换元法可以连续使用
= (arctan u ) 2 + C = (arctan x ) 2 + C .
二、不定积分的第二换元法
第一换元法中
∫
f (ϕ ( x))ϕ ′( x) d x = ∫ f (u ) d u 是被积表达式
ϕ ( J ) ⊂ I , 则在区间 J 上有
∫ f (ϕ ( x))ϕ ′( x) d x = ∫ f (u ) d u
= F (u ) + C = F (ϕ ( x)) + C.
证明过程请看书!
该定理称为不定积分的第一换元法,也叫“凑微分”法。
例1 解
求 ∫ sin 3 x cos x d x .
2
π
π
∫
dx a sec 2 t d t =∫ 2 2 a sec t x +a
= ∫ sec t d t
x2 + a2
t
x a
= ln | sec t + tan t | +C1
= ln | x + x 2 + a 2 | + C .
( C = C1 − ln a )
一般说来，含有
a 2 − x 2、 x 2 ± a 2 的表达式的积分，
=∫
(tan x + sec x)′ dx tan x + sec x
= ln | tan x + sec x | +C .
此题若按下面方式做，则有 cos x d x cos x d x du ∫ sec x d x = ∫ cos 2 x = ∫ 1 − sin 2 x = ∫ 1 − u 2 1 u +1 1 sin x + 1 = L = ln + C = ln +C 2 u −1 2 sin x − 1

高等数学《换元法》课件

4.2 换元积分法
4.2.1 第一类换元法（凑微分法） 4.2.2 第二类换元法
机动目录上页下页返回结束
基本思路
设F(u) f (u),
可导, 则有
dF[( x)] f [( x)]( x)dx
F[ ( x)] C F (u) C u( x)
f (u)du u( x)
第一类换元法第二类换元法
de x
(8)
f (ln x)1dx x
dln x
例6 求
解
原式 =
1
dln x 2ln
x
1 2
d(1 2ln x) 1 2ln x
机动目录上页下页返回结束
例7
求
e3
x
x
dx
.
解
原式 = 2 e3
xd
x
2 3
e
3
x d(3
x) 2e3 3
x C
例8 求 sec6 xdx .
解原式 = (tan2 x 1)2dsetca2nxxd x
三角代换外, 还可利用公式 ch2 t sh2 t 1
采用双曲代换 x ash t 或 x a ch t
消去根式, 所得结果一致.
机动目录上页下页返回结束
例19 求
a2 x4
x2
dx
.
解
令
x
1 t
,则
令x a sin t,t ( , )
22
原式
a2
1 t2
1
t4
t
1 4
(1
2cos
2x
cos2
2
x)
1 4
(1
2cos 2x

§4.3 换元积分法

例1 例2 例3 例4 例5
练习1 练习2
巩固练习1：
(1) (5 x 3) dx
11
1 60
(5 x 3) C
12
1 x 2 1 x x (3) xe dx e C 2 1 (4) sin(2 x 1)dx cos(2 x 1) C 2 1 2 ln x ln x C (5) dx 2 x sin x (6) dx arctan(cos x) C 2 1 cos x
例3 例4 例5 练习1 练习2
新课传授：二、第二类换元积分法:

f ( x ) dx
令x=ψ(t)

f [ ( t )] ( t )dt
=(t)+C
t=ψ -1(x)回代
[ψ-1(x)]+C．
例1 例2 例3 例4 例5
练习1 练习2
1、根式代换例题分析例2、求不定积分
(1)
2

1 x dx
2 t 2 )
x
1 x
2
1
解:令 x sin t (
t
则 dx cos tdt , 1 x 2 1 sin 2 t | cos t | cos t
2
1 x dx
cos
2
tdt

1 cos 2 t 2
根据 x sin t 作一辅助直角三角形利用边角关系有
令 u ( x ) f (u ) d (u ) F (u ) C F ( ( x )) C
例1 例2 例3 例4 例5
练习1 练习2
常用的凑微分法：
(1) dx

高教社2024高等数学第五版教学课件-4.2 换元积分法

如果由基本积分公式可以求得
න = +
那么
‫ ⋅ ])([ ׬‬′ ()＝[()] +
将上述过程联立起来，写成下面四个步骤:
න
⋅ ′
= ‫)(])([ ׬‬
凑微分
令=
=
换元

න
= () +
回代
‫])([ ׬‬′ () = [ + ]ȁ=−1() = −1
这种方法称为第二类换元积分法.
+ .
忽略变量符号的不同,下列示意图反映了这两类换元法之间的关
系,从左到右就是第一类换元法,从右到左则是第二类换元法.
第
类
一
元
换
法
令=()
′
‫)( ׬ = )(])([ ׬ = )( ])([ ׬‬
（8） = (− ) = −
（9） 2 =
（10）
1

1+ 2
=
在熟练掌握了上述四个步骤以后，我们可以省略第二步“换元”,从而把
这四个步骤简化为两步:
න
∙ ′ = න
=
+
例3 求
( )3
‫ ׬‬.
解法一
( )3
‫) (׬= ׬‬3
令=ln
回代 1
1 4
3
=
‫ = ׬‬4 + = 4 ( )4 +
解法二
( )3
‫) (׬= ׬‬3
1
= ( )4
分析
‫ ( ׬‬3 + 1) ≠ ( 3 + 1) + ,因为[( 3 + 1)]′ ≠ ( 3 + 1).

大学高等数学ppt课件第三章2第二类换元积分法-分部积分法

解令 u 3 x , 则 xu3, dx3u2du
原式
3u 2 u 1
du
3
u1u11du
3(3u2ulnu1)C 2
3(33x2ln3x1)C 2
例3 求不定积分
dx 1 ex
直接令根式为u，化根式为有理式
解
令
1 ex u,
则
xln
u2 1 ,
dx
2u u2 1
du
原式
1arctanxlnx1ln(1x2)C
x
2
例5 求不定积分 ln 2 xd x
udvuvvdu
解原式 xln2x x2lnx1dx
x
xln2x2lnxdx xln2x2[xlnxdx]
xln 2x 2 xln x 2 x C
udvuvvdu
例6 求不定积分 arcsinxdx
解原式 xarcxsinxdarcxsin
x 1dx x
解令 u x 1, 则 x u 2 1, dx2udu
原式
u u2 1
2udu
2
u2 11 u2 1 du
直接令根式为u，化根式为有理式
2(1u211)du 2uarctanuC
2( x 1arctanx 1)C
dx
例2 求不定积分 3 x 1
直接令根式为u，化根式为有理式
6(111u2 )du
6 (uarctanu)C
6(6xarctan6x)C
◆ 分部积分法
由 uvuvuv
得 u v ud v x u vd u x vdx 即 uvdxuvuvdx 或 udvuvvdu
分部积分公式 udvuv vdu

微积分换元积分法

解题技巧的总结与提炼
观察与分析
在解题过程中，学会观察和分析，识别题型和所使用的换元方法。
灵活运用公式
熟练掌握各种换元公式的形式和特点，根据题目条件灵活运用。
简化计算
在解题过程中，尽量简化计算，避免复杂的运算过程，提高解题效率。
实际问题的应用与解决
物理问题
将换元积分法应用于解决物理问题，如力学、热学等领域的问题。
详细描述
在选择换元变量时，应尽量选择容易处理的变量，如使积分区间变为常见的简单区间或使被积函数形式简化。同时，需要确保换元转换的等价性，即新旧变量之间的转换关系
必须是可逆的。
换元后积分的计算与化简
总结词
换元后需要对新的积分进行计算和化简，这一步涉及到对积分公式和技巧的掌握。
详细描述
在换元后，需要利用已知的积分公式和技巧对新积分进行计算。有时可能需要利用代数方法对积分表达式进行化简，如合并同类项、提取公因式等。此外，还需注意消除积分的上下限，并确保最终结果的正确性。
指数代换
对于形如$x^n$的被积函数，可以使用指数代换将其转换为容易积分的形式。
03
换元积分法的实践应用
三角函数换元法
总结词
通过引入三角函数变量替换，将复杂的积分问题转化为更易于解决的积分问题。
VS
详细描述
三角函数换元法通常用于处理包含平方根或与三角函数相关的积分。通过选择适当的三角函数和变量替换，可以将积分表达式简化，从而更容易计算出结果。
微积分换元积分法
目
CONTENCT
录
• 换元积分法简介 • 换元积分法的基本原理 • 换元积分法的实践应用 • 换元积分法的注意事项与难点 • 换元积分法的练习与提高

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

解决方法利用复合函数，设置中间变量.
令 t 2x dx 1 dt, 2
cos
2
xdx
1 2
cos
tdt
1 2
sin
t
C
1 2
sin
2
x
C
.
2020/3/24
2
利用基本积分表与积分的性质，所能计算的不定积分是非常有限的；我们可以把复合函数的微分法反过来用于求不定积分，利用中间变量的代换，得到复合函数的积分法，称为换元积分法。
x2 1 x2 dx ? 令 x sin t
x2 1 x2 dx (sint)2 1 sin2 t cos tdt
sin2 t cos2 tdt
目的是去掉根式。
2020/3/24
3
二第一类换元法
若 F (u) f (u), 则 f (u)du F (u) C .
设 u ( x)（且可微，根据复合函数微分法，） dF[ ( x)] f [ ( x)] ( x)dx
C
5
5
熟练以后就不需要进行 u ( x) 转化了
2020/3/24
8
例4 求
x (1 x)2 dx.
解
x
(1 x)2 dx
x 11 (1 x)2 dx
1
1
[ (1
x)
(1
x)2
]d
(1
x)
ln(
x
1)
C1
(1
1
x)
C2
ln( x 1) 1 C (1 x)
2020/3/24
cos 3x cos 2x 1 (cos x cos5x), 2
cos
3
x
cos
2 xdx
1 2
(cos
x
cos
5
x)dx
1 sin x 1 sin 5x C.
2
10
2020/3/24
16
例13 求 csc xdx.
解
cs
sin x
sin2 x dx
1
1 cos2 x d(cos x)
第二节换元积分法
(Substitution Rules)
一问题的提出二第一类换元法（凑微分法）三第二类换元法四小结五思考与判断题
2020/3/24
1
一问题的提出
我们知道 cos xdx sin x C
但是
cos2xdx sin2x C,
(sin2x C) cos 2x
2
2
24
例7 sin3 xdx
解 sin3 xdx sin2 x sin xdx
正弦余弦 (三1 角co函s2数x)积d c分os偶x 次幂(co降s x幂齐13 c次os幂3 x拆) 开C
放在微分号d 后面。
2020/3/24
11
例8
求
1 1 e xdx.
解
1
1 e
x
dx
(1
1 e x
)e
x
dx
1
ex ex
dx
ex
1
d(x)
dex
1 ex
1 ex
1
d (1 e x )
1 ex
ln(1 e x ) C .
2020/3/24
12
例9 求 tan5 x sec3 xdx
解 tan5 x sec3 xdx
tan4 x sec2 x secx tan xdx
(sin2 x 2sin4 x sin6 x)d(sin x)
1 sin3 x 2 sin5 x 1 sin7 x C.
3
5
7
说明当被积函数是三角函数相乘时，拆开奇次项去凑微分.
2020/3/24
15
例12 求 cos 3x cos 2xdx.
解利用三角学中的积化和差公式，得
1 2
1du u
1 ln u C 2
1 ln(3 2x) C. 2
2020/3/24
7
例3 求
x(1
1 5
ln
dx. x)
解
1 dx x(1 5ln x)
1
1 5ln
d(l x
n
x)
1 5
1
1 5l
n
d (1 x
5
ln
x)
u
1
1 lnu
2 ln
C
x
1l
1 5
1 u
du
n(1 5ln
x)
1
1 u2
du
1 2
1
1
u
1
1
u
du
1 ln 1 u C 1 ln 1 cos x C.
2 1u
2 1 cos x
ln(cscx cot x) C.
类似地可推出 secxdx ln(secx tan x) C.
剩下的因子2x恰好是u x2的导数，于是有
2xe x2 dx e x2 d( x2 )
eudu eu c
ex2 c
2020/3/24
6
例2 求
3
1 2
dx. x
解
1 1 1 (3 2x),
3 2x 2 3 2x
3
1 dx 2x
1 2
3
1 2
x
(3
2
x)dx
u 3 2x
f [( x)]( x)dx F[( x)] C [ f (u)du]u ( x)
于是可得下述定理
2020/3/24
4
定理1 设 f (u)具有原函数，u ( x)可导，
则有换元公式
f [ ( x)] ( x)dx [ f (u)du]u ( x)
第一类换元公式（凑微分法）
注意使用此公式的关键在于将
(sec2 x 1)2 sec2 xd secx
(sec6 x 2sec4 x sec2 x)d secx
1 sec7 x 2 sec5 x 1 sec3 x C
7
5
3
2020/3/24
13
例10
求
1
1 cos
x
dx.
解
1
1 cos
x
dx
1 cos x 1 cos2 x
dx
1 cos x sin2 x
dx
1
1
sin2 x dx sin2 x d(sin x)
cot x 1 C. sin x
2020/3/24
14
例11 求 sin2 x cos5 xdx.
解 sin2 x cos5 xdx sin2 x cos4 xd(sin x)
sin2 x (1 sin2 x)2 d(sin x)
9
例5 求
a2
1
x 2 dx .
解
a2
1
x 2 dx
1 a2
1
1
x a2
2dx
1 a
1
1
x a
2
d
x a
1 arctan a
x a
C.
2020/3/24
10
例6 求 cos2 xdx
解
cos2 xdx
1 cos 2x dx 2
1 ( dx 1 cox2xd(2x)) x sin2x C
f [ ( x)] ( x)dx f ( ( x))d ( x) F ( ( x)) C
即将 f [( x)]( x)dx拼凑成(( x))d( x)
第一类换元法又称为凑微分法。
2020/3/24
5
例1 求
2xe x2 dx
解被积函数中的一个因子为e x2 eu , u x2 ,