传热学习题课 文件

1-23 Consider a wall heated by convection on one side and cooled by convection on the other side. Show that the heat transfer rate through the wall is:12121//1/T T q h A x kA h A-=+∆+Solution:①To the side of the wall heated by convection, assuming the surface temperature is T W1 on this the side. From Newton’s law of cooling comes the following equation111()W q h A T T =-A is the surface area of the wall.② Consider the conduction of the wall flow one surface T w1 to the other surface T W2,12()W W AKq T T x=-∆ ③ To the side of the wall cooled by convection, from Newton’s low of cooling,222()W q Ah T T =-The heat in above three equations must be equal, so12121//1/T T q h A x kA h A-=+∆+1-24 One side of a plane wall is maintained at 100︒C, while the other side is exposed to a convection environment having T=10︒C and h=10 W/m 2⋅︒C. The wall has k=1.6 W/m ⋅︒C and is 40cm thick. Calculate the heat transfer rate through the wall Solution:The heat-transfer rate is 121total T T k x K h-=∆+T 1=100℃ T 2=10℃20.40.25/6/x mm C w K h w m C∆==⋅⋅ 1/h=0.1m 2•℃/wTherefore, 22210010257/0.25/0.1/C Cq w m m C w m C w-==⋅+⋅2-0 Explain the difference between the thermal conductivity and thermal diffusivity.2-1 A wall 2 cm thick is to be constructed from material which has an average thermal conductivity of 1.3 W/m ⋅︒C. The wall is to be insulated with material having an average thermal conductivity of 0.35 W/m ⋅︒C, so that the heat loss per square meter will not exceed 1830 W. Assuming that the inner and outer surface temperatures of the insulated wall are 1300 and 30 ︒C, calculate the thickness of insulation required. Solution:21211830/wall insuwall insu T T q w m x x A A K A K A-=⋅≤∆∆+ Therefore, 0.238insu x m ∆≥the thickness of the insulation requires of least 23.8cm.2-47 Derive an expression for the temperature distribution in a sphere of radius r1 with uniform heat generation and constant surface temperature T w . SolutionThe differential equation which governs the heat flow is221()0d rt qr dr k+= The boundary conditions areT Tw = at r=R,()0d rt dr= at r=0 We rewrite Equation()d d rt qr dr dr k⎛⎫=- ⎪⎝⎭ Then integration yields21()2d rt qr C dr k =-+ and rC C k r q T 2126++⋅-= According to the boundary conditions rC k R qT C w 2216-+= =0 Therefore, the final solution for the temperature distribution is )(622r R kqT T w -=-2-57温度为120℃的空气从导热系数为λ1 = 18 W/(m ⋅K)的不锈钢管内流过，表面传热系数为h 1 = 65 W/(m 2⋅K)，管内径为d 1 = 25 mm ，厚度为0.4 mm 。

传热学课习题第1章习题4. 面积为l m 2、厚度为25mm 的聚氨酯泡沫塑料平板，其两表面的温差为 5℃，导热系数为 0.032W/(m ·K)，试计算单位时间通过该平板的热量。

8. 面积为 3×4m 2的一面墙壁，表面温度维持 60℃，环境空气温度维持20℃，空气与壁面的对流换热系数为10W/(m 2·K)，试计算这面墙壁的散热量。

9. 一块黑度为0.8的钢板，温度为27℃，试计算单位面积上每小时内钢板所发射的辐射能。

10. 冬季室内空气温度 1f t =20℃，室外空气温温度 2f t =-25℃。

室内、外空气对墙壁的对流换热系数分别为 1α=10 W/(m 2·K)和 2α= 20 W/(m 2·K)，墙壁厚度为δ= 360mm ，导热系数λ=0.5W/(m ·K)，其面积F =15m 2。

试计算通过墙壁的热量损失。

第2章习题4. 试用傅里叶定律直接积分的方法，求平壁、长圆筒壁及球壁稳态导热下的热流量表达式及各壁内的温度分布。

5. 一铝板将热水和冷水隔开，铝板两侧面的温度分别维持 90℃和 70℃不变，板厚 10mm ，并可认为是无限大平壁。

0℃时铝板的导热系数λ=35.5 W/(m ·K)，100℃时λ=34.3 W/(m ·K)，并假定在此温度范围内导热系数是温度的线性函数。

试计算热流密度，板两侧的温度为50℃和30℃时，热流密度是否有变化？6. 厚度为20mm 的平面墙的导热系数为 1.3 W/(m ·K)。

为使通过该墙的热流密度q 不超过 1830W/m 2，在外侧敷一层导热系数为0.25 W/(m ·K)的保温材料。

当复合壁的内、外壁温度分别为 1300℃和50℃时，试确定保温层的厚度。

9. 某大平壁厚为25mm ，面积为0.1m 2，一侧面温度保持38℃，另一侧面保持94℃。

通过材料的热流量为1 kW 时，材料中心面的温度为60℃。

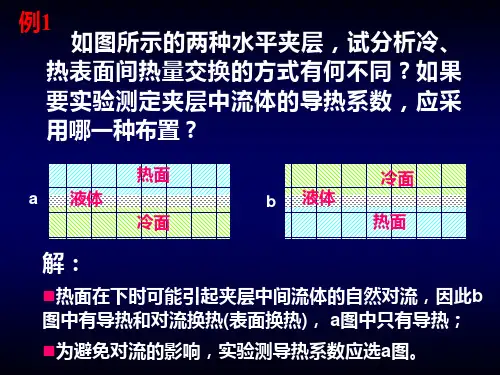
第1章绪论习题1-1 一大平板，高3m、宽2m、厚0.02m，导热系数为45 W/(m·K)，两侧表面温度分别为t1 = 100℃、t2 = 50℃，试求该平板的热阻、热流量、热流密度。

1-2 一间地下室的混凝土地面的长和宽分别为11m和8m，厚为0.2m。

在冬季，上下表面的标称温度分别为17℃和10℃。

如果混凝土的热导率为1.4 W/(m·K)，通过地面的热损失率是多少？如果采用效率为ηf = 0.90的燃气炉对地下室供暖，且天然气的价格为C g = $0.01/MJ，每天由热损失造成的费用是多少？1-3 空气在一根内径50mm，长2.5m的管子内流动并被加热，已知空气平均温度为80℃，管内对流传热的表面传热系数为h = 70W/(m2·K)，热流密度为q = 5000W/m2，试求管壁温度及热流量。

1-4 受迫流动的空气流过室内加热设备的一个对流换热器，产生的表面传热系数h = 1135.59 W/(m2·K)，换热器表面温度可认为是常数，为65.6℃，空气温度为18.3℃。

若要求的加热功率为8790W，试求所需换热器的换热面积。

1-5 一电炉丝，温度为847℃，长1.5m，直径为2mm，表面发射率为0.95。

试计算电炉丝的辐射功率。

1-6 夏天，停放的汽车其表面的温度通常平均达40～50℃。

设为45℃，表面发射率为0.90，求车子顶面单位面积发射的辐射功率。

1-7 某锅炉炉墙，内层是厚7.5cm、λ = 1.10W/(m·K)的耐火砖，外层是厚0.64cm、λ = 39W/(m·K)的钢板，且在每平方米的炉墙表面上有18只直径为1.9cm的螺栓[λ = 39W/(m·K)]。

假定炉墙内、外表面温度均匀，内表面温度为920K，炉外是300K的空气，炉墙外表面的表面传热系数为68 W/(m2 ·K)，求炉墙的总热阻和热流密度。

1-1为测定某材料的导热系数，用该材料制成厚5mm的大平壁，保持平壁两表面间的温差为30℃，并测得通过平壁的热流密度为6210W/m2。

试确定该材料的导热系数。

q=λΔtδ⟹λ=qδΔt=6210×0.00530=1.035W/mK1-6 在测定空气横掠单根圆管的对流传热实验中，得到如下数据：管壁平均温度t w=60℃，空气温度t f =20℃，管子外径d =14mm，加热段长L=80mm，输入加热段的功率Φ=8.6kW。

如果全部热量通过对流换热传给空气，问此时对流传热的表面传热系数多大？Q=hA∆t⟹h=QA∆t=Qπdl∆t=86003.14×0.014×0.08×(60−20)=61135W/m2K1-7 一电炉丝，温度为847℃，长1.5m ，直径2mm，表面发射率为0.95。

试计算电炉丝的辐射功率。

Q=εσAT4= εσπdlT4=0.95×5.67×3.14×0.002×1.5×(8.47+2.73)4= 798.42W2-2 厚度为100mm的大平壁稳态导热时的温度分布曲线为t=a+bx+cx2（x的单位为m），其中a=200℃，b=-200℃/m，c=30℃/m2，材料的导热系数为45 W/(m⋅K)。

（1）试求平壁两侧壁面处的热流密度；（2）该平壁是否存在内热源？若存在的话，强度是多大？(1)q(x)=−λdtdx=−45×(b+2cx)=−45×(−200+60x)=9000−2700xq(0)=9000W/m2q(0.1)= 9000−270=8730W/m2 (2)q v=8730−90000.1=−2700W/m33-5 平壁内表面温度为420℃，采用石棉作为保温材料，若保温材料的导热系数与温度的关系为λ=0.094+0.000125{t}℃ W/(m⋅K)，平壁保温层外表面温度为50℃，若要求热损失不超过340W/m2，问保温层的厚度应为多少？保温层平均温度t=0.5×(420+50)=235℃平均导热系数λ̅=0.094+0.000125{t}=0.094+0.000125×235=0.1234W/(m⋅K)q=λΔtδ⟹δ=λΔtq=0.1234×420−50340=0.134m3-26一种火焰报警器采用低熔点的金属丝作为传感元件，其原理是当金属丝受火焰或高温烟气作用而熔断时，报警系统即被触发。