机器人学第二章

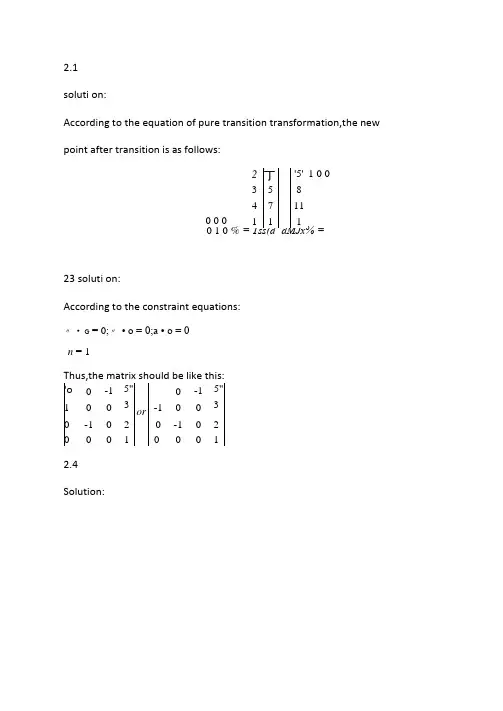
2.1 soluti on:According to the equation of pure transition transformation,the new point after transition is as follows:1 0 0 0 1 0 % = Tss(d“dMJx% =23 soluti on:According to the constraint equations:〃 • G = 0;〃 • o = 0;a • o = 0n = 1Thus,the matrix should be like this: 'o 0 -1 5"0 -1 5"1 00 3 or -1 0 0 30 -1 0 2 0 -1 0 2 0 0 0 1_0 0 0 12.4 Solution:2 丁 '5'3 58 4 7111 110 0 0coordinates are as follows:2.9Solution:Acording to the equations for the combined transformations ,the new coordinates are as follows:According to the equation of purerotation transformation , the new化川=s 心,45°)xP =Solution:V2 2返2o-丁'1 ' 0 310 0 49 1 110 1 0 00 -1 t . 1 0 AP = Rot(z,90 )X Trans(5y 3,6)x Rot(x.90°)x P= ° Q0 0Transformations relative to the reference frameTransformations relative to the current frame 2.10 A P=Trans(5,3,6)Rot(x,90)Rot(a,90) P二 -28 12.12而.527 0.369 -0.766 -0.601、T11 二-0.5740.819 0 -2.9472.14 a) For spherical coordinates we have (for posihon )1) r • cos y • sin 0 二 3.1375 2) r • siny • sin p = 2.195 3) r • cos P = 3.214I) Assuming sin P is posihve, from a and b —> y 二350 0o'「0-1 00\r3 0 0 -1 0 1 0 0 0 361 0 00 1 0 5 V0 0 lyJ< 1 >0 10 0 0 1 <0 0 0 0.6280.4390.643-5.380.92 -0.39 -3.82 -60.390.92 -3.79from b and c t 卩二50°r r unitsfrom c — r=5II) If sin p were negative. ThenY 二35°0 二50°r 二护tsSince orientation is not specified, no more information is available to check the results・b) For case I, substifa te corresp on ding values of sinR , cosp, siny, cosyand r in sperical coordinates to get:/O5265 -0.5735 0.6275 3.1375^ Tsph(Gp?Y)=Tsph(35,50,5)= 0.3687 0.819 0.439 2.195-0.766 0 0.6428 3.214< °0 0—2.16Solution:According to the equations given in the text book, we can get the Euler angles as follows:①①=arctan arctan 2(a v,a,)Which lead to :① = 215」o 厂35° ②W = arctan+”、C ①，一 yS ①+①）=0 or\80③ 。

第二章机器人基础知识2.3工业机器人运动学(一)【内容提要】本课主要学习工业机器人技术的运动学基础知识，涉及机器人正逆运动学的概念、平面二连杆机器人的运动学、以及机器人一般运动学的数学基础（位姿描述、齐次变换及运算）。

知识要点：✓机器人正逆运动学概念✓平面二连杆机器人的正逆运动学✓机器人的位姿描述✓齐次变换及运算重点：✓掌握机器人正逆运动学概念✓掌握平面二连杆机器人的正逆运动学✓理解机器人的位姿描述和齐次变换✓掌握齐次变换及运算难点：✓机器人的位姿描述、齐次变换及运算关键字：✓机器人正逆运动学、平面二连杆机器人、位姿描述、齐次变换及运算【本课内容相关资料】2.3机器人运动学从机构学的角度看，机器人可以看成开式运动链结构，由一系列连杆通转动或移动关节串联而成。

机器人运动学研究的是机器人各关节运动的几何关系，具体而言是各连杆之间的位移关系、速度关系和加速度关系。

本节仅研究位移关系，重点是研究手部相对于机座的位姿与各连杆之间的相互关系。

“位姿”是“位置和姿态”的简称。

工业机器人手部相对于机座的位姿与工业机器人各连杆之间的相互关系直接相关。

为了便于数学上的分析，一般将连杆和关节按空间顺序进行编号。

同时，选定一个与机座固联的坐标系，称为固定坐标系，并为每一个连杆（包括手部）选定一个与之固联的坐标系，称为连杆坐标系。

一般把机座也视为一个连杆，即零号连杆。

这样，连杆之间的相互关系可以用连杆坐标系之间的相互关系来描述。

工业机器人手部相对机座的位姿就是固联在手部的坐标系相对固定坐标系的位姿。

这样，就可以将“手部相对于机座的位姿”这样一个物理问题转化为一个数学问题，即，得到了工业机器人的运动学数学模型，便于用计算机进行分析计算。

工业机器人运动学主要包括正向运动学和反向运动学两类问题。

正向运动学是在已知各个关节变量的前提下，解决如何建立工业机器人运动学方程，以及如何求解手部相对固定坐标系位姿的问题。

反向运动学则是在已知手部要到达目标位姿的前提下，解决如何求出关节变量的问题。