
Linear Algebra and its Applications xxx (2011)
xxx–xxx
Contents lists available at ScienceDirect
Linear Algebra and its Applications
journal homepage:
https://www.doczj.com/doc/952964506.html,/locate/laa
A note on the Jacobian Conjecture
Dan Yan
School of Mathematical Sciences,Graduate University of Chinese Academy of Sciences,Beijing 100049,China
A R T I C L E I N F O
A B S T R A C T Article history:
Received 19December 2010
Accepted 11January 2011
Available online xxxx
Submitted by R.A.Brualdi
AMS classi?cation:
Primary:14E05
Secondary:14A05,14R15
Keywords:
Jacobian Conjecture
Polynomial mapping
Druzkowski mapping In this note,we show that,if the Druzkowski mappings F (X )=X +(AX )?3,i.e.F (X )=(x 1+(a 11x 1+···+a 1n x n )3,...,x n +(a n 1x 1+···+a nn x n )3),satisfy TrJ ((AX )?3)=0,then rank (A ) 12(n +δ)where δis the number diagonal elements of A which are equal to zero.Furthermore,we show the Jacobian Conjecture is true for the Druzkowski mappings in dimension 9in the case n i =1a ii =0.?2011Elsevier Inc.All rights reserved.
Let F
=(F 1(x 1,...,x n ),...,F n (x 1,...,x n ))t :C n →C n be a polynomial mapping,that is,F i (x 1,...,x n )∈C [x 1,...,x n ]for all 1 i n .Let JF = ?F i ?x j n ×n
be the Jacobian matrix of F .The well-known Jacobian Conjecture (JC)raised by Keller [1]states that a polynomial mapping F :C n →C n is invertible if the Jacobian determinant |JF |is a nonzero constant.This conjecture has being attacked by many people from various research ?elds and remains open even when n =2!(Of course,a positive answer is obvious when n =1by the elements of linear algebra.)See [2,3]and the references therein for a wonderful 70-years history of this famous conjecture.It can be easily seen that JC is true if JC holds for all polynomial mappings whose Jacobian determinant is 1.We make use of this convention in the present paper.Among the vast interesting and valid results,a relatively satisfactory result obtained by Wang [4]is that JC holds for all polynomial mappings of degree 2in all dimensions.The most powerful and surprising result is the reduction to degree 3,due to Bass et al.[2]and Yagzhev
[5],which asserts that JC is true if JC holds for all polynomial mappings of degree 3(what is more,if JC holds for all cubic homogeneous polynomial mapping!).In the same spirit of the above degree reduction method,another ef?cient way to tackle JC is the Druzkowski’s Theorem [6]:JC is true if it is true for all Druzkowski mappings (in all dimension 2).
E-mail address:yan-dan-hi@https://www.doczj.com/doc/952964506.html,
0024-3795/$-see front matter ?2011Elsevier Inc.All rights reserved.
doi:10.1016/https://www.doczj.com/doc/952964506.html,a.2011.01.016
2 D.Yan /Linear Algebra and its Applications xxx (2011)xxx–xxx
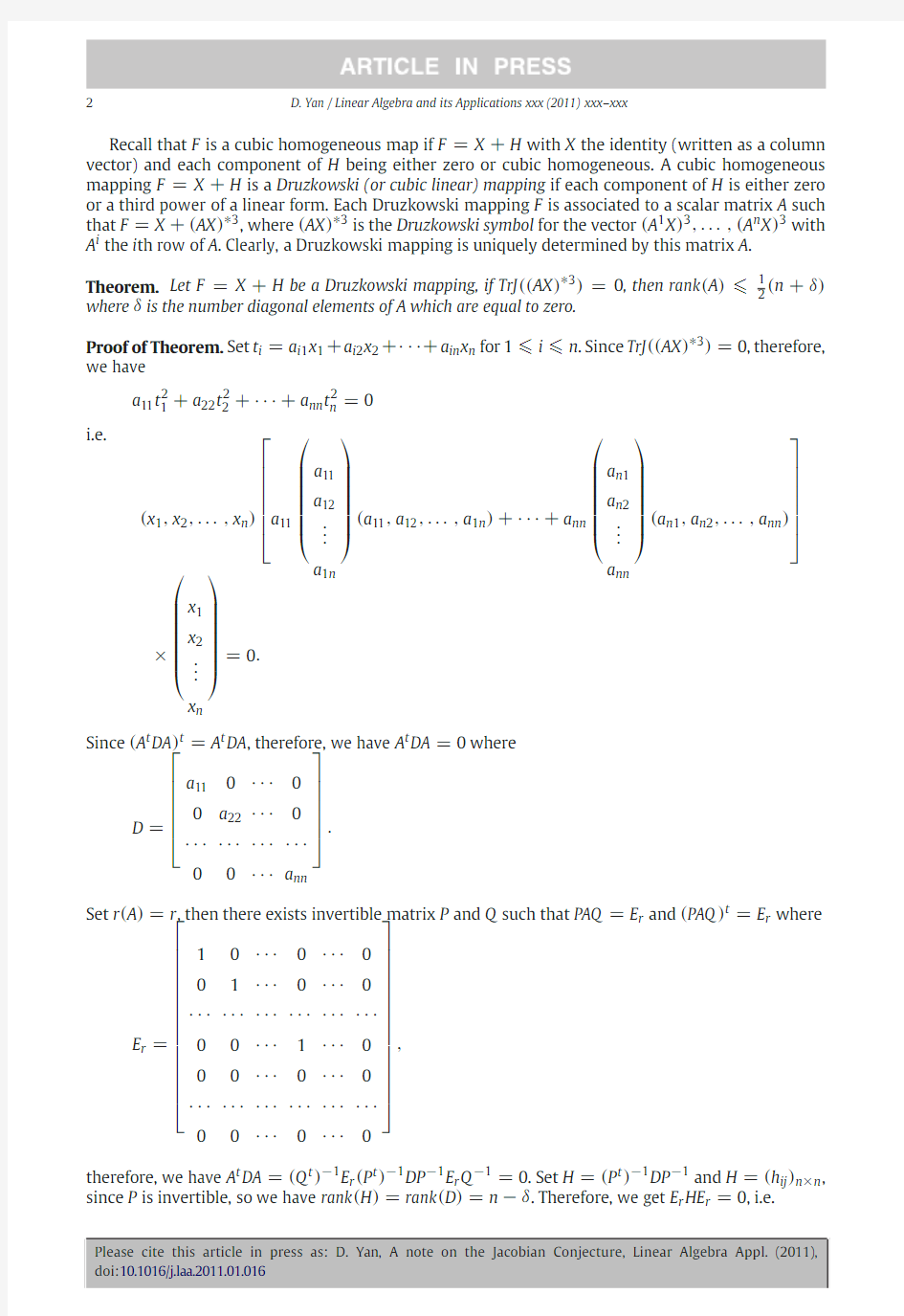
Recall that F is a cubic homogeneous map if F =X +H with X the identity (written as a column vector)and each component of H being either zero or cubic homogeneous.A cubic homogeneous mapping F =X +H is a Druzkowski (or cubic linear)mapping if each component of H is either zero or a third power of a linear form.Each Druzkowski mapping F is associated to a scalar matrix A such that F =X +(AX )?3,where (AX )?3is the Druzkowski symbol for the vector (A 1X )3,...,(A n X )3with A i the i th row of A .Clearly,a Druzkowski mapping is uniquely determined by this matrix A .
Theorem.Let F =X +H be a Druzkowski mapping,if TrJ ((AX )?3)
=0,then rank (A ) 12(n +δ)where δis the number diagonal elements of A which are equal to zero.
Proof of Theorem.Set t i
=a i 1x 1+a i 2x 2+···+a in x n for 1 i n .Since TrJ ((AX )?3)=0,therefore,we have
a 11t 21+a 22t 22+···+a nn t 2n =0
i.e.(x 1,x 2,...,x n )?????????a 11?????????
a 11a 12...a 1n ?????????(a 11,a 12,...,a 1n )+···+a nn ?????????a n 1a n 2...a nn ?????????(a n 1,a n 2,...,a nn )?
????????×?????????x 1x 2...x n
?
????????
=0.Since (A t DA )t
=A t DA ,therefore,we have A t DA =0where
D =????????a 110 0
0a 22···
0·········
···00···a nn
????????.Set r (A )=r ,then there exists invertible matrix P and Q such that PAQ =E r and (PAQ )t =E r where
E r =?
?????????????????
10...0...001...0...0..................00...1...000...0...0..................00...0 0
??????????????????,therefore,we have A t DA =(Q t )?1E r (P t )?1DP ?1E r Q ?1=0.Set H =(P t )?1DP ?1and H =(h ij )n ×n ,since P is invertible,so we have rank (H )=rank (D )=n ?δ.Therefore,we get E r HE r =0,i.e.
D.Yan /Linear Algebra and its Applications xxx (2011)xxx–xxx 3
?
?????????????????
h 11h 12...h 1r 0...0h 21h 22...h 2r 0...0.....................h r 1h r 2...h rr 0...000...00...0 (00)
···00···0??????????????????=0.Since rank (H )
=n ?δ,so r n +δ?r .Therefore,we have r 12
(n +δ).Which complete the proof. Since the Jacobian Conjecture is true for all cubic homogeneous polynomials in dimension 4and we know if rank A 4(see [8])F and G are paired,where G are cubic homogeneous polynomials in dimension no more than 4which related to F (see Theorem 2in [9]).Therefore,we have the following conclusion:
Corollary.Let F =X +H be a Druzkowski mapping,if
n i =1a ii =0,then the Jacobian Conjecture is true for the Druzkowski mappings in dimension 9.
In the proof of the theorem,we have r (A )
n 2.Now we give an example to show that is the best bound for rank (A )in such case.
Example.Let n =4,
???????????????F 1=(x 1+ix 2+x 3+x 4)3+x 1F 2=i (x 1+ix 2+x 3+x 4)3+x 2
F 3=?(x 1+ix 2?x 3+x 4)3+x 3F 4=?(x 1+ix 2?x 3+x 4)3+x 4
(1)
then we have A =????????1i 11
?i 1?i ?i ?1?i 1?1?1?i 1?1????????,clearly,F is invertible and rank (A )
=2.
Acknowledgments The author is very grateful to Professor Yuehui Zhang who introduced the Conjecture and gave great help when the author studied the problem and Professor Guoping Tang who read the paper carefully and gave some good advice.
References
[1]O.H.Keller,Ganze Cremona-transformationen Monatschr,Math.Phys.47(1939)229–306.
[2]H.Bass,E.Connell,D.Wright,The Jacobian Conjecture:reduction of degree and formal expansion of the inverse,Bull.Amer.Math.
Soc.7(1982)287–330.
4 D.Yan/Linear Algebra and its Applications xxx(2011)xxx–xxx
[3]Arno van den Essen,Polynomial Automorphisms and Jacobian Conjecture,?rst ed.,Birkhauser,Basel,2000.
[4]S.S.S.Wang,A Jacobian criterion for separability,J.Algebra65(1980)453–494.
[5]A.V.Yagzhev,On Keller’s problem,Siberian Math.J.21(1980)747–754.
[6]L.M.Druzkowski,An effective Approach to Keller’s Jacobian Conjecture,Math.Ann.264(1983)303–313.
[8]E.Hubbers,Nilpotent Jacobians,Ph.D.Thesis,University of Nijmegen,Toernooiveld,6525ED Nijmegen,The Netherlands,1998.
[9]Dan Yan,Some results on the Jacobian Conjecture and polynomial automorphisms,J.Pure Appl.Algebra,submitted for publication. Further reading
[7]G.Gorni,G.Zampieri,On the cubic-linear polynomial mappings,Indag.Math.8(4)(1997)471–492.