关于举办第二届丘成桐大学生数学竞赛
的通知
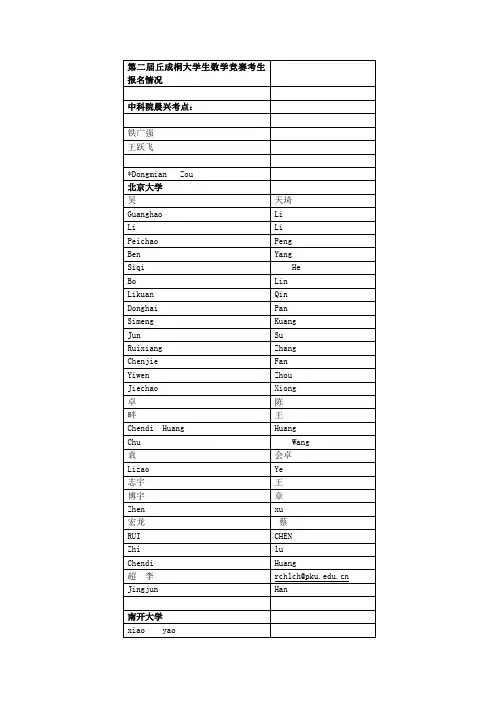
经丘成桐大学生数学竞赛组委会研究决定，第二届丘成桐大学生数学竞赛笔试将于2011年7月9日至10日举行。届时竞赛组委会将组织专家集中阅卷，评选出参加决赛(面试)的团队和个人名单。第二届丘成桐大学生数学竞赛决赛将于2011年8月19日至20日在北京举行。竞赛组委会现已开通了第二届丘成桐大
学生数学竞赛网上报名系统：
https://www.doczj.com/doc/0d10392493.html,/registration/register.php报名截止日期为2011年6月20日。参加过首届丘成桐大学生数学竞赛的在读大学生仍可报名参加第二届丘成桐大学生数学竞赛。欢迎中国(包括大陆、香港、澳门和台湾)高校在读大学生踊跃报名参加第二届丘成桐大学生数学竞赛。
丘成桐大学生数学竞赛组委会
2011年3月11日
当代著名数学家丘成桐教授最近提议，在中国（包括中国大陆、香港和台湾）的高校开展大学生数学竞赛，以全面测试大学生的数学知识、修养与能力，促进中国的大学数学教育改革。丘先生领衔的学术与命题委员会将基础数学与应用数学凝练成分析与微分方程，几何与拓扑，代数、组合与数论，计算、统计与应用数学等四个方面，并将提供详细的大纲与参考书。测试范围和国外知名大学的数学资格考试相当。测试成绩将为国内外录取研究生提供重要的参考，这将为年轻学子进入现代数学大门提供有利的条件。
中国是泱泱大国，可造就之人才不可胜数，关键是全面提高中国数学人才的修养与能力，尤其是正在成长中的新生力量能得到有效、及时的指导。近现代数学硕果累累，大师不断，是现代文明的瑰宝。继承、发展现代数学也是中国贡献于世界的重要方面。这一竞赛将对中国的大学数学教育起良好的促进作用，在课程设置和思维方法上与国际一流的数学系接轨，通过测试的学子有望通过国内外的数学博士资格考试。此举将为中国和世界数学提供源源不断的优异的后备力量，其中将涌现出许多优秀的数学家。本竞赛将全面提升中国的大学数学教育，为把中国的人口大国变成人才大国做贡献。
就举办大学生数学竞赛致数学同仁的一封公开信
尊敬的同仁，
我们最近倡议举办大学生数学竞赛，特写此信向您说明为何要举办这样的竞赛。我们希望竞赛能够达到如下的目标：
1．培养一位好的数学家，一个重要的步骤是在他们开展研究工作时具备数学的基础知识和技能。为此，世界主要的数学机构都为研究生设置资格考试。中国好的学生应该能通过世界上主要数学机构的资格考试，例如哈佛、斯坦福、伯克利、普林斯顿、哥伦比亚等。可是近年来，这些学校来自中国的学生常常有通不过资格考试的情况，而来自东欧的好学生则无问题。中国大学数学竞赛的竞赛内容将足够广泛与基本。这样将有助于为中国和世界的数学机构提供良好的数学生源，为中国和世界的数学发展培植深厚的土壤。
2．应当为没能进入到中国顶尖大学的学生提供公平的竞争机会。这一点很重要。一个高中生可能有很多原因使得他（她）没能进入到顶尖大学，例如运气不好、身体不适、贫穷或者不成熟等。可是这些情况可能会发生变化，而这些学生突然决定要好好干。可在目前中国的情况下他们到世界和中国一流机构深造的机会较少。我们应当给他们以机会。我本人在香港就有类似的经历。我曾经很难进入到一流大学，经过艰苦的努力与奋斗才得到承认。
3．要让在顶尖学校学习的学生感到在数学上经常有压力和挑战，使得他们刻苦工作，保持一个良好的工作状态。现在经常有来自中国大陆的学生大谈数学的哲学，而不能坐下来做扎实的计算。这个竞赛的内容就是数学的基本知识与基本功，通过这个竞赛将能够非常有效地改变这种状况。
4．我们将邀请世界著名的数学家口试我们竞赛优秀的学生（暂定前15名）。我相信许多一流数学家将会乐意面试我们的优秀学生。这些数学家将亲自鉴定这些学生的水平与能力，为他们进入世界一流的研究院提供帮助。
总之，我相信这个竞赛将把中国大学生的数学教育提高到一个新的水平。我们诚请您的学生参加这次大学生数学竞赛。
致礼
丘成桐
哈佛大学数学系主任
丘成桐大学数学竞赛学术与命题委员会主席
二零一零年七月十一日https://www.doczj.com/doc/0d10392493.html,//conference/YCMC/rules-c.html
首届的有关资料供参考
--------------------------竞赛规则
1 本竞赛面向中国大陆、香港、台湾高校的尚未取得学士学位的本科在校生。
2 各高校可组队参加。每队由四位队员组成。每校不超过两个队。各高校个人参赛的名额没有限制。团体赛与个人赛分别举行。比赛共两天。
3 第一天早晨举行团体赛。在团体比赛中，每队队员先用半小时时间讨论分题目。而后的两个半小时各位队员独立做题。
4 在余下的一天半举行个人赛。每个科目的做题时间为两个半小时。
5 在比赛中，不允许队员讨论或与外界联系。
竞赛内容
竞赛将全面测试学生的知识与技能。测试将分成4个科目：
1 分析与微分方程
2 几何与拓扑
3 代数、数论与组合
4 应用、计算和概率统计
竞赛学术与出题委员会将提供每个科目的详细大纲和参考书。每个科目将有五道题目。每个参加者可选其中的三个科目。
竞赛前15名优胜者将参加2010年10月14日的口试。
打分
每题20分。全部正确解决问题的得满分。解题中有一定想法和进展的可得部分分数。
竞赛时间
首次竞赛在2010年10月12日举行。
竞赛地点
竞赛将在中国大陆、香港、台湾的主要大学举行。
注册
可从浙江杭州浙江大学数学中心及网页索取注册材料。每位参加者应在八月中之前完成注册。
参考文献
Geometry and T opology (preliminary draft)
Differential geometry of curves and surfaces
Differentia geometry of curves and surfaces.
M. do Carmo: Prentice- Hall, 1976 (25th printing)
Table of Contents
1. Curves: Parametrized Curves.
2. Regular Surfaces: Regular Surfaces; Inverse Images of Regular Values.
3. Geometry of the Gauss Map: Definition of the Gauss Map and Its Fundamental Properties.
4. Intrinsic Geometry of Surfaces: Isometrics; Conformal Maps.
5. Global Differential Geometry: Rigidity of the Sphere.
Differential Manifolds and Riemannian Geometry
An Introduction to Differentiable Manifolds and Riemannian Geometry. W.M. Boothby: Academic Press, Inc., Orlando, FL, 1986
Contents
I. Introduction to Manifolds
1. Preliminary Comments on Rn
2. Rn and Euclidean Space 4
3. Topological Manifolds 6
4. Further Examples of Manifolds. Cutting and Pasting Abstract Manifolds. Some Examples 14
II. Functions of Several Variables and Mappings
1. Differentiability for Functions of Several Variables 20
2. Differentiability of Mappings and Jacobians 25
3. The Space of Tangent Vectors at a Point of Rn 29
4. Another Definition of Ta(Rn) 32
5. Vector Fields on Open Subsets of Rn
6. The Inverse Function Theorem 41
7. The Rank of a Mapping 46
III. Differentiable Manifolds and Submanifolds
1. The Definition of a Differentiable Manifold
2. Further Examples 59
3. Differentiable Functions and Mappings
4. Rank of a Mapping, Immersions 68
5. Submanifolds 74
6. Lie Groups 80 (*)
7. The Action of a Lie Group on a Manifold. Transformation Groups (*)
8. The Action of a Discrete Group on a Manifold 93 (*)
9. Covering Manifolds 98 (*)
IV. Vector Fields on a Manifold
1. The Tangent Space at a Point of a Manifold
2. Vector Field 113
3. One-Parameter and Local One-Parameter Groups Acting on a Manifold
4. The Existence Theorem for Ordinary Differential Equations 127
5. Some Examples of One-Parameter Groups Acting on a Manifold (*)
6. One-Parameter Subgroups of Lie Groups 142 (*)
7. The Lie Algebra of Vector Fields on a Manifold (*)
8. Frobenius's Theorem 153 (*)
9. Homogeneous Spaces 160 (*)
V. Tensors and Tensor Fields on Manifolds
1. Tangent Covectors 171
Covectors on Manifolds 172
Covector Fields and Mappings 174
2. Bilinear Forms. The Riemannian Metric
3. Riemannian Manifolds as Metric Spaces
4. Partitions of Unity 186
Some Applications of the Partition of Unity
5. Tensor Fields 192
Tensors on a Vector Space
Tensor Fields 194
Mappings and Covariant Tensors 195
The Symmetrizing and Alternating Transformations
6. Multiplication of Tensors 199
Multiplication of Tensors on a Vector Space
Multiplication of Tensor Fields 201
Exterior Multiplication of Alternating Tensors
The Exterior Algebra on Manifolds 206
7. Orientation of Manifolds and the Volume Element
8. Exterior Differentiation 212
An Application to Frobenius's Theorem 177
VI. Integration on Manifolds
1. Integration in Rn Domains of Integration 223
Basic Properties of the Riemann Integral 224
2. A Generalization to Manifolds 229
Integration on Riemannian Manifolds
3. Integration on Lie Groups 237
4. Manifolds with Boundary 243
5. Stokes's Theorem for Manifolds 251
6. Homotopy of Mappings. The Fundamental Group 258 (*)
Homotopy of Paths and Loops. The Fundamental Group (*)
7. Some Applications of Differential Forms.
The de Rham Groups
The Homotopy Operator 268 (*)
8. Some Further Applications of de Rham Groups (*)
The de Rham Groups of Lie Groups 276 (*)
9. Covering Spaces and Fundamental Group 280 (*)
VII. Differentiation on Riemannian Manifolds
1. Differentiation of Vector Fields along Curves in Rn
The Geometry of Space Curves 292
Curvature of Plane Curves 296
2. Differentiation of Vector Fields on Submanifolds of Rn
Formulas for Covariant Derivatives 303
Differentiation of Vector Fields 305
3. Differentiation on Riemannian Manifolds 308
Constant Vector Fields and Parallel Displacement
4. Addenda to the Theory of Differentiation on a Manifold
The Curvature Tensor 316 (*)
The Riemannian Connection and Exterior Differential Forms (*)
5. Geodesic Curves on Riemannian Manifolds 321 (*)
6. The Tangent Bundle and Exponential Mapping. Normal Coordinates (*)
7. Some Further Properties of Geodesics 332 (*)
8. Symmetric Riemannian Manifolds 340 (*)
9. Some Examples 346 (*)
VIII. Curvature
1. The Geometry of Surfaces in E^3
The Principal Curvatures at a Point of a Surface 359
2. The Gaussian and Mean Curvatures of a Surface 363
The Theorema Egregium of Gauss 366
3. Basic Properties of the Riemann Curvature Tensor
4. Curvature Forms and the Equations of Structure
5. Differentiation of Covariant Tensor Fields 384 (*)
6. Manifolds of Constant Curvature 391 (*)
Spaces of Positive Curvature 394
Spaces of Zero Curvature 396
Spaces of Constant Negative Curvature
Other references:
M. Spivak, A comprehensive introduction to differential geometry
N. Hicks, Notes on differential geometry, Van Nostrand.
J. Milnor, Morse Theory
Basic topology
M. Armstrong, Springer, Undergraduate texts in mathematics
Table of contents:
Preface. 1: Introduction. 2: Continuity. 3: Compactness and connectedness. 4: Identification spaces. 5: The fundamental group. 6: Triangulations. 7: Surfaces. 8: Simplic ial homology. 9: Degree and Lefschetz number. 10: Knots and covering spaces. Appendix: Generators and relations.
Algebraic topology
Algebraic topology
By A Hatcher (https://www.doczj.com/doc/0d10392493.html,/~hatcher/A T/A Tpage.html)
Chapter 0. Some Underlying Geometric Notions
Homotopy and Homotopy Type. Cell Complexes. Operations on Spaces. Two Criteria for Homotopy Equivalence. The Homotopy Extension Property. Chapter 1. Fundamental Group and Covering Spaces
1. Basic Constructions.
Paths and Homotopy. The Fundamental Group of the Circle. Induced Homomorphisms.
2. V an Kampen's Theorem
Free Products of Groups. The van Kampen Theorem. Applications to Cell Complexes.
3. Covering Spaces
Lifting Properties. The Classification of Covering Spaces. Deck Transformations and Group Actions.
4. Additional Topics (*)
Graphs and Free Groups. K(G,1) Spaces and Graphs of Groups.
Chapter 2. Homology
1. Simplicial and Singular Homology
Delta-Complexes. Simplicial Homology. Singular Homology. Homotopy Invariance. Exact Sequences and Excision. The Equivalence of Simplicial and Singular Homology.
2. Computations and Applications
Degree. Cellular Homology. Mayer-Vietoris Sequences. Homology with Coefficients.
3. The Formal Viewpoint (*)
Axioms for Homology. Categories and Functors.
4. Additional Topics (*)
Homology and Fundamental Group. Classical Applications. Simplicial Approximation.
Chapter 3. Cohomology
1. Cohomology Groups
The Universal Coefficient Theorem. Cohomology of Spaces.
2. Cup Product
The Cohomology Ring. A Kunneth Formula. Spaces with Polynomial Cohomology.
3. Poincare Duality
Orientations and Homology. The Duality Theorem. Cup Product and Duality.
Other Forms of Duality.
4. Additional Topics (*)
The Universal Coefficient Theorem for Homology. The General Kunneth Formula. H-Spaces and Hopf Algebras. The Cohomology of SO(n). Bockstein Homomorphisms. Limits. More About Ext. Transfer Homomorphisms. Local Coefficients.
Chapter 4. Homotopy Theory (*)
1. Homotopy Groups
Definitions and Basic Constructions. Whitehead's Theorem. Cellular Approximation. CW Approximation.
2. Elementary Methods of Calculation
Excision for Homotopy Groups. The Hurewicz Theorem. Fiber Bundles. Stable Homotopy Groups.
3. Connections with Cohomology
The Homotopy Construction of Cohomology. Fibrations. Postnikov Towers.
Obstruction Theory.
4. Additional Topics
Basepoints and Homotopy. The Hopf Invariant. Minimal Cell Structures.
Cohomology of Fiber Bundles. The Brown Representability Theorem. Spectra and Homology Theories. Gluing Constructions. Eckmann-Hilton Duality.
Stable Splittings of Spaces. The Loopspace of a Suspension. Symmetric Products and the Dold-Thom Theorem. Steenrod Squares and Powers. Appendix
Topology of Cell Complexes. The Compact-Open Topology.
Other references:
J. Milnor, Topology from the differentiable viewpoint
R. Bott and L. Tu, Differential forms in algebraic topology
V. Guillemin, A. Pollack, Differential topology
Note: Chapters and sections with (*) shall not be covered in the contests.
----------------------------------------------Algebra, Number Theory and Combinatorics (preliminary draft)
Linear algebra
Linear algebra. Matrices, linear transformations, change of basis; nullity-rank theorem. Eigenvalues and eigenvectors; determinants, characteristic and minimal polynomials, Cayley-Hamilton theorem; diagonalization and triangularization of operators; Jordan normal form, rational canonical form; invariant subspaces and canonical forms; inner product spaces, hermitian and unitary operators, adjoints. Multilinear algebra, bilinear forms, orthogonal groups, spectral theorem (real and complex cases).
Texts:
Strang, Linear algebra, Academic Press.
Noble-Daniel, Applied linear algebra, Prentice-Hall;
Hoffman-Kunze, Linear algebra, Prentice-Hall
Algebra
I. GROUP THEORY. Groups, subgroups, normal subgroups, homomorphisms, quotient groups, automorphisms, groups acting on sets, Sylow theorems and applications, finitely generated abelian
groups. Examples: permutation groups, cyclic groups, dihedral groups, matrix groups, simple groups, Jordan-Holder theorem, linear groups (GL(n, F) and its subgroups), p-groups, solvable and nilpotent groups, group extensions, semi-direct products, free groups, amalgamated products and group presentations.
II. COMMUTA TIVE RINGS. Basic properties of rings, units, ideals, homomorphisms, quotient rings, prime and maximal ideals, fields of fractions, Euclidean domains, principal ideal domains and unique factorization domains, polynomial and power series rings, Chinese Remainder Theorem, local rings and localization, Nakayama's lemma, Noetherian rings, Hilbert basis theorem, Artin rings, integral ring extensions, Nullstellensatz, Dedekind domains(*),algebraic sets(*), Spec(A)(*).
III. MODULES. Finitely generated modules over PID's and applications, chain conditions (especially Noetherian modules), projective and injective modules, tensor products, sym-
metric and exterior powers, Hom , Tor and Ext(*) .
IV. FIELD THEORY. Degree of an extension, algebraic and transcendental field extensions, splitting fields and algebraic closure, finite fields, normal and separable extensions, theorem of the primitive element, inseparable extensions, field embeddings and automorphisms, Galois theory, norms and traces, cyclic extensions, Galois theory of number fields, transcendence degree, function fields.
V. GROUP REPRESENTA TIONS and Non-commutative rings. Irreducible representations, Schur's lemma, characters, Schur orthogonality, character tables, semisimple group rings, induced representations, Frobenius reciprocity, tensor products, symmetric and exterior powers, complex, real, and rational representations, division rings(*), matrix rings(*), semisimple rings and modules(*).
References:
Jacobson, Nathan Basic algebra. I. Second edition. W. H. Freeman and Company, New York, 1985. xviii+499 pp.
Jacobson, Nathan Basic algebra. II. Second edition. W. H. Freeman and Company, New York, 1989. xviii+686 pp.
Dummit and Foote: Abstract Algebra
S. Lang, Algebra, Addison-Wesley;
M. Artin, Algebra
J. P. Serre, Linear representations of finite groups.
冯克勤，李尚志，查建国，章璞，《近世代数引论》
刘绍学，《近世代数基础》
Elementary Number Theory
1. Integers: a) Divisibility of integers, Euc lidean algorithm, unique dec omposition theorem of integers; b) c ongruenc e and c ongruenc e c lasses, solving c ongruenc e
equations, the Chinese Remainder theorem; c) Prime roots and indexes; d) Quadratic reciprocity ; e)Indeterminate Equations.
2. Polynomials：a) Polynomials of one variable, Euc lidean algorithm, uniqueness dec omposition theorem, zeros; b) The fundamental theorem of algebra, unit roots; c) Polynomials of integer coeffic ients, the Gauss lemma and the Eisenstein c riterion; d) Polynomials of several variables, homogenous and symmetric polynomials, the fundamental theorem of symmetric polynomials.
References:
Hardy, G. H.; Wright, E. M. An introduction to the theory of numbers. Sixth edition. Revised by D. R. Heath-Brown and J. H. Silverman. Oxford University Press, Oxford, 2008. xxii+621 pp.
Borevich, A. I.; Shafarevich, I. R. Number theory. Translated from the Russian by Newcomb Greenleaf. Pure and Applied Mathematics, V ol. 20 Academic Press, New Y ork-London 1966 x+435 pp.
J. P. Serre, A course in arithmetics.
《整数与多项式》冯克勤余红兵著高等教育出版社
Combinato rics (*)
van Lint, J. H.(NL-EIND); Wilson, R. M.(1-CAIT) A course in combinatorics. (English summary) Cambridge University Press, Cambridge, 1992. xii+530 pp.
Table of Contents
Preface
1. Graphs
2. Trees
3. Colorings of graphs and Ramsey's theorem
4. Tur a n's theorem and extremal graphs
5. Systems of distinct representatives
6. Dilworth's theorem and extremal set theory
7. Flows in networks
8. De Bruijn sequences
9. The addressing problem for graphs
10. The principle of inclusion and exclusion inversion formulae
11. Permanents
12. The V an der Waerden conjecture
13. Elementary counting Stirling numbers
14. Recursions and generating functions
15. Partitions
16. (0,1)-matrices
17. Latin squares
18. Hadamard matrices, Reed-Muller codes
19. Designs
20. Codes and designs
21. Strongly regular graphs and partial geometries
22. Orthogonal Latin squares
23. Projective and combinatorial geometries
24. Gaussian numbers and q-analogues
25. Lattices and Mobius inversion
26. Combinatorial designs and projective geometries
27. Difference sets and automorphisms
28. Difference sets and the group ring
29. Codes and symmetric designs
30. Association schemes
31. Algebraic graph theory: eigenvalue techniques
32. Graphs: planarity and duality
33. Graphs: colorings and embeddings
34. Electrical networks and squared squares
35. P o lya theory of counting
36. Baranyai's theorem
Note: Chapters and sections with (*) shall not be covered in the contests.
Analysis and differential equations (preliminary draft) Mathematical analysis (including ODE)
1. Basic Calculus. Basic first- and second-year calculus. Derivatives of maps from Rm to Rn, gradient, chain rule; maxima and minima, Lagrange multipliers; line and surface integrals of scalar and vector functions; Gauss’s, Green’s and Stokes’theorems. Ordinary differential equations; explicit solutions of simple equations.
2. Classical analysis. Topology of Rn and metric spaces; properties of continuous functions, compactness, connectedness, limit points; least upper bound property of R. Sequences and series, Cauchy sequences, uniform convergence and its relation to derivatives and integrals; power series, radius of convergence, Weierstrass M-test; convergence of improper integrals. Compactness in functions spaces. Inverse and implicit function theorems and applications; the derivative as a linear map; existence and uniqueness theorems for solutions of ordinary differential equations; elementary Fourier series.
References:
Rudin, Principles of mathematical analysis, McGraw-Hill.
Courant, Richard; John, Fritz Introduction to calculus and analysis. V ol. I. Reprint of the 1989 edition.Classics in Mathematics. Springer-V erlag, Berlin, 1999. xxiv+661 pp.
Courant, Richard; John, Fritz Introduction to calculus and analysis. V ol. II. With the assistance of Albert A. Blank and Alan Solomon. Reprint of the 1974 edition. Springer-V erlag, New Y ork, 1989. xxvi+954 pp.
Hardy, G. H.; Littlewood, J. E.; Pólya, G. Inequalities. Reprint of the 1952 edition. Cambridge Mathematical Library. Cambridge University Press, Cambridge, 1988. xii+324 pp.
Pólya, George; Szeg?, Gabor Problems and theorems in analysis. I. Series, integral calculus, theory of functions. II. Theory of functions, zeros, polynomials, determinants, number theory, geometry. Translated from the German by C. E. Billigheimer. Reprint of the 1976 English translation. Classics in Mathematics. Springer-V erlag, Berlin, 1998.
Coddington, Earl A.; Levinson, Norman Theory of ordinary differential equations. McGraw-Hill Book Company, Inc., New Y ork-Toronto-London, 1955. xii+429 pp.
V. I. Arnold, Ordinary Differential Equations, Springer-Verlag, Berlin, 2006.
Real analysis and functional analysis
Set Theory.
Countable and uncountable sets, the axiom of choice, Zorn's lemma.
Metric spaces. Completeness; separability; compactness; Baire category; uniform continuity; connectedness; continuous mappings of compact spaces.
Functions on topological spaces. Equicontinuity and Ascoli's theorem; the
Stone-Weierstrass theorem; topologies on function spaces; compactness in function spaces.
Measure.
Measures and outer measures; measurability and _-algebras; Borel sets;
extension of measures; Lebesgue and Lebesgue-Stieltjes measures; signed measures; absolute continuity and singularity; product measures.
Measurable functions. Properties of measurable functions; approximation by
simple functions and by continuous functions; convergence in measure; Egoro v's theorem; Lusin's theorem; Jensen's inequality.
Integration.
Construction and properties of the integral; convergence theorems;
Radon-Nykodym theorem; Fubini's theorem; mean convergence.
Special properties of functions on the real line. Monotone functions; functions
of bounded variation and Borel measures; absolute continuity; differentiation
and integration; convex functions; semicontinuity; Borel sets; properties of the Cantor set.
Elementary properties of Banach and Hilbert spaces.
Lp spaces; C(X); completeness and the Riesz-Fischer theorem; orthonormal bases; linear functionals; Riesz representation theorem; linear transformations and dual spaces; interpolation
of linear operators; Hahn-Banach theorem; open mapping theorem; uniform boundedness
(or Banach-Steinhaus) theorem; closed graph theorem.
Basic harmonic analysis.
Basic properties of Fourier series and the Fourier transform; Poission summation formula; convolution.
Compact operators
Basic properties of compact operators, Riesz- Fredholm theory, spectrum of compact operators.
References:
Royden, Real Analysis, except chapters 8, 13, 15.
E.M. Stein and R. Shakarchi，Real Analysis: Measure Theory, Integration, and Hilbert Spaces, Princeton University Press, 2005
周民强, 实变函数论, 北京大学出版社, 2001
夏道行等，《实变函数论与泛函分析》，人民教育出版社.
Peter D. Lax, Functional Analysis, Wiley-Interscience, 2002.
吉田耕作, 泛函分析.
Complex analysis
An Introduction to the Theory of Analytic Functions of
One Complex V ariable
By Lars V alerian Ahlfors, McGraw-Hill College, August 1979
Preface
Chapter 1: Complex numbers
1. The algebra of complex numbers
2. The geometric Representation of Complex Numbers Chapter 2: Complex functions
1. Introduction to the concept of Analytic function
2. Elementary Theory of Power Series
3. The exponential and trigonometric Functions Chapter 3: Analytic functions as mappings
1. Elementary Point Set Topology
2. Conformality
3. Linear Transformations
4. Elementary Conformal Mappings
Chapter 4: Complex Integration
1. Fundamental Theorems
2. Cauchy's Integral Formula
3. Local Properties of Analytic Functions
4. The General Form of Cauchy's Theorem
5. The Calculus of Residues
6. Harmonic Functions
Chapter 5: Series and Product Developments
1. Power Series Expansions
2. Partial Fractions and Factorization
3. Entire Functions
4. Normal Families
Chapter 6: Conformal Mapping, Dirichlet’s Problem
1. The Riemann Mapping Theorem
2. Conformal Mapping of Polygons
3. A closer Look at Harmonic Functions
4. The Dirichlet Problem
5. Canonical Mappings of Multiply Connected Regions
Chapter 7: Elliptic Functions
1. Simply Periodic Functions
2. Doubly Periodic Functions
3. The Weierstrass Theory
Chapter 8: Global Analytic functions
1. Analytic Continuation
2. Algebraic Functions
3. Picard's Theorem
4. Linear Differential Equations
Other references:
K. Kodaira, Complex Analysis
Rudin, Real and complex analysis
龚升，简明复分析
Basic partial differential equations
First order partial differential equations, linear and quasi-linear PDE
Wave equations: initial condition and boundary condition, well-poseness, Sturn-Liouville eigen-value problem, energy functional method, uniqueness and stability of solutions
Heat equations: initial conditions, maximal principle and uniqueness and stability Potential equations: Green functions and existence of solutions of Dirichlet problem, harmonic functions, Hopf’s maximal principle and existence of solutions of Neumann’s problem, weak solutions, eigen-value problem of the Laplace operator
Generalized functions and fundamental solutions of PDE
References:
《Basic Partial Differential Equations》, D. Bleecker, G. Csordas 著, 李俊杰译，高等教育出版社，2008.
《数学物理方法》，柯朗、希尔伯特著。
Gilbarg and Trudinger, Elliptic Partial Differential Equations of Second Order, Chapter 7.
Computational Mathematics, Applied Mathematics,
Probability and Statistics (preliminary draft) Computational Mathematics
1. Interpolation and approximation
--- polynomial interpolation and least square approximation
--- trigonometric interpolation and approximation,
fast Fourier transform
--- approximations by rational functions
--- splines
2. Nonlinear equation solvers
--- convergence of iterative methods (bisection,
secant method, Newton method, other iterative
methods) for both scalar equations and systems
--- finding roots of polynomials
3. Linear systems and eigenvalue problems
--- direct solvers (Gauss elimination, LU decomposition,
pivoting, operation count, banded matrices,
round-off error accumulation)
--- iterative solvers (Jacobi, Gauss-Seidel, successive
over-relaxation, conjugate gradient method, multi-grid method, Krylov methods)
--- numerical solutions for eigenvalues and eigenvectors
4. Numerical solutions of ordinary differential equations
--- one step methods (Taylor series method and Runge-Kutta method)
--- stability, accuracy and convergence
--- absolute stability, long time behavior
--- multi-step methods
5. Numerical solutions of partial differential equations
--- finite difference method
--- stability, accuracy and convergence, Lax equivalence theorem
--- finite element method, boundary value problems
References:
[1] C. de Boor and S.D. Conte,
Elementary Numerical Analysis, an algorithmic approach, McGraw-Hill, 2000.
[2] G.H. Golub and C.F. van Loan,
Matrix Computations, third edition, Johns Hopkins University Press, 1996.
[3] E. Hairer, P. Syvert and G. Wanner,
Solving Ordinary Differential Equations, Springer, 1993.
[4] B. Gustafsson, H.-O. Kreiss and J. Oliger,
Time Dependent Problems and Difference Methods, John Wiley Sons, 1995.
[5] G. Strang and G. Fix,
An Analysis of the Finite Element Method, second edition, Wellesley-Cambridge Press, 2008.
Probability
Introduction to Probability Models
by Sheldon M. Ross
1 Introduction to Probability Theory
2 Random V ariables
3 Conditional Probability and Conditional Expectation
4 Markov Chains
5 The Exponential Distribution and the Poisson Process
6 Continuous-Time Markov Chains
7 Renewal Theory and Its Applications
8 Queueing Theory
9 Reliability Theory
10 Brownian Motion and Stationary Processes
11 Simulation
Statistics
Overview
The statistics part contains seven areas: (1) distribution theory, (2) likelihood principle, (3) testing, (4) estimation, (5) Bayesian statistics, (6) nonparametric statistics, (7) regression and (8) large sample theory.
1. Distribution Theory and Basic Statistics
Families of continuous distributions: Chi-sq, t, F, gamma, beta
Families of discrete distributions: Multinomial, Poisson, negative binomial
Basic statistics: Mean, median, quantiles, order statistics
2. Likelihood principle
Likelihood function, parametric inference, sufficiency, factorization theorem, ancillary statistic, conditional likelihood, marginal likelihood.
3. Testing
Neyman-Pearson paradigm, null and alternative hypotheses, simple and composite hypotheses, type I and type II errors, power, most powerful test, likelihood ratio test, Neyman-Pearson Theorem, monotone likelihood ratio, uniformly most powerful test, generalized likelihood ratio test.
4. Estimation
Parameter estimation, method of moments, maximum likelihood estimation, unbiasedness, quadratic and other criterion functions, Rao-Blackwell Theorem, Fisher information, Cramer-Rao bound, confidence interval, duality between confidence interval and hypothesis testing.
5. Bayesian Statistics
Prior, posterior, conjugate priors, Bayesian loss
6. Nonparametric statistics
Permutation test, permutation distribution, rank statistics, Wilcoxon-Mann-Whitney test, log-rank test, Kolmogorov-Smirnov-type tests.
7. Regression
Linear regression, least squares method, Gauss-Markov Theorem, logistic regression, maximum likelihood
8. Large sample theory
Consistency, asymptotic normality, chi-sq approximation to likelihood ratio statistic, large-sample based confidence interval, asymptotic properties of empirical distribution.
References
Casella, G. and Berger, R.L. (2002). Statistical Inference (2nd Ed.) Duxbury Press.
茆诗松，程依明，濮晓龙，概率论与数理统计教程（第二版），高等教育出版社，2008.
陈家鼎，孙山泽，李东风，刘力平，数理统计学讲义，高等教育出版社，2006.
郑明，陈子毅，汪嘉冈，数理统计讲义，复旦大学出版社，2006.
陈希孺，倪国熙，数理统计学教程，中国科学技术大学出版社，2009.
Applied Mathematics
https://www.doczj.com/doc/0d10392493.html,place method
2.Stationary phase method
3.Regular perturbation method
4.Singular perturbation method
5.Matched asymptotic method
6.Two time scale asymptotic expansions for ODEs
7.V ariational methods
8.Method of averaging for ODEs
9.Homogenization method for elliptic PDEs
10.Homogenization method for linear hyperbolic PDEs
11.Homogenization and front propagation of Hamilton-Jacobi equations
12.Front propagation of reaction-diffusion-advection equations in
heterogeneous media.
13.Geometric optics for dispersive wave equations
14.Slowly varying amplitude expansions for dispersive PDEs (sine-Gordon,
nonlinear Schroedinger)
Reference Books:
1. N. De Bruijn, “Asymptotic Methods in Analysis”, Dover, NY, 1981.
2. J. Kervorkian, J. Cole, “Perturbation Methods in Applied Mathematics”, Applied Math Sciences, 34, Springer, 1981.
3. G. Whitham, “Linear and Nonlinear Waves”, John-Wiley and Sons, 197
4.
4. J. Keener, “Principles of Applied Mathematics”, Addison-Wesley, 1988.
5. A. Benssousan, P-L Lions, G. Papanicolaou, “Asymptotic Analysis for Periodic Structures”, North-Holland Publishing Co, 1978.
6. L.C. Evans, “Weak convergence methods for nonlinear PDEs”, NSF-CBMS regional conference notes, 1988.
7. V. Jikov, S. Kozlov, O. Oleinik, “Homogenization of differential operators and integral functions”, Springer, 1994.
8. J. Xin, “An Introduction to Fronts in Random Media”, Surveys and Tutorials in Applied Math Sciences, No. 5, Springer, 2009.
2017年度学科竞赛奖、科技竞赛奖评选方案 一、评选原则 1、学科竞赛奖、科技竞赛奖均为了鼓励广大同学通过积极参与各类赛事，提高自身素质，为校争光。 2、学科竞赛奖分为三个等级，一等奖3000元/人，二等奖2000元/人，三等奖1000元/人；科技竞赛奖分为三个等级，一等奖3000元/人，二等奖2000元/人，三等奖1000元/人。各个等级获奖人数按照当年参与竞赛的学生人数与获得奖励的质量确定。总获奖人数不超过总参赛人数的60%，总奖励金额均不超过20万元。 3、不能出现下列现象： A、某同学获得多个不同低赛事、低等级的竞赛奖，累加后得分相对较高，甚至高于某单个高赛事的得分。 B、某团队的成员在团队贡献上相对较低，但其单项得分与其他成员相同。 C、某团队的同一作品获得同一类型赛事奖励，在计算得分时出现累加情况。 D、非在校本科生参与评选。 二、各指标分值确定 1、影响因子 影响因子的确定两个部门比较一致，它与竞赛的名称有关，最高值为5分，以分为刻度。以下为各个竞赛的影响因子：
2、等级分值 等级分值与竞赛级别和获奖等级均有关，最高值为100分，以
10分为刻度。以下为各个等级的分值： 3、作者排序 作者排序只与团队参与竞赛的作者排序有关，如提供作者排序的奖项，第一作者值为1，以后按照的分值递减，即第二作者值为，第三为……
4、附加 获得不同竞赛奖励的同学，在累加得分时，减去附加值，附加值计算为：50*（获得竞赛奖励次数-1），如某同学获得3项奖励，则附加值为50*（3-1）=100。（因为竞赛最低得分不低于50分，故以50定为因子，若有竞赛得分低于50分，则直接取其竞赛项目最高分为最终得分）。 根据评选原则，为避免同一赛事相互累加，同一名同学在同一时间同一赛事不同项目获奖或者获得多个奖项，取该名同学在该赛事中获得的最高级奖项计算。
第二届(2010年)全国大学生数学竞赛预赛试卷及参考答案 （非数学类） (150分钟) 一、（25分，每小题5分） （1）设22(1)(1)(1),n n x a a a =+++ 其中||1,a <求lim .n n x →∞ （2）求2 1lim 1x x x e x -→∞??+ ???。 （3）设0s >，求0(1,2,)sx n I e x dx n ∞ -==? 。 （4）设函数()f t 有二阶连续导数，1(,)r g x y f r ??== ???，求2222g g x y ??+??。 （5）求直线10:0 x y l z -=??=?与直线2213:421x y z l ---==--的距离。 解：（1）22(1)(1)(1)n n x a a a =+++ =22(1)(1)(1)(1)/(1)n n x a a a a a =-+++- =222(1)(1)(1)/(1)n a a a a -++- = =1 2(1)/(1)n a a +-- 1 2lim lim(1)/(1)1/(1)n n n n x a a a +→∞→∞=--=-∴ (2) 22211ln (1)ln(1)1lim 1lim lim x x x e x x x x x x x x e e e x -++--→∞→∞→∞??+== ??? 令x=1/t,则 原式=1(ln(1)) 1/(1)112(1)220 00lim lim lim t t t t t t t t t e e e e +-+---+→→→=== （3）0000112021011()()[|](1)!!sx n n sx n sx sx n n sx n n n n n I e x dx x de x e e dx s s n n n n n n e x dx I I I s s s s s ∞∞∞---∞-∞----+==-=--=-=====???? （4）略（不难，难得写） （5 二、（15分）设函数()f x 在(,)-∞+∞上具有二阶导数，并且 ()0,lim ()0,lim ()0,x x f x f x f x αβ→+∞→-∞ ''''>=>=<且存在一点0x ，使得0()0f x <。
1 x ? ? ? ? a ? 第四届全国大学生数学竞赛决赛试题标准答案 一、(本题15分): 设A 为正常数，直线?与双曲线x 2 ? y 2 = 2 (x > 0) 所围的有 限部分的面积为A . 证明： (i) 所有上述?与双曲线x 2 ? y 2 = 2 (x > 0) 的截线段的中点的轨迹为双曲线. (ii)?总是(i)中轨迹曲线的切线. 证明：将双曲线图形进行45度旋转，可以假定双曲线方程为y = 1 , x > 0. 设 直线?交双曲线于(a, 1/a )和(ta, 1/ta ), t > 1, 与双曲线所围的面积为A . 则有 1 1 ∫ ta 1 1 1 1 1 A = 2 (1 + t )(t ? 1) ? dx = + )(t 1) log t = t ) log t. x 2 t 2 t 令f (t ) = 1 (t ? 1 ) ? log t . 由于 2 t 1 1 2 f (1) = 0, f (+∞) = +∞, f ′ (t ) = 2 (1 ? t ) > 0, (t > 1), 所以对常数A 存在唯一常数t 使得A = f (t ) (5分). ?与双曲线的截线段中点 坐标 为 1 1 1 1 x = 2 (1 + t )a, y = 2 (1 + t ) a . 于是，中点的轨迹曲线为 1 1 xy = 4 (1 + t )(1 + t ). (10分) 故中点轨迹为双曲线, 也就是函数y = 1 (1 + t )(1 + 1 ) 1 给出的曲线. 该 曲线在上述中点处的切线斜率 4 t x 1 1 1 1 k = ? 4 (1 + t )(1 + t ) x 2 = ? ta 2 , 它恰等于过两交点(a, 1/a )和(ta, 1/ta )直线?的斜率: 1 1 1 故?为轨迹曲线的切线. (15分) ta ? a ta ? a = . 二、(本题15分): 设函数f (x )满足条件: 1) ?∞ < a ≤ f (x ) ≤ b < +∞, a ≤ x ≤ b ; 2) 对于任意不同的x, y ∈ [a, b ]有|f (x ) ? f (y )| < L |x ? y |, 其中L 是大
丘成桐中学数学奖手册 引言 数学科学在当今国际科技和人才竞争力方面具有突出的重要地位，在与人类日常生活有关的科学技术中的应用也日趋广泛。我们相信，为了更好地适应未来社会的挑战，青少年应该拥有良好的数学教育。国际上很早就倡导尽可能早地培养学生的科研创新能力，并为此设奖鼓励更多的人参与进来。比如在美国，与数学有关的面向高中生科研成果的“西屋科技奖”（），这个奖项不同于普通的数学竞赛，而是注重创新与实践，鼓励团队精神，极大地促进了美国高中生、大学生的科研热情，许多获奖者后来都成为著名的科学家。据统计，“西屋科技奖”（现更名为“英特尔科技奖”）得主中有位后来成为诺贝尔科学奖获得者，人当选为美国科学院院士。 背景 任何科技发展都不能缺乏数学作为根基，数学在科技年代，地位日益重要。为鼓励华人数学研究和教育发展，激发全球华人青少年对数学的兴趣，并及早发掘与培养全世界的华人数学英才，国际数学大师丘成桐教授提出举办一个中学生数学比赛，希望通过专题研究，培养新一代中学生的数学素养，引发青年人探索知识的兴趣及提升他们的创新能力。 泰康人寿保险股份有限公司董事长陈东升先生对丘成桐教授的想法给予全面的赞赏和大力的支持。丘成桐教授与陈东升先生在北京、杭州多次会面商谈设立中学生数学奖的具体事宜，最终决定由双方协作配合，联合设立“丘成桐中学数学奖”。 泰康人寿保险股份有限公司多年来一直将“回馈社会，奉献爱心”作为企业发展的准则，积极组织、发起和参与了多项社会公益活动，曾荣获“搜狐新视角高峰论坛”颁发的“最佳社会公益奖”。 “丘成桐中学数学奖”将借鉴和采用“西屋科技奖”的组织与选拔模式，强调创新与团队精神，面向全球的华人中学生。我们将通过全国主流新闻媒体向社会广泛宣传报道，并努力将“丘成桐中学数学奖”打造成为拥有国际知名度与良好社会效应的青少年科技奖项。 年月日，“丘成桐中学数学奖”签约仪式暨新闻发布会于第四届华人数学家大会期间的在杭州举行；年月26日，将在北京举办隆重的启动仪式。第一届“丘成桐中学数学奖”颁奖仪式定于200年月日在北京举行，美国哈佛大学、布朗大学等名校的本科招生主任将会出席，并面试部分获奖学生。 主旨 ?激发全球华人中学生对于数学研究的兴趣和创造力； ?发现和培养有前途的年轻数学天才；
丘成桐：把精力放奥数上很荒谬 2011年11月11日11:15 来源：光明日报作者：丘成桐 0人参与0条评论打印转发 丘成桐：把精力放奥数上很荒谬（图片来源：光明日报）丘成桐(清华大学数学系教授) 吴正宪(北京市教科院小学数学教学研究室主任) 似乎是从上世纪八九十年代开始,“奥数班”的星星之火开始点燃在城市的每一个角落。二十年后的今天,如果你问一个学龄儿童,他们十之八九接触过奥数或正在“奥数班”苦读。这其中,诚然有热爱数学的孩子,但大多数却只是为了升学的“权宜之计”。 “全民奥数”有没有必要?讨论与反思一直持续,奥数却越发成为一道解不开的“魔咒”。有人说,“奥数锻炼思维,培养创新能力”,也有人说,“奥数是数学杂技、数学八股”。 奥数到底是一门什么样的学问?我们应该怎样对待它? 要调动学生的兴趣,而不是扼杀它,有兴趣才能学好数学 记者:数学是不少学科的基础,学好数学的关键是什么?
丘成桐:我们国家研究数学有几千年的历史了,没有哪个人是因为偶尔的灵光一现就成为数学家的。如果没有走过前人的路,没有坚实的基础,不可能成为数学家。 我认为学好数学应重视基础,但并不是说否认创新,否认考试。考试是很重要的,不考试不能自知。我在中学时也考年考,但是题目很基础,不是挖空心思难倒学生的。很多学者成才都是从中学开始的,老师要懂得调动学生的兴趣,有兴趣才能学好数学。 我们在清华大学举办以陈省身等四个数学家命名的数学讲座目的就在于吸引学生的兴趣。由于他们的影响力,我邀请到很多国际知名的数学家,他们愿意来到清华,以延续大师之风。学生们也因此晓得真正的前沿学者在做什么,他们怎么做事。我想,让他们对数学感兴趣,这是最有效的学习方法。 吴正宪:学好数学首先要激发学生学习的兴趣和求知欲望,保护好奇心。孔子说过:“知之者不如好之者,好之者不如乐之者。”“好”与“乐”就是愿意学、喜欢学,就是学习兴趣,这是学好数学的重要前提。 其次,教师要注重引导学生积累数学学习经验,感悟数学思想,培养学生良好的学习方法。学生在观察、猜测、尝试、验证、推理与交流的数学活动中,经历“数学化”的学习过程,积累数学活动经验,获得数学思想和方法。在运用数学知识的过程中掌握解决问题的方法和策略,这是学好数学的重要途径。 以学医来说,奥数就像疑难杂症,如果不扎实打好基础,只攻疑难杂症,到最后可能连普通的感冒都不会治 记者:奥数到底是什么,它是否等同于数学?学好奥数又意味着什么? 丘成桐:据我看来,奥数不少题目很刁钻,作为爱好偶一为之是可以的。如果作为主业精心揣摩,甚至为了应付升学,,则是很荒谬的事。 打个比方,以学医来说,奥数就像疑难杂症,如果不扎实打好基础,只攻疑难杂症,到最后可能连普通的感冒都不会治。这能说是合格的医生吗?这样子学,学懂了无异于没学懂。 吴正宪:“奥数”是什么?一两句话很难说清,大概在学生家长的心中“奥数”就是要学习比日常数学课堂中要难得多的数学题。它似乎源于数学教材,又远远高于教材,它是作为数学学习中比较有难度的一部分。 “奥数”所涉及的内容广泛、难度大,小学生做的“奥数题”甚至大人们都很费解。解决这类问题,一般都需要对实际问题的数学意义进行分析、归纳,把实际问题抽象成为数学问题,然后用相应的数学知识和方法去解决。教学过程中如果能处理好此类问题,就可以培养学生用数学观点看待和处理实际问题的能力,提高学生用数学语言和模型解决实际问题的意识和能力。它可以开拓学生思路,启迪学生思维,提高知识的运用能力。
全国大学生数学竞赛预赛试卷（非数学类） 2009年 第一届全国大学生数学竞赛预赛试卷（非数学类） 一、填空题（每小题5分，共20分） 1． 计算()ln(1) d y x y x y ++=??，其中区域D 由直线1=+y x 与两坐标轴所围成三角形区域. 2．设)(x f 是连续函数，且满足22 ()3()d 2f x x f x x =--? ，则()f x =. 3．曲面2 222 x z y =+-平行平面022=-+z y x 的切平面方程是. 4．设函数)(x y y =由方程29ln )(y y f e xe =确定，其中f 具有二阶导数，且 1≠'f ，则=22d d x y . 二、（5分）求极限x e nx x x x n e e e )(lim 20+++→Λ，其中n 是给定的正整数. 三、（15分）设函数)(x f 连续，10()() g x f xt dt =?，且A x x f x =→) (lim 0，A 为常数，求()g x '并讨论)(x g '在0=x 处的连续性. 四、（15分）已知平面区域}0,0|),{(ππ≤≤≤≤=y x y x D ，L 为D 的正向边界，试证： （1）??-=---L x y L x y x ye y xe x ye y xe d d d d sin sin sin sin ； （2）2sin sin 2 5d d π?≥--L y y x ye y xe . 五、（10分）已知x x e xe y 21+=，x x e xe y -+=2，x x x e e xe y --+=23是某二阶常系数线性非齐次微分方程的三个解，试求此微分方程. 六、（10分）设抛物线c bx ax y ln 22++=过原点.当10≤≤x 时，0≥y ，又已知该抛物线与x 轴及直线1=x 所围图形的面积为3 1.试确定 c b a ,,，使此图形绕x 轴旋转一周而成的旋转体的体积V 最小. 七、（15分）已知)(x u n 满足1()()1,2,n x n n u x u x x e n -'=+=L ，且n e u n =)1(，求 函数项级数∑∞ =1 )(n n x u 之和.
大学生学科竞赛种类调研明细目录： 全国大学生数学建模竞赛 5 美国大学生数学建模竞赛（MCM/ICM） 6 全国大学生数学竞赛 6 丘成桐大学生数学竞赛 7 国际大学生物理竞赛 7 中国大学生物理学术竞赛（CUPT） 8 南京大学青年物理学家锦标赛（NYPT） 9 国际全局轨道优化竞赛 9 全国深空轨道设计竞赛 10 全国大学生英语竞赛 10 国家大学生创新性实验计划 11 挑战杯系列赛事 13 大学生学术科技作品展 15 基础学科论坛 15 学生学科竞赛项目一览表：
全国大学生数学建模竞赛 主办： 教育部高等教育司中国工业与应用数学学会(CSIAM) 校管理部门： 教务处 校承办单位： 数学系 竞赛时间： 每年9月 竞赛简介： 数模竞赛是由美国工业与应用数学学会在1985年发起的一项大学生竞赛活动。我国大学生数学建模竞赛是面向全国高等院校的、每年一届的通讯竞赛。其宗
旨是：创新意识、团队精神、重在参与、公平竞争。1992载在中国创办，自从创办以来，得到了教育部高教司和中国工业与应用数学协会的得力支持和关心，呈现出迅速的发展势头。 竞赛内容一般来源于工程技术和管理科学等方面经过适当简化加工的实际问题，不要求参赛者预先掌握深入的专门知识，只需要学过普通高校的数学课程。题目有较大的灵活性供参赛者发挥其创造能力。参赛者应根据题目要求，完成一篇包括模型的假设、建立和求解、计算方法的设计和计算机实现、结果的分析和检验、模型的改进等方面的论文（即答卷）。竞赛评奖以假设的合理性、建模的创造性、结果的正确性和文字表述的清晰程度为主要标准。 竞赛形式为全国统一竞赛题目，采取通讯竞赛方式，以相对集中的形式进行；竞赛一般在每年9月末的三天内举行。大学生以队为单位参赛，每队3人，专业不限。研究生不得参加。每队可设一名指导教师（或教师组），从事赛前辅导和参赛的组织工作。 美国大学生数学建模竞赛（MCM/ICM） 主办： 美国数学及其应用联合会 竞赛简介： 美国大学生数学建模竞赛每年的比赛时间一般定在二月初，需要通过官方网站报名，而且需要有固定的指导教师。 竞赛简介：美国大学生数学建模竞赛（MCM/ICM），是一项国际级的竞赛项目，为现今各类数学建模竞赛之鼻祖。
S.-T.Yau College Student Mathematics Contests 2011 Analysis and Di?erential Equations Individual 2:30–5:00pm,July 9,2011 (Please select 5problems to solve) 1.a)Compute the integral: ∞?∞x cos xdx (x 2+1)(x 2+2) ,b)Show that there is a continuous function f :[0,+∞)→(?∞,+∞)such that f ≡0and f (4x )=f (2x )+f (x ). 2.Solve the following problem: d 2u dx 2 ?u (x )=4e ?x ,x ∈(0,1),u (0)=0,du dx (0)=0.3.Find an explicit conformal transformation of an open set U ={|z |>1}\(?∞,?1]to the unit disc. 4.Assume f ∈C 2[a,b ]satisfying |f (x )|≤A,|f (x )|≤B for each x ∈[a,b ]and there exists x 0∈[a,b ]such that |f (x 0)|≤D ,then |f (x )|≤2√AB +D,?x ∈[a,b ]. 5.Let C ([0,1])denote the Banach space of real valued continuous functions on [0,1]with the sup norm,and suppose that X ?C ([0,1])is a dense linear subspace.Suppose l :X →R is a linear map (not assumed to be continuous in any sense)such that l (f )≥0if f ∈X and f ≥0.Show that there is a unique Borel measure μon [0,1]such that l (f )= fdμfor all f ∈X . 6.For s ≥0,let H s (T )be the space of L 2functions f on the circle T =R /(2πZ )whose Fourier coe?cients ?f n = 2π0e ?inx f (x )dx satisfy Σ(1+n 2)s ||?f n |2<∞,with norm ||f ||2s =(2π)?1Σ(1+n 2)s |?f n |2. a.Show that for r >s ≥0,the inclusion map i :H r (T )→H s (T )is compact. b.Show that if s >1/2,then H s (T )includes continuously into C (T ),the space of continuous functions on T ,and the inclusion map is compact.1
第二届全国大学生数学竞赛（辽宁赛区）通知 根据全国大学数学竞赛委员会工作安排，第二届大学生数学竞赛分区预赛在2010年10月30日（星期六）上午9：00—11：30举行，决赛于2011年3月份的第三周周六上午在北京航空航天大学举行。 现将xx赛区竞赛的具体事宜通知如下： 一、参赛对象： 大学本科二年级或二年级以上的在校大学生。竞赛分为非数学专业组和数学专业组(含数学与应用数学、信息与计算科学专业的学生)。数学专业学生不得参加非数学专业组的竞赛。 二、竞赛内容： 非数学专业组竞赛内容为本科高等数学内容（高等数学内容为理工科本科教学大纲规定的高等数学的教学内容）。 数学专业组竞赛内容含数学分析、高等代数和解析几何（均为数学专业本科教学大纲规定的教学内容），所占比重分别为50%、35%及15%左右。 三、报名与收费 1、各个学校务必将参赛人数和参赛学生名单9月20日前用电子邮箱发给所在考点的负责人，各个考点的负责人9月25日前将本赛区的参赛名单发给韩友发（参赛名单统一按Excel格式，见附件）； 2、报名费为每人60元，由各单位于10月20日前交齐。统一汇入如下帐号（收到款后开发票）： 单位:xx师范大学 开户行：中国建设银行大连市分行，沙河口支行(辽） 四、考点设置
根据辽宁省高校的分布情况，我们将在沈阳、大连、鞍山和锦州四个城市设立考点。每个考点要统一组织考试。其他城市的学校到就近的考点参加考试。 五、阅卷工作安排 考试结束后我们将统一阅卷。 1、阅卷时间：2010年11月6—7日。 2、阅卷地点：另行通知。 3、试卷统一印刷和分发。 4、推荐阅卷教师：每参赛５０人推荐1名阅卷教师（不足５０人按５０计算）；并注明阅卷科目，同一个学校阅卷教师要分布在不同科目（分析、代数、几何、高等数学）；阅卷教师推荐名单１０月２０日前用电子邮箱发给韩友发（名单统一按Excel格式，见附件）；由竞赛委员会确定阅卷教师。 六、评奖办法详见国家通知。 xx发联系方式 电话： 邮箱： （收到此通知后务请回复） xx数学会2010年８月23日 第二届全国大学生数学竞赛辽宁赛区竞赛委员会主任： xx（东北大学） 副主任： xx（大连理工大学）
大学数学怎么学?学好大学数学的8个方法 大学数学怎么学?学好大学数学的8个方法 学好大学数学的8个方法 1)大一生大都自我感觉良好，认为自己的学习方法是成功的。自己能考上不错的本科，就说明自己在学习上有一套。自己高中怎样学，大学还怎样学，就一定能成功。不知道改进学习方法的必要性。 2)缺少迎难而上的思想准备。基础知识大滑坡，基本技能大退步，头脑时常出现空白。学习时跟不上教学的进度与要求。 3)对大学课程的学习特点，缺少全面准确的了解。对大学生应该掌握的学习方法，缺少系统的学习和掌握。 提高大学数学学习成绩的关键： 大学生学数学，靠的是一个字：悟! 借助这8个方法，教你更好领悟高数 1 先看笔记后做作业 有的学生感到，老师讲过的，自己已经听得明明白白了。但是，为什么自己一做题就困难重重了呢?其原因在于，学生对教师所讲的内容的理解，还没能达到教师所要求的层次。 因此，每天在做作业之前，一定要把课本的有关内容和当天的课堂笔记先看一看。 2 做题之后加强反思 现在正做着的题，一定不是考试的题目。而是要运用现在正做着的题目的解题思路与方法。因此，要把自己做过的每道题加以反思，总结一下自己的收获。要总结出：这是一道什么内容的题，用的是什么方法。做到知识成片，问题成串，构建起一个内容与方法的科学的网络系统。 要看看自己做对了没有;还有什么别的解法;题目处于知识体系中的什么位置;解法的本质什么;题目中的已知与所求能否互换，能否进行适当增删改进。
主动复习和总结 进行章节总结是非常重要的。 怎样做章节总结呢? ①要把课本，笔记，校期末测验试卷，都从头到尾阅读一遍。 ②把本章节的内容一分为二，一部分是基础知识，一部分是典型问题。 ③在基础知识的疏理中，要罗列出所学的所有定义，定理，法则，公式。 ④把重要的，典型的各种问题进行编队。 ⑤总结那些尚未归类的问题，作为备注进行补充说明。 4 重视改错，错不重犯 一定要重视改错工作，做到错不再犯。 5 积累资料随时整理 把课堂笔记，练习，试卷，都分门别类按时间顺序整理好。每读一次，就在上面标记出自己下次阅读时的重点内容。这样，复习资料才能越读越精，一目了然。 6 精挑慎选课外读物 大学数学考的是学生解决常规题的能力。作为一名大学生，如果还想围着自己的老师转，是不可能的，老师一般一下课就走，所以这种方法会存在着很大的局限性。因此，要想学好数学，必须打开一扇门，看看外面的世界。当然，也不要自立门户，另起炉灶。一旦脱离校内教学和自己的老师的教学体系，也必将事倍功半。 7 配合老师主动学习 大学生必须提高自己学习的主动性，随时预防挂科。
浙江省首届高等数学竞赛试题（2002.12.7） 一． 计算题(每小题5分，共30分) 1 ．求极限lim x →。 2．求积分 |1|D xy dxdy -??，11{(,)2,2}22D x y x y =≤≤≤≤。 3．设2x y x e =是方程hx y ay by ce '''++=的一个解，求常数,,,a b c h 。 4．设()f x 连续，且当1x >-时，20()[()1]2(1)x x xe f x f t dt x +=+? ，求()f x 。 5．设21 1arctan 2n n k S k ==∑，求lim n n S →∞。 6．求积分1 2121(1)x x x e dx x ++ -?。 2003年浙江省大学生高等数学竞赛试题（2003.12.6） 一．计算题 7．求20 50sin()lim x x xt dt x →?。 8．设31()sin x G x t t dt =?，求21()G x dx ?。 9．求2401x dx x ∞+?。 10． 求∑=∞→++n k n k n k n 12lim 。 浙江省大学生第三届高等数学竞赛试题 1．计算：( )()2 00cos 2lim tan 1x t x x e tdt x x x →----?。 2．计算：20cos 2004 x dx x x π ππ+-+?。
3．求函数()22,415f x y x y y =++在 (){}22,41x y x y Ω=+≤上的最大、小值。 4．计算：()3max ,D xy x d σ?? ，其中(){},11,01D x y x y =-≤≤≤≤。 5. 设()1tan 1x f x arc x -=+，求)0()(n f 。 天津市竞赛题 1.证明??+≤?+020220 21cos 1sin dx x x dx x x ππ. 2. 设函数)(x f 在闭区间]2,2[-上具有二阶导数,,1)(≤x f 且 ,4)]0([)]0([22='+f f 证明：存在一点),2,2(-∈ξ使得0)()(=''+ξξf f . 3. （1）证明：当x 充分小时,不等式422tan 0x x x ≤-≤成立. （2）设,1tan 12 k n x n k n +=∑=求.lim n x x ∞ → 4. 计算??????+-??? ??+-∞→61231e 2lim n n n n n n 。5. 设()x x x f +-=11arctan ，求()()05f 。 6. 对k 的不同取值，分别讨论方程01323=+-kx x 在区间()+∞,0内根的个数。 7. 设a ，b 均为常数且2->a ，0≠a ，问a ，b 为何值时，有 ()()??-=?? ????-+++∞ +10212d 1ln d 122x x x a x x a bx x 。 8.设121-≥a ， ,,,n ,a a n n 321121=+=+，证明：n n a ∞ →lim 存在并求其值。 9.设()x f 是区间[]2+a,a 上的函数，且()1≤x f ，()1≤''x f ，证明：()2≤'x f ，[]2+∈a,a x 。 北京市竞赛试题(2008、2007、2006) .______,111,1.11 =-+++-→-m x x x m x m 则的等价无穷小是时设当 .________)1(,) ()2)(1()()2)(1()(.2='+++---=f n x x x n x x x x f 则设
第十九届北京市大学生数学竞赛本科甲、乙组试题解答 一、填空题（每小题3分，共30分） 1. ?? ????+-+-+∞→1)2(lim 61 23x e x x x x x = 1/6 . 2．设)(x f 连续，在1=x 处可导，且满足 ,0,)(8)sin 1(3)sin 1(→+=--+x x o x x f x f 则曲线)(x f y =在1=x 处的切线方程为 y =2x －2 . 3. 设243),(lim 2 20 =+-+→→y x y x y x f y x , 则 ='+')0,0()0,0(2y x f f －2 . 4．设函数()u ?可导且(0)1?=，二元函数()xy z x y e ?=+满足 0z z x y ??+=??，则()u ?=2 4u e - . 5. 设D 是由曲线x y sin = )22(π≤≤π- x 和直线2 π -=x , 1=y 所围成的区域, f 是连续函数, 则=++=??D dxdy y x f y x I )](1[223 －2 . 6. 123ln 1ln 1ln 1ln 1lim 123n n n n n n n n n n n n n n n →+∞??????????++++ ? ? ? ? ????????? ?++++= ?++++ ??? L 2ln 21- . 7. 数项级数 ∑∞ =--1 )! 2()! 2()1(n n n n n n 的和=S －1+cos1+ln2. 8. 计算积分???++π= 1 021 01 0)](6[cos dz z y x dy dx I = 1/2 . 9. 已知入射光线的路径为23 1 41-=-=-z y x , 则此光线经过平面01752=+++z y x 反射后的反射线 方程为 4 1537-= +=+z y x . 10. 设曲线2 2 2 :a y xy x C =++的长度为L , 则=++?C y x y x ds e e e b e a )sin()sin() sin()sin(L b a 2 + . 二、(10分) 设()f x 在[,)a +∞上二阶可导，且,0)(,0)(<'>a f a f 而当a x >时, ,0)(≤''x f 证明在(,)a +∞内，方程()0f x =有且仅有一个实根． 证明 由于当x a >时，,0)(≤''x f 因此'()f x 单调减，从而'()'()0f x f a ≤<，于是又有()f x 严格单调减．再由()0f a >知，()f x 最多只有一个实根． 下面证明()0f x =必有一实根．当x a >时，
Algebra,Number Theory and Combinatorics(second draft) Linear Algebra Abstract vector spaces;subspaces;dimension;matrices and linear transformations;matrix algebras and groups;determinants and traces;eigenvectors and eigenvalues,characteristic and minimal polynomials;diagonalization and triangularization of operators;invariant subspaces and canonical forms;inner products and orthogonal bases;reduction of quadratic forms; hermitian and unitary operators,bilinear forms;dual spaces;adjoints.tensor products and tensor algebras; Integers and polynomials Integers,Euclidean algorithm,unique decomposition;congruence and the Chinese Remainder theorem;Quadratic reciprocity;Indeterminate Equations.Polynomials,Euclidean algorithm, uniqueness decomposition,zeros;The fundamental theorem of algebra;Polynomials of integer coefficients,the Gauss lemma and the Eisenstein criterion;Polynomials of several variables, homogenous and symmetric polynomials,the fundamental theorem of symmetric polynomials. Group Groups and homomorphisms,Sylow theorem,finitely generated abelian groups.Examples: permutation groups,cyclic groups,dihedral groups,matrix groups,simple groups,Jordan-Holder theorem,linear groups(GL(n,F)and its subgroups),p-groups,solvable and nilpotent groups, group extensions,semi-direct products,free groups,amalgamated products and group presentations. Ring Basic properties of rings,units,ideals,homomorphisms,quotient rings,prime and maximal ideals,fields of fractions,Euclidean domains,principal ideal domains and unique factorization domains,polynomial and power series rings,Chinese Remainder Theorem,local rings and localization,Nakayama's lemma,chain conditions and Noetherian rings,Hilbert basis theorem, Artin rings,integral ring extensions,Nullstellensatz,Dedekind domains,algebraic sets,Spec(A). Module Modules and algebra Free and projective;tensor products;irreducible modules and Schur’s lemma;semisimple,simple and primitive rings;density and Wederburn theorems;the structure of finitely generated modules over principal ideal domains,with application to abelian groups and canonical forms;categories and functors;complexes,injective modues,cohomology;Tor and Ext. Field
第一部分：组合数学 第一章计数的基本原则 一．组合数学的历史和内容 1.历史：组合数学最早起源于中世纪的印度，在漫长的历史中，一 直发展缓慢。随着上一世纪计算机的出现，组合数学开始快速地发展。近几年，由于计算机安全领域受到重视以及组合数学在计算机安全领域的应用，组合数学受到越来越多的重视。 2.内容：组合数学主要包括以下几个内容： (1)组合分析（也称为组合计数理论） (2)组合优化（包括线性规划，整数规划等） (3)组合设计（包括区组设计等） (4)组合算法（例如：搜索算法，DFS算法与分支定界法，动态规 划等） *图论本是组合数学这个家族的一个主要成员，但它已成长壮大，独立成一门学科。 3. 本课程介绍的主要内容：组合计数理论 二．加法原则与乘法原则 1. 加法原则： 设事件A有m种产生方式，事件B有n种产生方式，则“事件A 或事件B”有m+n种产生方式。 例子：大于0而小于10的偶数有4个，即：{2，4，6，8}，大于0而小于10的奇数有5个，即：{1，3，5，7，9}。则大于0而小于10
的整数有：4+5=9个，即：{1，2，3，4，5，6，7，8，9}。 *如果A1,A2,?,A n是互不相交的有穷集，那么 |A1∪A2∪?∪A n|=|A1|+|A2|+?+|A n| 2.乘法原则： 若事件A有m种产生方式，事件B有n种产生方式，则“事件A 与事件B”有mn种产生方式。 例1：设一个符号由两个字符组成，第一个字符有a,b,c,d,e五种方式，第二个字符有1,2,3三种方式。则根据乘法原则，该符号具有5×3= 15种方式，即 a1,b1,c1,d1,e1;a2,b2,c2,d2,e2;a3,b3,c3,d3,e3. 例2：从A到B有3条不同的道路，从B到C有2条不同的道路，从A经B到C共有n=3×2=6条不同的道路。 例3：求比10000小的正整数中含有数字1的数的个数。 解：先求所有4位数中不含有数字1的个数，即求由{0，2，3，4，5，6，7，8，9} 9个数字组成的4位数的个数。每一位都有9种出现方式，根据乘法原则，由9个数字组成的4位数个数为：9×9×9×9= 6561，其中包含0000不是正整数。故比10000小不含数字1的4位正整数的个数=6561?1=6560. 所以小于10000含有数字1的4位数个数=9999?6560=3439.
第十九届北京市大学生数学竞赛本科丙组试题及解答 一、填空题（每小题3分，共30分） 1．?? ????+-+-+∞→1)2(lim 6 1 23x e x x x x x = 1/6 . 2．设)(x f 连续，在1=x 处可导，且满足 )0(,)(8)sin 1(3)sin 1(→+=--+x x o x x f x f 则曲线)(x f y =在1=x 处的切线方程为 y =2x －2 . 3．设)(x y y =是由0sin ) ln(2 =- ? +-y y x t dt e y 所确定的函数,则 ==0 y dx dy －1 . 4. 设243),(lim 2 20 0=+-+→→y x y x y x f y x , 则='+')0,0()0,0(2y x f f －2 . 5. 1sin 1cos x x e dx x +=+?tan 2 x x e C + . 6．设函数()u ?可导且(0)1?=，二元函数()xy z x y e ?=+满足 0z z x y ??+=??，则()u ?=24 u e - . 7. = += +≤+??D dxdy y x I y x y x D )32(,:22则设π4 5 . 8. 数项级数 ∑∞ =--1 )! 2()! 2()1(n n n n n n 的和=S －1+cos1+ln2. 9. 123ln 1ln 1ln 1ln 1lim 123n n n n n n n n n n n n n n n →+∞?? ????????++++ ? ? ? ? ? ???????? ? ++++= ?++++ ??? 2ln21- . 10.= ='==+'+''? ∞+0 )(1)0(,0)0(044)(dx x y y y y y y x y 则，，且满足方程函数设4 1 .
趣味数学知识竞赛复习题 一、填空题 1、（苏步青）是国际公认的几何学权威，我国微分几何派的创始人。 2、（华罗庚）是一个传奇式的人物，是一个自学成才的数学家。 3、编有《三角学》，被称为“李蕃三角”且自称为“三书子”的是（李锐夫）。 4、世界上攻克“哥德巴赫猜想”的第一个人是（陈景润）。 5、（姜立夫）是现代数学在中国最早而又最富成效的播种人”，这是《中国大百科全书》和《中国现代数学家传》对他的共同评价。 6. 设有n个实数,满足|xi|<1(I=1,2,3,…,n), |x1|+|x2|+…+|xn|=19+|x1+x2+…+xn| ,则n的最小值20 7. 三角形的一个顶点引出的角平分线,高线及中线恰将这个顶点的角四等分,则这个顶角的度数为___90° ___ 8. 某旅馆有2003个空房间,房间钥匙互不相同,来了2010们旅客,要分发钥匙,使得其中任何2003个人都能住进这2003个房间,而且每人一间(假定每间分出的钥匙数及每人分到的钥匙数都不限),最少得发出_16024______把钥匙. 9. 在凸1900边形内取103个点,以这2003个点为顶点,可将原凸1900边形分割成小三角形的个数为______2104 _____. 10. 若实数x满足x4+36<13x2,则f(x)=x3-3x的最大值为______18_____ 11 ."我买鸡蛋时，付给杂货店老板12美分，"一位厨师说道，"但是由于嫌它们太小，我又叫他无偿添加了2只鸡蛋给我。这样一来，每打(12只)鸡蛋的价钱就比当初的要价降低了1美分。" 厨师买了＿18只鸡蛋? 12.已知f(x)∈[0,1],则y=f(x)+1的取值范围为 ___[7/9,7/8]____ 13. 已知函数ｆ（ｘ）与ｇ（ｘ）的定义域均为非负实数集，对任意的ｘ≥0，规定ｆ（ｘ）＊ｇ（ｘ）＝ｍｉｎ｛ｆ（ｘ），ｇ（ｘ）｝．若ｆ（ｘ）＝3－ｘ，ｇ（ｘ）＝，则ｆ（ｘ）＊ｇ（ｘ）的最大值为____（2√3－1） _____ 14．已知a,b,cd∈N,且满足342(abcd+ab+ad+cd+1)=379(bcd+b+d),设M=a×103+b×102+c×10+d,则M的值为______ 1949 ___.